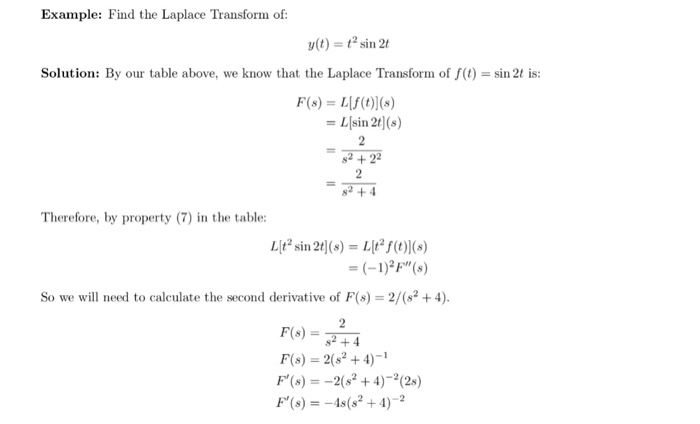
Example: Find the Laplace Transform of: y(t) = 1 sin 2t Solution: By our table above, we know that the Laplace Transform of f(t) = sin 2t is: F(s) = Lif(t)](8) = L[sin 2t] (8) Therefore, by property (7) in the table: = F(s) = 82 +22 2 8 +4 = L[t sin 2t] (s) Lt f(t)](s) = (-1)F" (8) So we will need to calculate the second derivative of F(s) = 2/(s + 4). 2 8 +4 F(s) = 2(8+4)- F'(s) = -2(s+4)-(28) F'(s) = -48 (8+4)- Now we use the product rule. Therefore, F" (s) = -48 [-2(8 + 4)-(28)] + (-4) (s+4)- 4 (82+4) 48 +16 (8+4) = = 168 (82+4)3 168 (8+4)3 12816 (8+4) L[t sin 2t] (s) = L[t f(t)](s) = (-1)F" (s) 12s - 16 (8+4) 2. For each of the following exercises, find the Linear Transform of each of the following functions. Show the steps as shown in the example above. Use Matlab to check each answer and staple your Matlab sheet to the back of your homework. (a) y(t) = t sin 5t (b) y(t) = 1 cos 3t Recall: Recall the property of the Laplace Transform for the first and second derivatives. Ly' (t)] (s) = sLly(t)](s) - y(0) Lly" (t)] (s) = s Ly(t)](s) - sy(0) - y'(0) We can shorten the notation for this if we let Y(s) = Ly(t)](s). Therefore, we will also write this in a more compact way. L(y')(s) sY(s)- y(0)