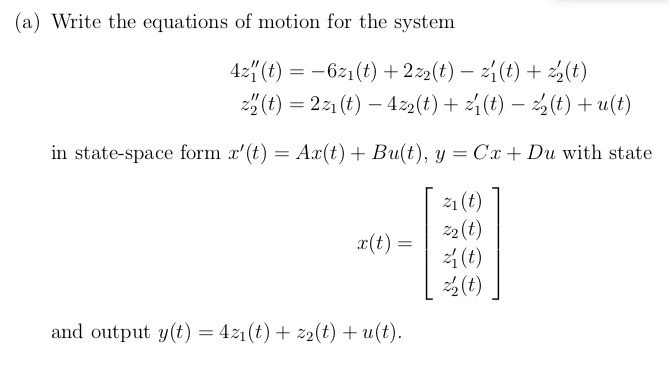
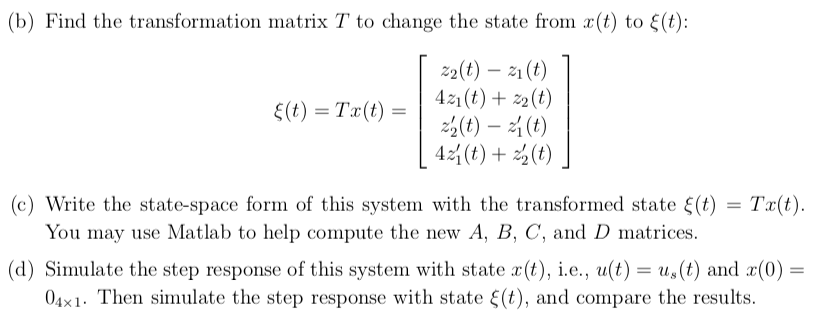
**** Please explain step by step ****

Thank you

(a) Write the equations of motion for the system 4z"(t) = -6z(t) + 2z2(t) z(t) + z(t) z(t) = 2z(t) 4z2(t)+7(t) z(t) +u(t) in state-space form x'(t) = Ax(t) + Bu(t), y = Cx + Du with state .(t) = z2(t) z(t) z(t) and output y(t) = 4z(t) + z2(t) +u(t). (b) Find the transformation matrix T to change the state from r(t) to f(t): f(t) = Tx(t) = z2(t) zi(t) 4zi(t) + z2(t) z(t) (t) 42(t) + z(t) (c) Write the state-space form of this system with the transformed state &(t) Tx(t). You may use Matlab to help compute the new A, B, C, and D matrices. (d) Simulate the step response of this system with state x(t), i.e., u(t) = ug(t) and x(0) = 04x1. Then simulate the step response with state f(t), and compare the results. (a) Write the equations of motion for the system 4z"(t) = -6z(t) + 2z2(t) z(t) + z(t) z(t) = 2z(t) 4z2(t)+7(t) z(t) +u(t) in state-space form x'(t) = Ax(t) + Bu(t), y = Cx + Du with state .(t) = z2(t) z(t) z(t) and output y(t) = 4z(t) + z2(t) +u(t). (b) Find the transformation matrix T to change the state from r(t) to f(t): f(t) = Tx(t) = z2(t) zi(t) 4zi(t) + z2(t) z(t) (t) 42(t) + z(t) (c) Write the state-space form of this system with the transformed state &(t) Tx(t). You may use Matlab to help compute the new A, B, C, and D matrices. (d) Simulate the step response of this system with state x(t), i.e., u(t) = ug(t) and x(0) = 04x1. Then simulate the step response with state f(t), and compare the results