Please finish question 3.1 and 3.2. I will upvote

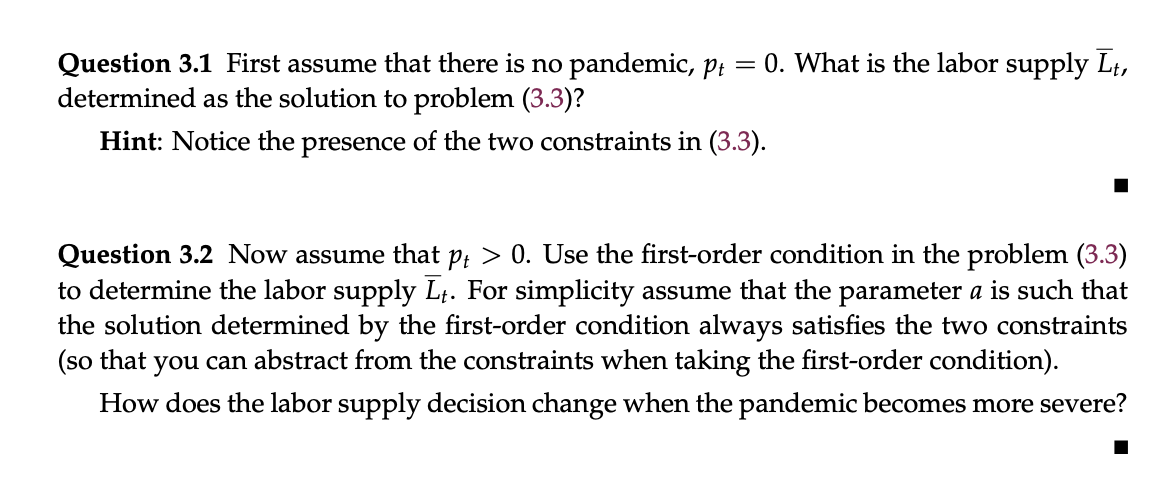
We will assume that actual output is produced using the production function Yt=AtKLt1, where At is unobserved TFP, K is a known level of capital (which we will keep fixed for simplicity), and Lt is the number of workers actually working. On the other hand, potential output is defined as Yt=AtK(Lt)1, where Lt is the number of workers who would be willing to work at the prevailing wage, given the pandemic situation, described next. Workers supply labor but are afraid to work when the pandemic is severe. In particular, their labor supply is determined by the following optimization problem maxLtLtwtpt2aLt2subjecttoLt0andLt1 Here, pt is the current state of the pandemic, which is increasing in its current severity, while a>0 is a parameter. You can think about pt as probability of getting infected when working. The first term in the objective function, Ltwt, represents the wage income, while the second term, pt2aLt2, represents the disutility associated with risks of getting infected while working. The constraint Lt0 states that labor supply cannot be negative, while Lt1 states that total available labor supply (the number of all potentially available workers in the economy) is normalized to one. The solution to problem (3.3) represents the number of workers who are willing to work in the prevailing state of the pandemic, at the prevailing wage wt. This is the quantity of labor Lt used in the definition of potential output in expression (3.2). Question 3.1 First assume that there is no pandemic, pt=0. What is the labor supply Lt, determined as the solution to problem (3.3)? Hint: Notice the presence of the two constraints in (3.3). Question 3.2 Now assume that pt>0. Use the first-order condition in the problem (3.3) to determine the labor supply Lt. For simplicity assume that the parameter a is such that the solution determined by the first-order condition always satisfies the two constraints (so that you can abstract from the constraints when taking the first-order condition). How does the labor supply decision change when the pandemic becomes more severe? We will assume that actual output is produced using the production function Yt=AtKLt1, where At is unobserved TFP, K is a known level of capital (which we will keep fixed for simplicity), and Lt is the number of workers actually working. On the other hand, potential output is defined as Yt=AtK(Lt)1, where Lt is the number of workers who would be willing to work at the prevailing wage, given the pandemic situation, described next. Workers supply labor but are afraid to work when the pandemic is severe. In particular, their labor supply is determined by the following optimization problem maxLtLtwtpt2aLt2subjecttoLt0andLt1 Here, pt is the current state of the pandemic, which is increasing in its current severity, while a>0 is a parameter. You can think about pt as probability of getting infected when working. The first term in the objective function, Ltwt, represents the wage income, while the second term, pt2aLt2, represents the disutility associated with risks of getting infected while working. The constraint Lt0 states that labor supply cannot be negative, while Lt1 states that total available labor supply (the number of all potentially available workers in the economy) is normalized to one. The solution to problem (3.3) represents the number of workers who are willing to work in the prevailing state of the pandemic, at the prevailing wage wt. This is the quantity of labor Lt used in the definition of potential output in expression (3.2). Question 3.1 First assume that there is no pandemic, pt=0. What is the labor supply Lt, determined as the solution to problem (3.3)? Hint: Notice the presence of the two constraints in (3.3). Question 3.2 Now assume that pt>0. Use the first-order condition in the problem (3.3) to determine the labor supply Lt. For simplicity assume that the parameter a is such that the solution determined by the first-order condition always satisfies the two constraints (so that you can abstract from the constraints when taking the first-order condition). How does the labor supply decision change when the pandemic becomes more severe








