


:Please help me answer the following questions correctly.
You are a network professional. Your company's network consists of one file and print server, one database server, and 25 workstations. The company wants to add a web server for customer access and an Internet connection to the network for the workstations.
For this assignment:
Identify four likely security threats to the web server and/or Internet connection
Research and describe appropriate countermeasures to maintain security of the web server and Internet connection.
Form a short report that describes each threat and the countermeasures you selected. Discuss the reasons you chose those countermeasures. Discuss the benefits and limitations of each countermeasure. Do not use the same countermeasure more than once.
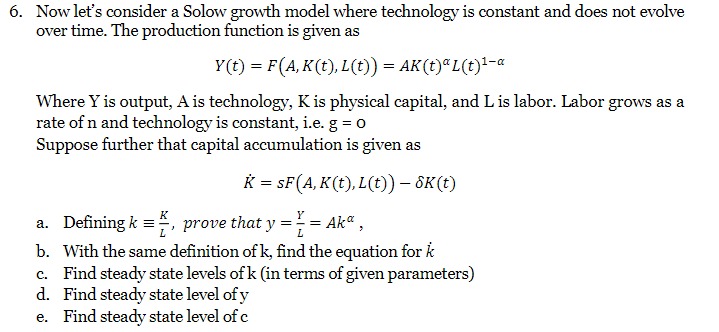
EXERCISE Question 1 (Intertemporal Choice: Basic Example) Suppose there are two people living for two periods, Ann and Bill. Both persons have identical preferences within each period and across time. They have no endowment at the beginning of their lives and will not bequeath at the end of their lives. Let the interest rate be R = 10%. Suppose the lifetime income of Ann is (31, 9/2) = (100,0) and Bill is (31, 92) = (0, 110). Whose consumption is larger in the first period? Question 2 (Substitution and Wealth Effect in Two-period Model, Problem 3.7) What are the effects of the following changes on current and future consumption (c1, c2) and work effort (61, (2)? Explain both substitution and wealth effect. 1. A permanent parallel upward shift in production function in both periods. (Hint: Recall Chapter 2.) 2. An increase in interest rate. 3. A temporary change in the marginal product of labor. Question 3 (Intertemporal Optimization) Suppose household has a utility function a (ci, c2) = log (a) + 1/slog (c2). The income stream is (31, 12) = (50, 60). Assume P = 1, bo = 0, by = 0, and R = 20%% = 0.2. What is the optimal consumption (c1, c2 ) and saving (b1 - bo) plan? Question 4 (Wealth Effects for Infinitely-lived Household, Problem 3. 10) Consider the household's budget constraint in real term over the infinite horizon 02 12 + ... =yi+ 1 + R + ... +- bo (1 + R) Ci # 1+ R P Evaluate the wealth effect of the following scenarios. 1. An increase in price level P, for a household that has a positive value of by. 2. An increase in the interest rate R, for a household that has bo = 0 and at = y in all period t = 1, 2,... 3. An increase in the interest rate R, for a household that has by = 0 with the consumption plan such that c yt for t > T. Question 5 (Permanent Income, Adapted from Midterm 2003) A consumer lives for three years. He can save or borrow to smooth his consumption over his lifetime. Assuming that his goal in life is to smooth his consumption over time. His income in Year 1, 2 and 3 are $27000, $50000, and $25000, respectively. The interest rate between the first and second year is 25%, while between the second and third year is 100%. Assume he receives no endowment and plans to leave no bequest. 1. What is his permanent income? 2. What is his annual consumption? 3. What is the amount that he saves or borrow, if any, in the first year? Question 6 (Money Demand, Adapted from Midterm 2003 and Problem 4.9, 4.10) Consider a worker with an annual income of $12090. Suppose he receives wage payments (of equal value) once a month. Consumption is constant at $12000 per year. Assume that the worker holds no bonds; that is, he holds all financial assets in the form of money. 1. Assume that he shops continuously. (a) What is his average money balance? Draw the graph denoting the money balance over time. (b ) If he receives wage twice a month instead of once a month, what is his average money balance? 2. Revert to the case that he receives his wage once a month. Suppose instead that he shops 5 times each month, also, he immediately shops when he has received his wage. (a) What is his average money balance? Draw the graph denoting the money balance over time. (b ) If he shops 2 times each month instead of 5 times each month, what is his average money balance? (c) If it is more expensive to go shopping, e.g. UW-Madison charges higher bus fare, what will happen to his shopping frequency? What will happen to his average money balance?6. Now let's consider a Solow growth model where technology is constant and does not evolve over time. The production function is given as Y (t) = F(A, K(t), L(t)) = AK(t) "L(t ) 1-a Where Y is output, A is technology, K is physical capital, and L is labor. Labor grows as a rate of n and technology is constant, i.e. g = 0 Suppose further that capital accumulation is given as K = SF(A, K(t), L(t) ) - 6K(t) a. Defining k = * 7. prove that y = = = Ak", b. With the same definition of k, find the equation for k c. Find steady state levels of k (in terms of given parameters) d. Find steady state level of y e. Find steady state level of cQuestion Question 4A Suppose that individual consumer has the following tastes u(r. y) = 50Inx + y. Py = 1, Pr = p is price of good r and income is I. (a) Derive individual, own price demand curve for good a with this utility function and the standard two good budget constraint. Producers of good r operate in competitive markets in which w = $10 and r = $20 and all producers face common technology, captured by the production function z == f((, k) = 40/0.251025. In the short run, production function has form f(6, &) = A(025 and capital is fixed at K. In addition to the input costs of labor and capital, this firm has to pay a recurring fixed cost which is license fee, equal to $1000 per period. This fee has to be paid, even if firm shuts down production in short run and can only be driven to zero in the long run when firm exits the industry. (b) Derive the cost function and then the marginal and average cost functions for this technology for a given firm in short run. What is the long run total cost function, long run average cost and long run marginal cost function for a given firm. (e) Derive the short run and long run output supply function for a given firm. You can assume that capital is fixed at & in short run and variable in long run. (d) Assume that number of firms in industry in short run equilibrium is A and number of consumers are M. Which condition defines the industry equilibrium in short run? Set up this condition to derive short run market equilibrium price and quantity. The equilibrium will be a function of unknown parameters such as N, M and E. (e) What is the minimum level of long run average cost and minimum level of short run average cost if k = 200 for an individual firm? (f) If N = 10000, MY = 15000 and & = 200, what is the output supplied by every individual firm in short run equilibrium and what are the numbers for short run equilibrium price level and equilibrium quantity at industry level? (g) Is every individual firm making an economic profit or loss or neither at this equilibrium price with respect to short run average costs? What about the profit or loss with respect to long run average costs for each firm? In light of these answers, do you expect entry or exit in the industry in long run at this current short run equilibrium price? Explain briefly.1. Consider the following moral hazard game, with a risk-neutral principal hiring a risk neutral agent. In other words, the principal's payoff is given by 1!: = q w and the agent's payoff is given by U = w e. The agent's reservation utility is given by fl = 5, and the agent can choose between an effort level of 0 or an effort level of 10. There is a bankruptcy constraint, such that w 2 0. Output follows the following probability distribution, a function of effort level: Probability (q=0) Probability (q=400] e=0 \"-5;- e=10 'E- a} What would be the agent's effort choice and utility if he owned the rm, i.e. the agent gets all of the output? bl What is the participation constraint for the principal (PC)? What about the incentive compatibility constraint (ICC)? Write out the expression for the principal's expected profits as well. c) If agents are scarce and principals compete for them, find an optimal contract under full information? What will the agent's utility be? What about the principal's profits? d) If principals are scarce and agents compete to work for them, find an optimal contract under full information? What will the agent's utility be? What about the principal's profits? e} If principals are scarce and agents compete for work, what will the contract be when the principal cannot observe effort? What will the payoffs be for each player














