Please help me i will give good rating

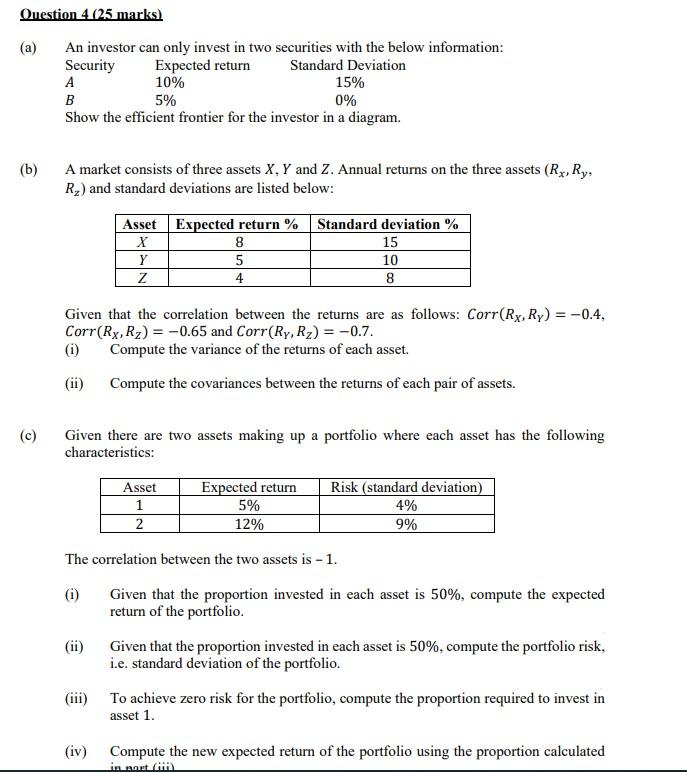
Question 4 (25 marks) (a) An investor can only invest in two securities with the below information: Security Expected return Standard Deviation A 10% 15% B 5% 0% Show the efficient frontier for the investor in a diagram. (b) A market consists of three assets X, Y and Z. Annual returns on the three assets (Rx, Ry, R) and standard deviations are listed below: Asset Expected return % Standard deviation % X 8 15 Y 5 10 Z 4 8 Given that the correlation between the returns are as follows: Corr (Ry, Ry) = -0.4, Corr (Rx, Rz) = -0.65 and Corr (Ry, Rz) = -0.7. (i) Compute the variance of the returns of each asset. (ii) Compute the covariances between the returns of each pair of assets. (c) Given there are two assets making up a portfolio where each asset has the following characteristics: Asset Expected return 1 5% Risk (standard deviation) 4% 9% 2 12% The correlation between the two assets is - 1. (i) Given that the proportion invested in each asset is 50%, compute the expected return of the portfolio. Given that the proportion invested in each asset is 50%, compute the portfolio risk, i.e. standard deviation of the portfolio. (iii) To achieve zero risk for the portfolio, compute the proportion required to invest in asset 1. (iv) Compute the new expected return of the portfolio using the proportion calculated in nort (iii) Question 4 (25 marks) (a) An investor can only invest in two securities with the below information: Security Expected return Standard Deviation A 10% 15% B 5% 0% Show the efficient frontier for the investor in a diagram. (b) A market consists of three assets X, Y and Z. Annual returns on the three assets (Rx, Ry, R) and standard deviations are listed below: Asset Expected return % Standard deviation % X 8 15 Y 5 10 Z 4 8 Given that the correlation between the returns are as follows: Corr (Ry, Ry) = -0.4, Corr (Rx, Rz) = -0.65 and Corr (Ry, Rz) = -0.7. (i) Compute the variance of the returns of each asset. (ii) Compute the covariances between the returns of each pair of assets. (c) Given there are two assets making up a portfolio where each asset has the following characteristics: Asset Expected return 1 5% Risk (standard deviation) 4% 9% 2 12% The correlation between the two assets is - 1. (i) Given that the proportion invested in each asset is 50%, compute the expected return of the portfolio. Given that the proportion invested in each asset is 50%, compute the portfolio risk, i.e. standard deviation of the portfolio. (iii) To achieve zero risk for the portfolio, compute the proportion required to invest in asset 1. (iv) Compute the new expected return of the portfolio using the proportion calculated in nort (iii)