Please help me out with these mathematical problems! Thanks a lot!

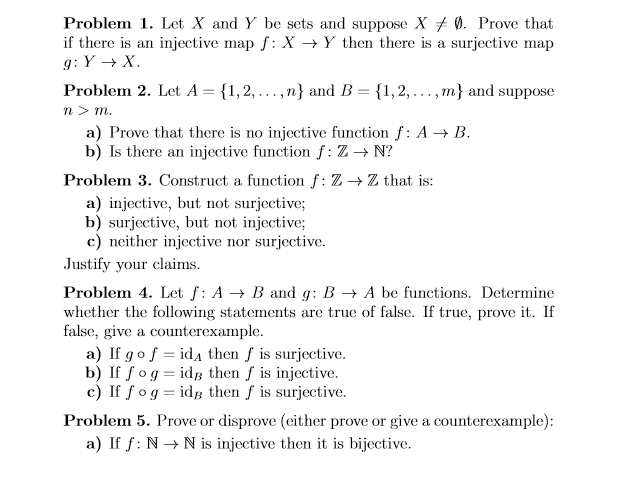
b] \"151 I- f. mllm ff !1 than I ll: hiijh-n- 11] If f: I i- I HHl-il flu-Jr id:I Lhtm f in hijnnliwt. Pmblcm IE. LuL a, b C E and mnsidnr Lhn I'Imnt'mn LEHII-E; I[:,y}=m:+by. a] What is the rm1gc [lmagn] HI I? h] I'Imrtr l.hJ-1L f i.H HIIrjnt:t.iw1 if and \""11" if Elwlfmlll _ ]_ c} Pmm that I Ll; nomr injctivc. Pulhlnm T. Lul f: H :u: H 1r H IZH! Lhn lnntiml Ikrrlm I13: I [mm] = 2'\" 3\". H] I'Invtr that I i.H injlj'L b} In. J" Hurjumtiwf.' Pruh-Iurn E. Lul I: I :r I. III! (inlll'l 1:3: n+4 il'n.=E| [mu-d 3}. n] = 1r: :1 if n. :1 [mad ii}, n+1 if\". E 2 [Inn-ti 3}. a} 15'. _.|" 'mjmtim'? h] In I mlrjlmljw-f? {L} 1-3. _.|" hijmtim'? If m, {lmplll its inrm-31:. Pruh-Iurn 9. LEI. JI'-_ A i* H I'Ht H. funnl'unn. Shim Lhr. JI' iH injtx:tiw1 if and Dl'lljl' 'II' it. Hti'if the fullnwing mnditinn: Ftnr [hwy ml.- X and funaLinnsg: X ]r.r"l and 31:37 1- .41, fug Inhimplim thug h._ Give an rmmplc [an aw that if I is um, injwmi'm, Lhn mnitiun fails. Problem 11]. Lu'r. % {lllljuqm 1} and 3:. = {kw-:3," :gdikaml = 1}~ LEI. a C 3;, and dcm: M\": In]. J- Em; Mnfk} = [aklm whrrm |ak|m dumb}: Lhn unique damn-m in I.\" mngnmm tr.- aJ: [mud m}- a} Pram that M, 15'. hijmlim. h] I'Invtr I: E 35"\" if lll'l {ml}r if M, {In} E IL. {L} Pram that Ll'm mshl'ichinn {if M, tn Z; is a hijaclinn uni-u 3;... Pruh-Iurn 11. Lnl. :1 and H 1m Halal HIH'I ll'.l.- FU'IL PU?) thunk: 1.hn rmpmtiw: mum .'it'rl-H. a} Dulinn an injnctiw map (,9: A :r Fm} b] Suppnsrr 1': J1 :- B is hijarrLivc- Dene a hij'rntiun F: 11m} } FIB}. Problem 1. Let X and Y he sets and suppose X 7} . Prove that if there is an injective map I : X > 1" then there is a surjcctivc map 5-: F i X. Problem 2. Let A = {1,2, .. .,'irr} and B = {1,2, . .. ,m} and suppose ppm. 3.) Prove that there is no injective function f : A } B. b) Is there an injectivc function f : E > N? Problem 3. Construct a function f : E > E that is: a} hijectiw, but not siirjective; b} surjeetive, but not injective', e} neither injective nor surjective. Justify 3mm claims. Problem 4. Let f : A } B and g: B } A be functions. Determine whether the illim'ing statements are true of false. if true, prove it. If false, give a enunterexmnple. a) If g o f = 1d,, then f is surjectivc. b) If f o g = 1d,; then f is hijcctive. c) If f o g = 111;; then f is surjcctivc. Problem 5. Prove or disprove (either prove or give a counterexample}: a) If f : N > N is injective then it is hijective