Please help me to understand

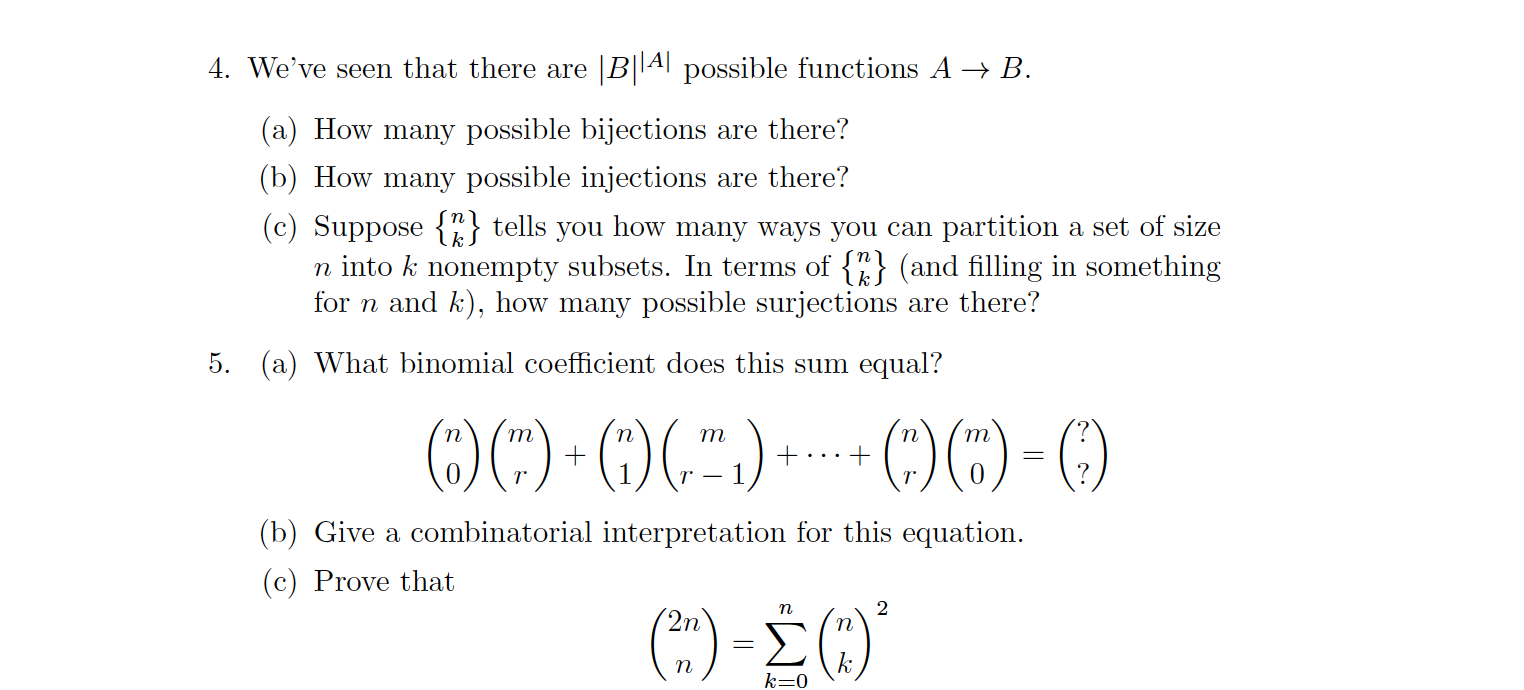
2. Let K6 denote the complete graph on 6 vertices (that is, there is an edge between every pair of vertices). A triangle is a set of three vertices that are all connected to each other. A set of two edges that share a vertex is called an incident pair (i.p.). The shared vertex is called the center of the i.p. For example7 {(uv), (vw)} is an i.p. Where 115,1), and w are distinct vertices and v is the center. (a) How many triangles are there in K5? (b) How many incident pairs are there? Now suppose every edge is colored red or blue. A triangle or i.p. is called multicolored when its edges are not all the same color. (c) Consider the mapping from incident pairs to triangles we get by adding the \"third\" edge: {Cu/iv): (viwll '-> HEW): (WU): (114%)} Note that multicolored i.p.s map to multicolored triangles. Show that this mapping is 2to1 on multicolored objects. ((1) Show that at most 6 multicolored i.p.s can have the same center. (e) Show that there are at most 36 possible multicolored i.p.s. (f) If every pair of people in a group are friends, or if every pair are strangers7 the group is called uniform. Show the above results imply that every set of 6 people includes two uniform threeperson groups. 3. Given a 5card hard from a 52card deck: (a) A sequence is a hand consisting of ve consecutive cards of any suit (e.g., 5Q? 6Q? 7. 8 91.). An ace may be either high (as in 10-JQK-A, or low, as in A23-45, but can't \"wrap around\" (QK- A23 is not a valid sequence). How many different sequence hands are possible? (b) How many hands consist of cards that are all of the same suit? (0) A straight flush has both of these properties , a sequence all of the same suit. How many different straight ushes are possible? ((1) A straight is a hand that has the sequence property but not all cards are of the same suit. How many different straights are possible? 4. We've seen that there are [ B| A possible functions A -> B. (a) How many possible bijections are there? (b) How many possible injections are there? (c) Suppose {} tells you how many ways you can partition a set of size n into k nonempty subsets. In terms of { } (and filling in something for n and k), how many possible surjective are there? 5. (a) What binomial coefficient does this sum equal? (" ) ( ) + ( " ) ( , " , ) + . + (" ) (") - ( " ) (b) Give a combinatorial interpretation for this equation. (c) Prove that 2 ) = n 2