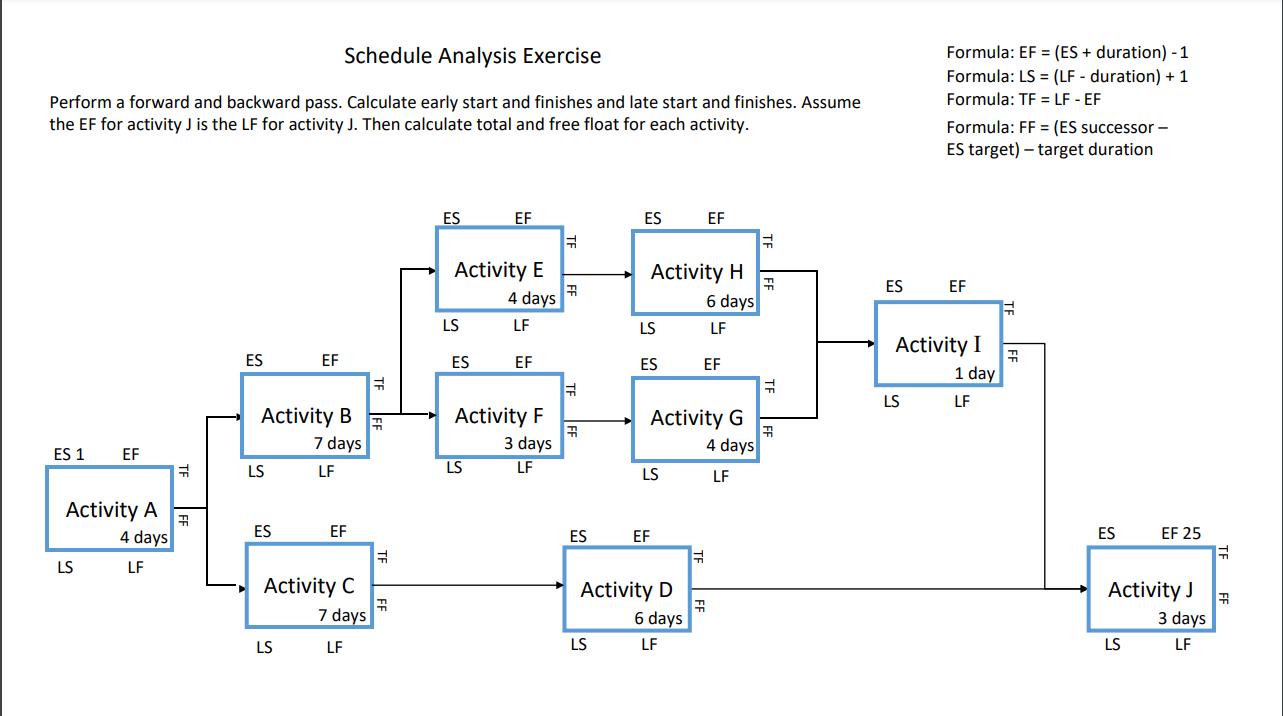
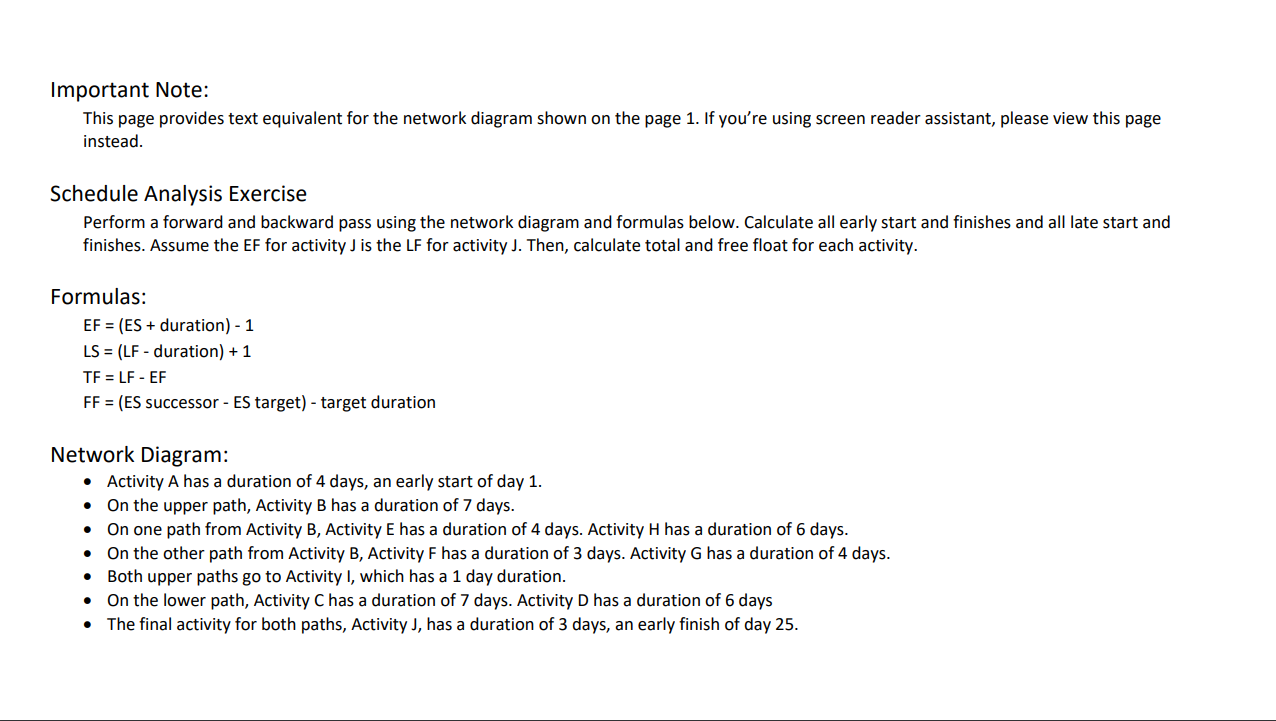
PLEASE HELP ME WITH THESE QUESTIONS!!

What is the critical path duration for this project?

a: 25

b: 20

c: 22

d: 31

How many activities have free float?

a: 0

b: 1

c: 2

d: 3

Which activity has exactly three days of total float?

a: Activity F

b: Activity B

c: Activity D

d: Activity I

Which path has the most total float?

a: A-C-D-J

b: A-B-E-H-I-J

c: A-b-F-G-I-J

What is the predecessor for Activity E?

a: Activity B

b: Activity A

c: Activity F

d: Activity H

How many path convergences are there in this schedule?

a: 3

b: 2

c: 0

d: 1

How many paths are in this schedule?

a: 3

b: 1

c: 4

d: 2

Which activity has an early finish of day 15?

a: Activity F

b: Activity H

c: Activity G

d: Activity E

Activity E and Activity F are on the same path.

a: True

b: False

Which path has a duration of exactly 22 days?

a: A-C-D-J

b: A-B-F-G-I-J

c: A-B-E-H-I-J

Schedule Analysis Exercise Formula: EF=(ES+ duration )1 Formula: LS=(LF duration )+1 Perform a forward and backward pass. Calculate early start and finishes and late start and finishes. Assume the EF for activity J is the LF for activity J. Then calculate total and free float for each activity. Formula: FF= (ES successor Important Note: This page provides text equivalent for the network diagram shown on the page 1. If you're using screen reader assistant, please view this page instead. Schedule Analysis Exercise Perform a forward and backward pass using the network diagram and formulas below. Calculate all early start and finishes and all late start and finishes. Assume the EF for activity J is the LF for activity J. Then, calculate total and free float for each activity. Formulas: EF=(ES+duration)1LS=(LFduration)+1TF=LFEFFF=(ESsuccessorEStarget)targetduration Network Diagram: - Activity A has a duration of 4 days, an early start of day 1. - On the upper path, Activity B has a duration of 7 days. - On one path from Activity B, Activity E has a duration of 4 days. Activity H has a duration of 6 days. - On the other path from Activity B, Activity F has a duration of 3 days. Activity G has a duration of 4 days. - Both upper paths go to Activity I, which has a 1 day duration. - On the lower path, Activity C has a duration of 7 days. Activity D has a duration of 6 days - The final activity for both paths, Activity J, has a duration of 3 days, an early finish of day 25