Please help me with this calc assignment. I'm completely stuck.

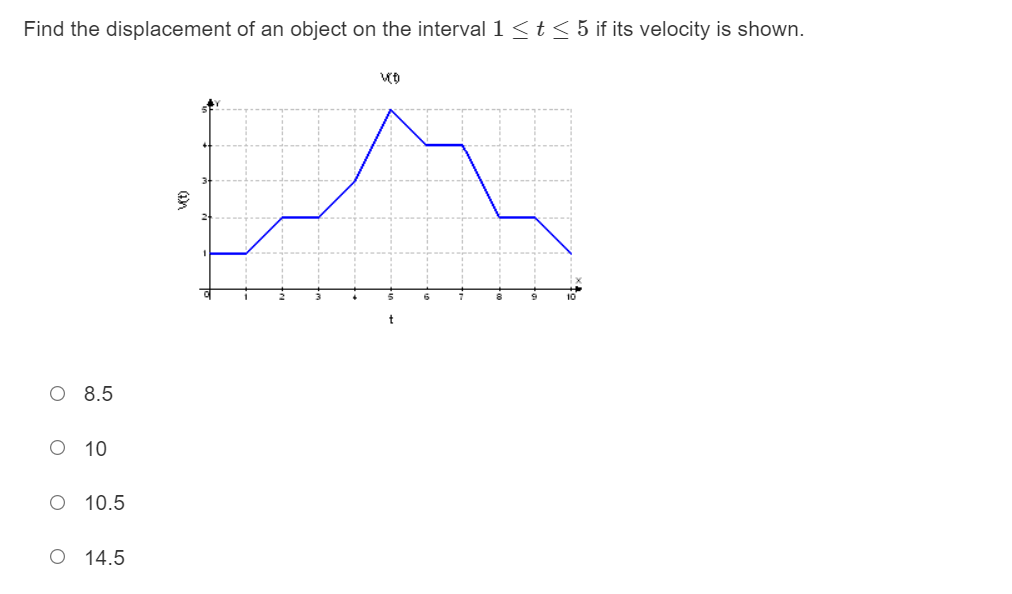
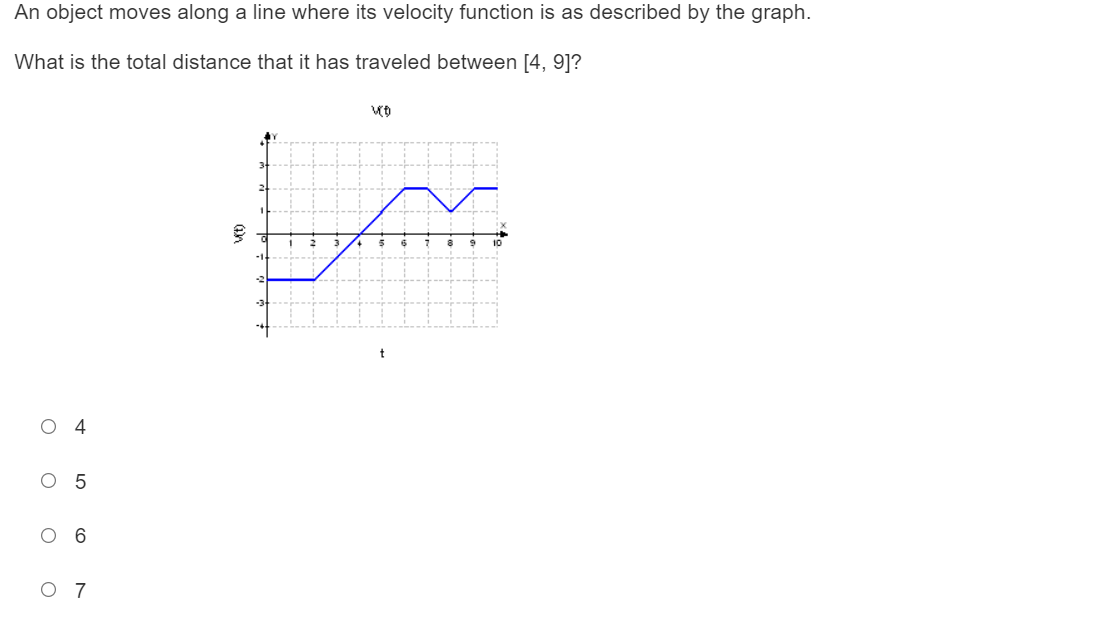
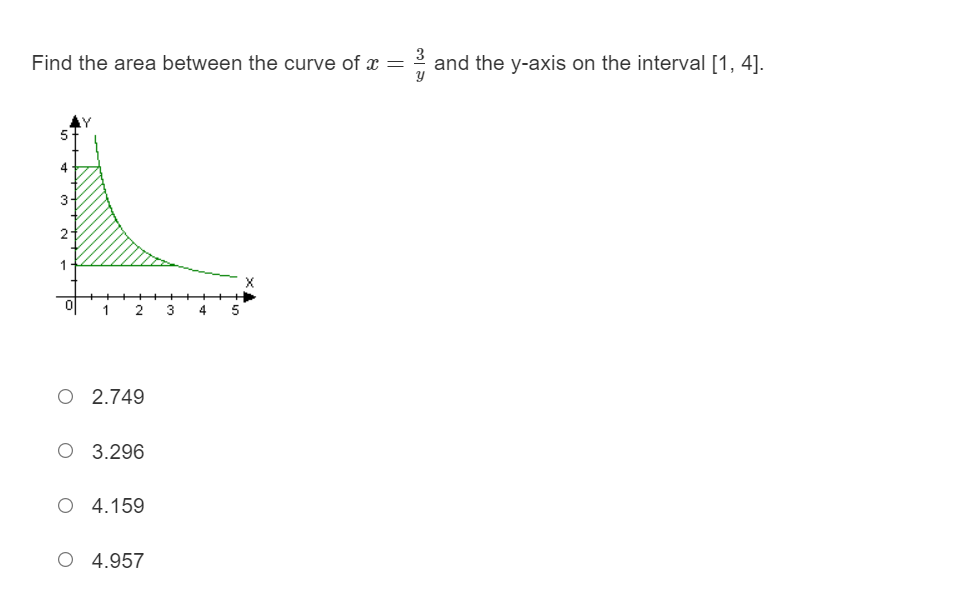
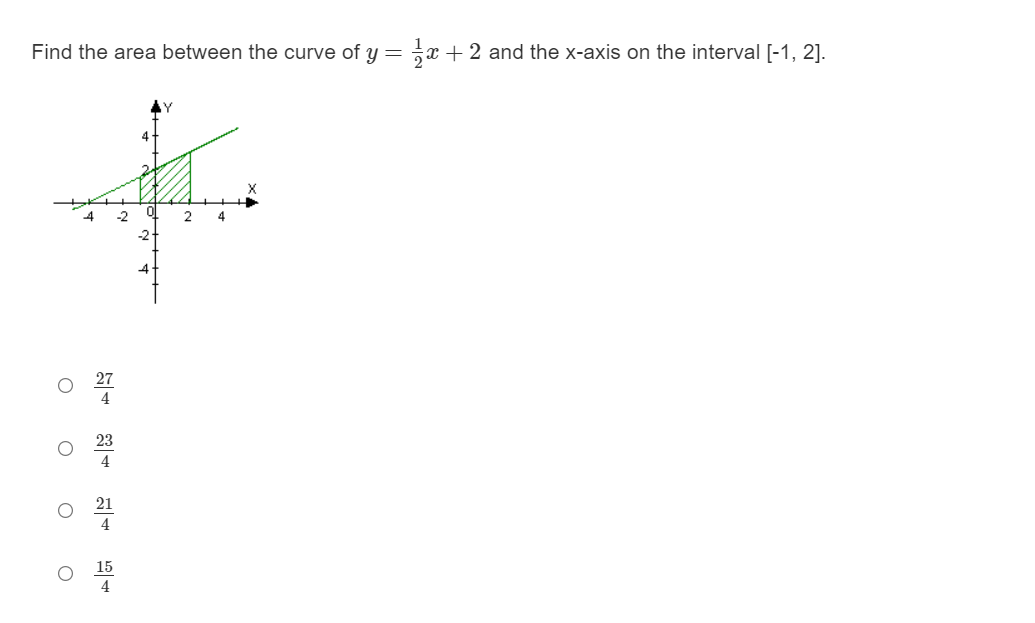
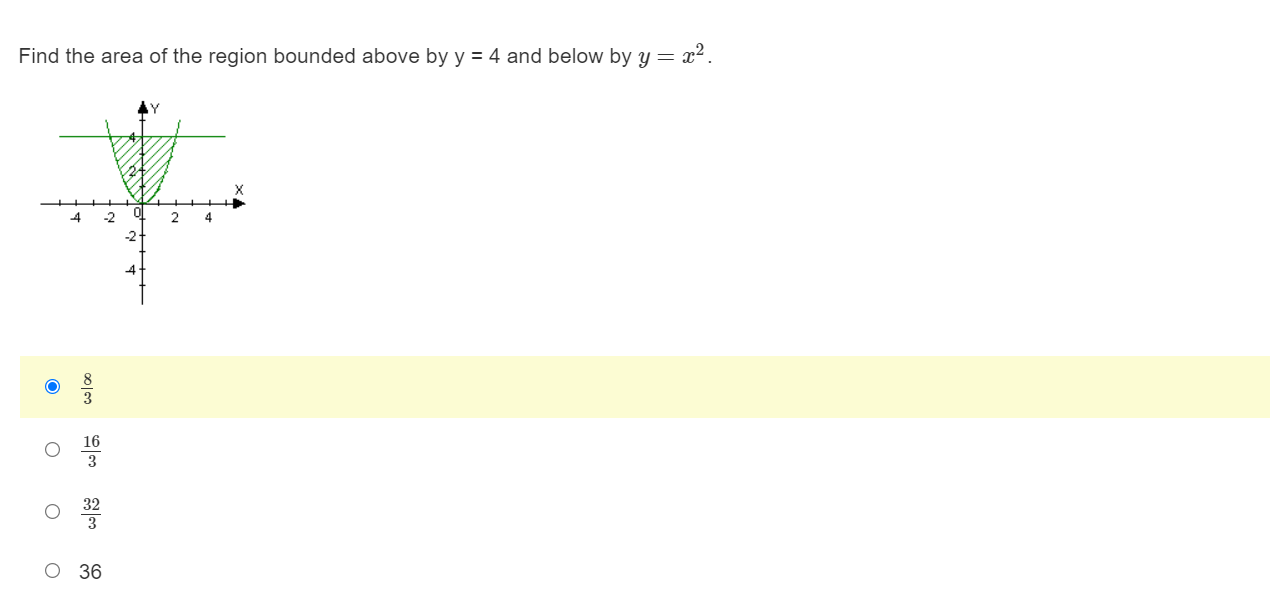
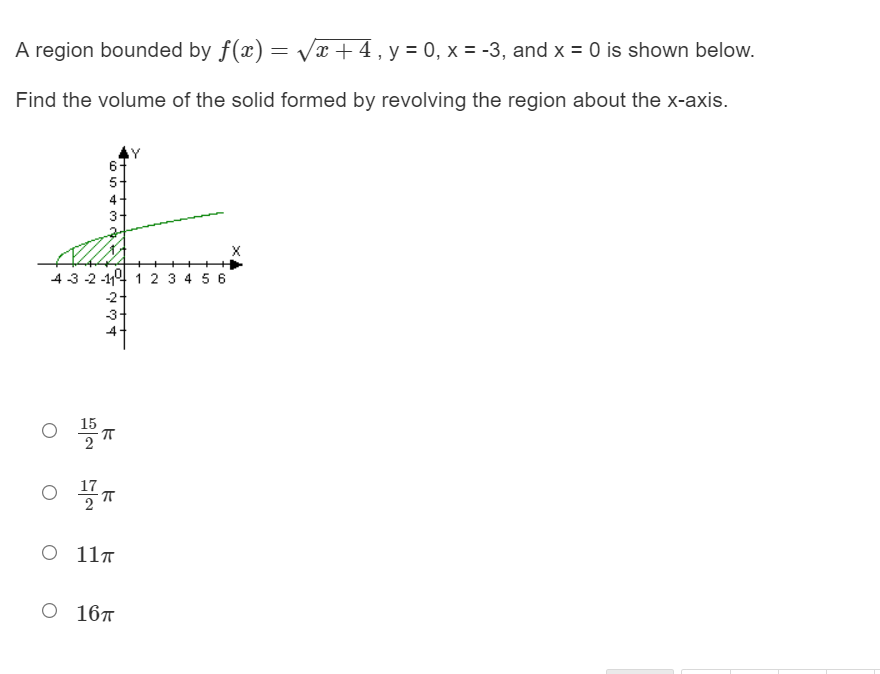
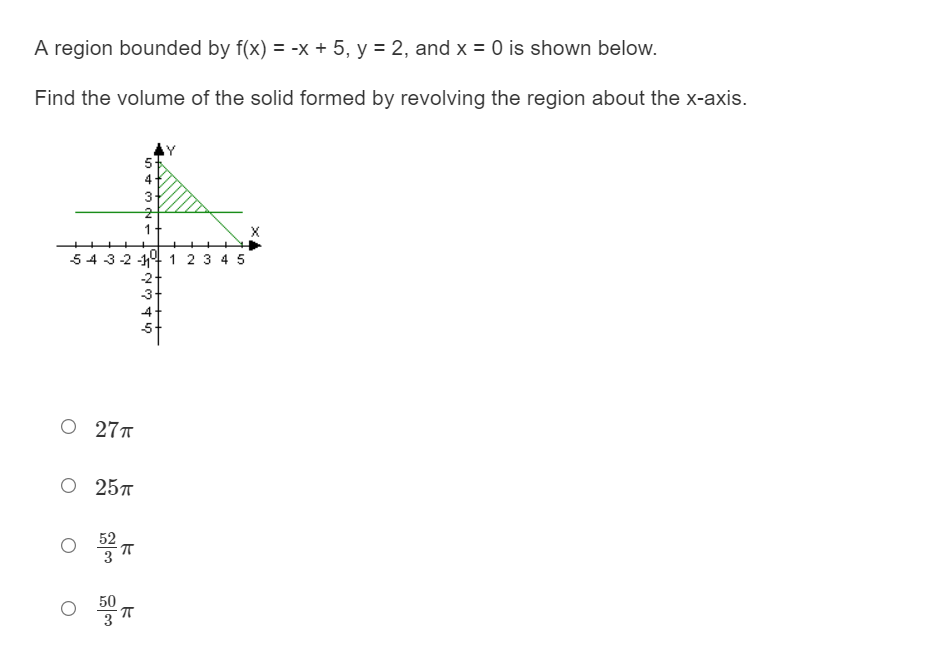
1 An object moves along a path with a velocity t) : t2 gt, and the initial position of the object is x(0) = 3. What is the equation for the position of the object when the acceleration is zero? 0 310\" () du O 3f1(lu2gu) d'u. An object is moving with v(t) = 4 and the position X(1) = 4. What is the position of the object at t = 5? O 731.774 O 735.774 O 738.66 O 739.774Find the displacement of an object on the interval 1 g t g 5 if its velocity is shown. \"It! O 10.5 0 14.5 The position of an object is given by s(t) = In(t3). What is the displacement of the object between t = 1 and t = 4? 0 3 O 4.159 O 4.328 O 16.87An object is moving along a horizontal line such that it's velocity is v(t) = (3t+4) What is the object's displacement between t = 0 and t = 5? O 1.558 O 1.944 O 2.558 O 2.944An object moves along a line where its velocity function is as described by the graph. What is the total distance that it has traveled between [4, 9]? Assume v(t) = 3t - 6 on the interval [0, 4]. What is the total distance that the object traveled on [0, 4]? O 12 O 10 0 8 0 6Find the formula for the total distance traveled by an object with a velocity v(t) = to - 1 on the interval [0, 4]. o - So' (+3 - 1) at + Si (+3 - 1) dt o ful (+3 - 1) dt - S (+3 - 1) dt o fol (+3 - 1) at + S (+3 - 1) dt O - fo' (+3 - 1) dt - S (+3 - 1) dt\fFind the area between the curve of :1: : g and the y-axis on the interval [1, 4]. O 2.749 O 3.296 O 4.159 O 4.957 Find the area between the curve of y : %:B + 2 and the x-axis on the interval [-1, 2]. 27 O? 23 O? 21 0? 0E Find the area of the region bounded above by y = 4 and below by y = $2. \fFind the area of the region bounded to the right by x = y + 3 and to the left by x = y2 + 2y + 1. 2 X 4 6 8 O 4.5 0 4 O 3.75 O 60 / 00The base of a three-dimensional figure is bound by the line y = 4 - x on the interval [-2, 2]. Vertical cross-sections that are perpendicular to the x-axis are rectangles with height equal to 5. Algebraically, find the area of each rectangle. X 32 -1 1 23 4 5 67 O A(x) = 5 (4 +x) O A(x) = 5 (4 -2) O A(a) = (4 -2) O A(x) = 5(4-2)2The base of a three-dimensional figure is bound by the semi-circle y = v4 - x2. Vertical cross-sections that are perpendicular x-axis are squares. Algebraically, find the area of each square. W . X 5432 -1 1 2345 O A(x) = 4- 22 O A(x) = 2(4 + 2) O A(x) = (4 - 2) O A(x) = 2 (4 - a)The base of a three-dimensional figure is bound by the graph x = y3 and the y-axis on the interval [1, 2]. Vertical cross-sections tha are perpendicular to the y-axis are rectangles with height equal to 2. Algebraically, find the area of each rectangle. NW . X 12 3 4 5 6 7 8 9 O y3 O O 3 O 2y3The base of a three-dimensional figure is bound by the line y = 2x + 4 on the interval [-2, 1]. Vertical cross-sections that are perpendicular to the x-axis are squares. Find the volume of the figure. X 3/2 -10 1 23 4 5 6 7 O O 32 3 O 44 3 O 36The base of a three-dimensional figure is bound by the line y = -x2 + 4 on the interval [-1, 1]. Vertical cross-sections that are perpendicular to the x-axis are right triangles with height equal to 6. Find the volume of the figure. HUWAGON X -32 -1 1 23 45 6 7 O O 32 O 44 3 O 22The base of a three-dimensional figure is bound by the line x = y and the y-axis on the interval [0, 2]. Vertical cross-sections that are perpendicular to the y-axis are rectangles with height equal to 3. Find the volume of the figure. -NW X 5432-14 2345 2+ O 13 4 O 15 4 O 17 4 0 8A region bounded by f[x) = x, y = 0, x = 1, and x = 4 is shown below. Find the volume of the solid formed by revolving the region about the x-axis. Auto-hm -2 123456?3 -2 3 .4 5 A region bounded by f(:t?) = 1/3: + 4 , y = 0, x = -3, and x = Dis shown below. Find the volume of the solid formed by revolving the region about the x-axis. 00me 443-2311123455 .4 15 O 1;11' O 11% A region bounded by f(x) = -x + 5, y = 2, and x = 0 is shown below. Find the volume of the solid formed by revolving the region about the x-axis