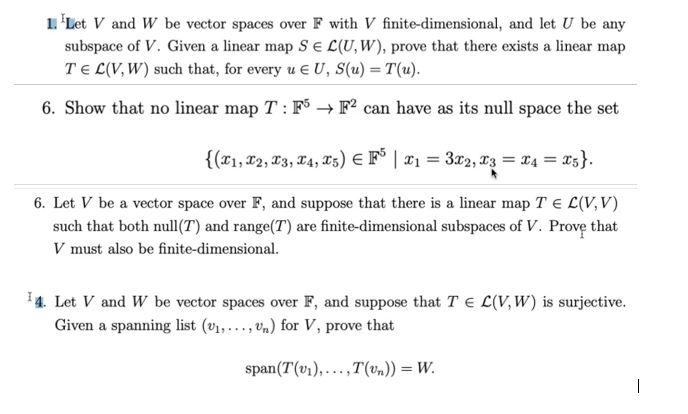
1 Let V and W be vector spaces over F with V finite-dimensional, and let U be any subspace of V. Given a linear map SE L(U,W), prove that there exists a linear map TE L(V, W) such that, for every u e U, S(u) = T(u). 6. Show that no linear map T: F F can have as its null space the set {(*1, 2, T3, T4, T5) E F | 1 = 3x2, x3 = 04 = 05}. 6. Let V be a vector space over F, and suppose that there is a linear map TE L(V, V) such that both null(T) and range(T) are finite-dimensional subspaces of V. Prove that V must also be finite-dimensional. 14. Let V and W be vector spaces over F, and suppose that TE L(V, W) is surjective. Given a spanning list (v),..., en) for V, prove that span(T(v1),...,T(vn)) = W.