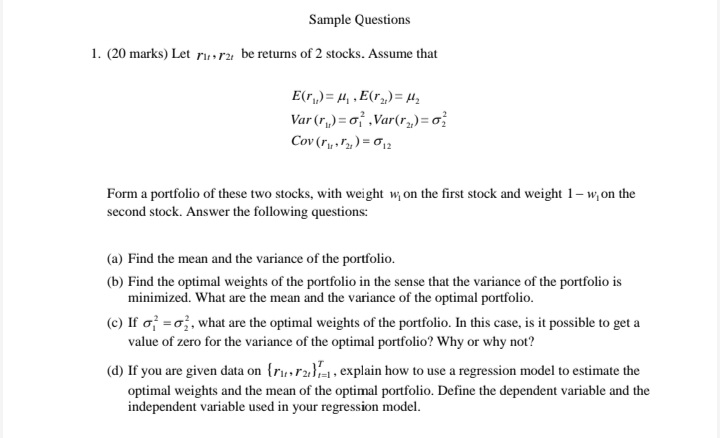
please insert formula for the first 3 questions thanks

Question 3 (25% = 10 points) The concept of likeness is not defined in the GATT but jurisprudence established a four-tier test; discuss the relevance of the four criteria in the Banana case and in the Asbestos case. In the Japan - Alcoholic Beverages II case the Panel added a fifth criterion, namely the "market place"; what are the relevant elements of this criterion? Question 4 (25% = 10 points) According to new Canadian regulations, renewable energy producers domiciled in Canada are permitted to enter into long-term fixed-price electricity purchase contracts at premium rates in exchange for feeding this electricity into the grid if specific domestic content requirements are fulfilled. Do minimum domestic content requirements (LCR) comply with WTO law? If the answer to the above question would be "no", could Canada justify the regulations by referring to the need of strengthening renewable energy in the interest of combatting climate change?Question 1 (25% = 10 points) In a recent book, Nobel prize winner Joseph Stiglitz writes: "Today we have a system of global governance without global government - an array of institutions and agreements (global, regional, bilateral) affecting every aspect of life." In addition he complains about the inadequacy of enforcement mechanisms. How would you assess this statement with regard to international economic law? Which are the key institutions and what is their role? Question 2 (25% = 10 points) Several countries have introduced so called plain packaging laws for cigarettes. Do you see any legal issues that may arise with such laws? How would you advise a tobacco company that wants to take legal steps against a plain packaging law? What are the options?3. (20 marks) Consider the following model: RV, = Bo + B, D., + B, Du + B, Da + B. D., + E, (1) where RV= Realized volatility (annualized) of a stock on day t 1 if t is a Monday 0 otherwise DE 1 if t is a Tuesday 0 otherwise Dy = lift is a Wednesday 0 otherwise D. = 1 if t is a Thursday 0 otherwise After obtaining 40 observations on daily RV, we obtain the following OLS estimation results (with the standard errors in parenthesis). RV, = 0.20 + 0.08D,, -0.01D2, -0.02D -0.03D. + e, (2) (0.01) (0.02) (0.02) (0.02) (0.02) R' =0.25,n = 40 (a) Interpret all the coefficients in model (1), including the intercept. (b) Test the null hypothesis that , = 0 at the 5% level. Explain in detail each step in your test. (c) Test the null hypothesis that B, = B, = B; = B, =0 at the 5% level. Explain in detail each step in your test. (d) Propose a test statistic to test the null hypothesis that B, = B, = B, =0.2. (20 marks) Let ry, be the yield to maturity of 1-month US Treasury bill and ry, be the yield to maturity of 1-year US Treasury bill. Suppose the monthly data between January 1995 and October 2015 (250 monthly observations) on both variable are available. First run two regressions and get the following Eviews output 0.090 + 0.983 /1-1 +e, (0.046) (0.008) 121 = 0.087 + 0.985 /21-1 +e, (0.046) (0.007 ) Answer the following questions: (a) Test the null hypothesis that the slope parameter is zero against it is different from zero at the 5% level in each of the equations. (b) Test the null hypothesis that the slope parameter is one against it is less than one at the 5% level in each of the equations. c) Propose a method to test if r, and ry are cointegrated and explain how to implement the test. (d) Suppose that r,, and r,, are cointegrated. The following estimation results are obtained: Ary, = -0.13(1-, + 0.23 -0.9672,-1) + i, Arz, = 0.16(1-, + 0.23 -0.9672-1) + 1 2 Interpret all three estimates of the slope coefficients (i.e. -0.13, 0.16 and -0.96). (e) Find the VAR model corresponding to the estimated model in (d).Sample Questions 1. (20 marks) Let missry be returns of 2 stocks. Assume that E(r ) = H, , E(r,,) = #2 Var (r )= 01 . Var(r2 )= 0; Cov (ry,2) = 012 Form a portfolio of these two stocks, with weight w on the first stock and weight 1 - w on the second stock. Answer the following questions: (a) Find the mean and the variance of the portfolio. (b) Find the optimal weights of the portfolio in the sense that the variance of the portfolio is minimized. What are the mean and the variance of the optimal portfolio. (c) If of =o, , what are the optimal weights of the portfolio. In this case, is it possible to get a value of zero for the variance of the optimal portfolio? Why or why not? (d) If you are given data on {rus /27=1 , explain how to use a regression model to estimate the optimal weights and the mean of the optimal portfolio. Define the dependent variable and the independent variable used in your regression model