Please organize the work

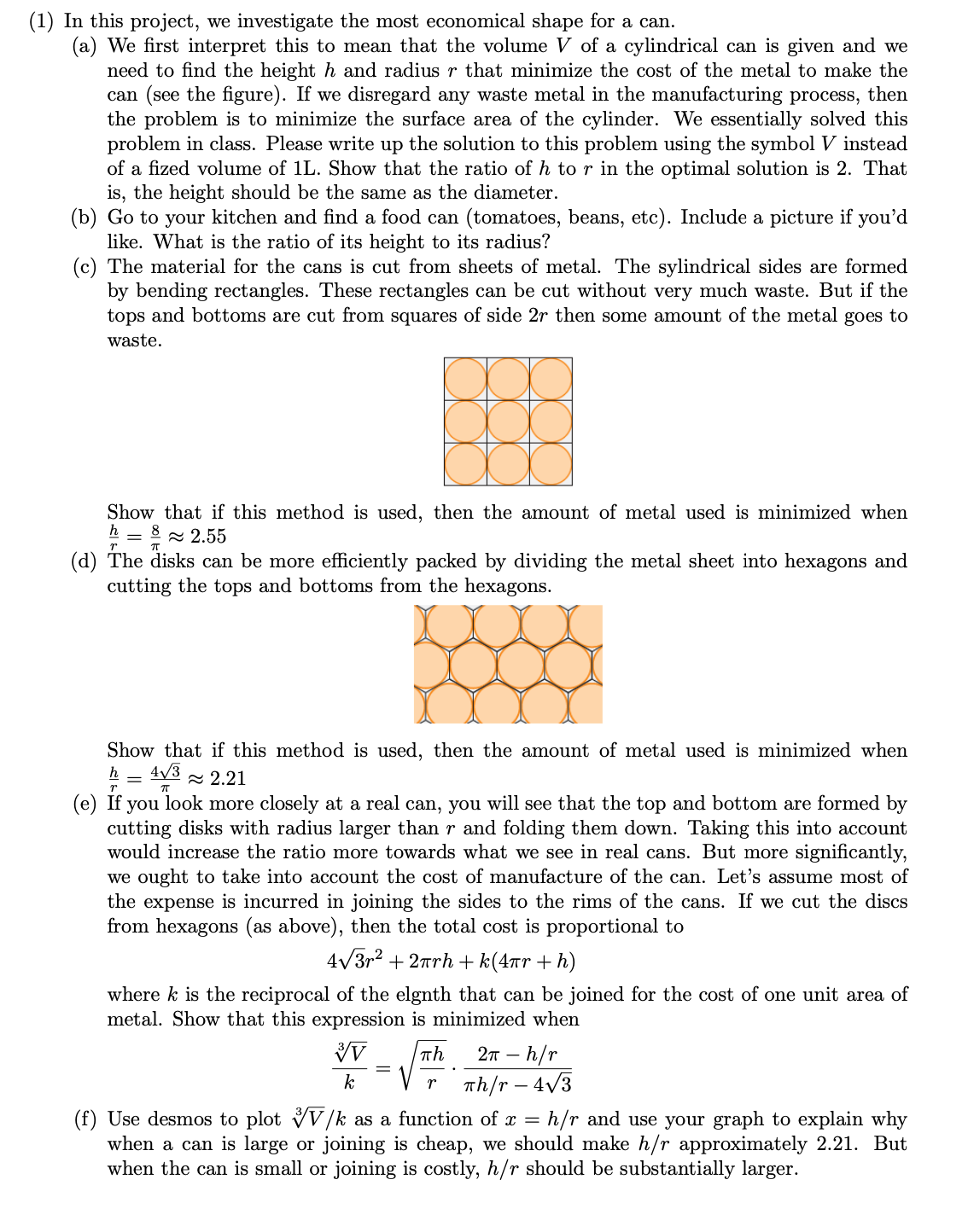
(1) In this project, we investigate the most economical shape for a can. (a) We rst interpret this to mean that the volume V of a cylindrical can is given and we need to nd the height h and radius 1' that minimize the cost of the metal to make the can (see the gure). If we disregard any waste metal in the manufacturing process, then the problem is to minimize the surface area of the cylinder. We essentially solved this problem in class. Please write up the solution to this problem using the symbol V instead of a zed volume of IL. Show that the ratio of h to r in the optimal solution is 2. That is, the height should be the same as the diameter. (b) Go to your kitchen and nd a food can (tomatoes, beans, etc). Include a picture if you'd like. What is the ratio of its height to its radius? (c) The material for the cans is cut from sheets of metal. The sylindrical sides are formed by bending rectangles. These rectangles can be cut without very much waste. But if the tops and bottoms are cut from squares of side 21" then some amount of the metal goes to waste. {I}. r1r 1V1 L 1L 1L1 r.1r 1r 1 L AL AL A Show that if this method is used, then the amount of metal used is minimized when hI=8~2.55 (d) The disks can be more eiciently packed by dividing the metal sheet into hexagons and cutting the tops and bottoms from the hexagons. Show that if this method is used, then the amount of metal used is minimized when ; =i~2.21 (e) If you look more closely at a real can, you will see that the top and bottom are formed by cutting disks with radius larger than 1' and folding them down. Taking this into account would increase the ratio more towards what we see in real cans. But more signicantly, we ought to take into account the cost of manufacture of the can. Let's assume most of the expense is incurred in joining the sides to the rims of the cans. If we cut the discs from hexagons (as above), then the total cost is proportional to 4\\/r2 + erh + k(47rr + h.) where k is the reciprocal of the elgnth that can be joined for the cost of one unit area of metal. Show that this expression is minimized when W _ h . 271' h/r '1" \"Eh/1" 4\\/ (f) Use deemos to plot W/k as a function of :1: = h/r and use your graph to explain why when a can is large or joining is cheap, we should make 11/? approximately 2.21. But when the can is small or joining is costly, h/r should be substantially larger