please please give it's answer perfectly and as soon as possible. I will rate positive

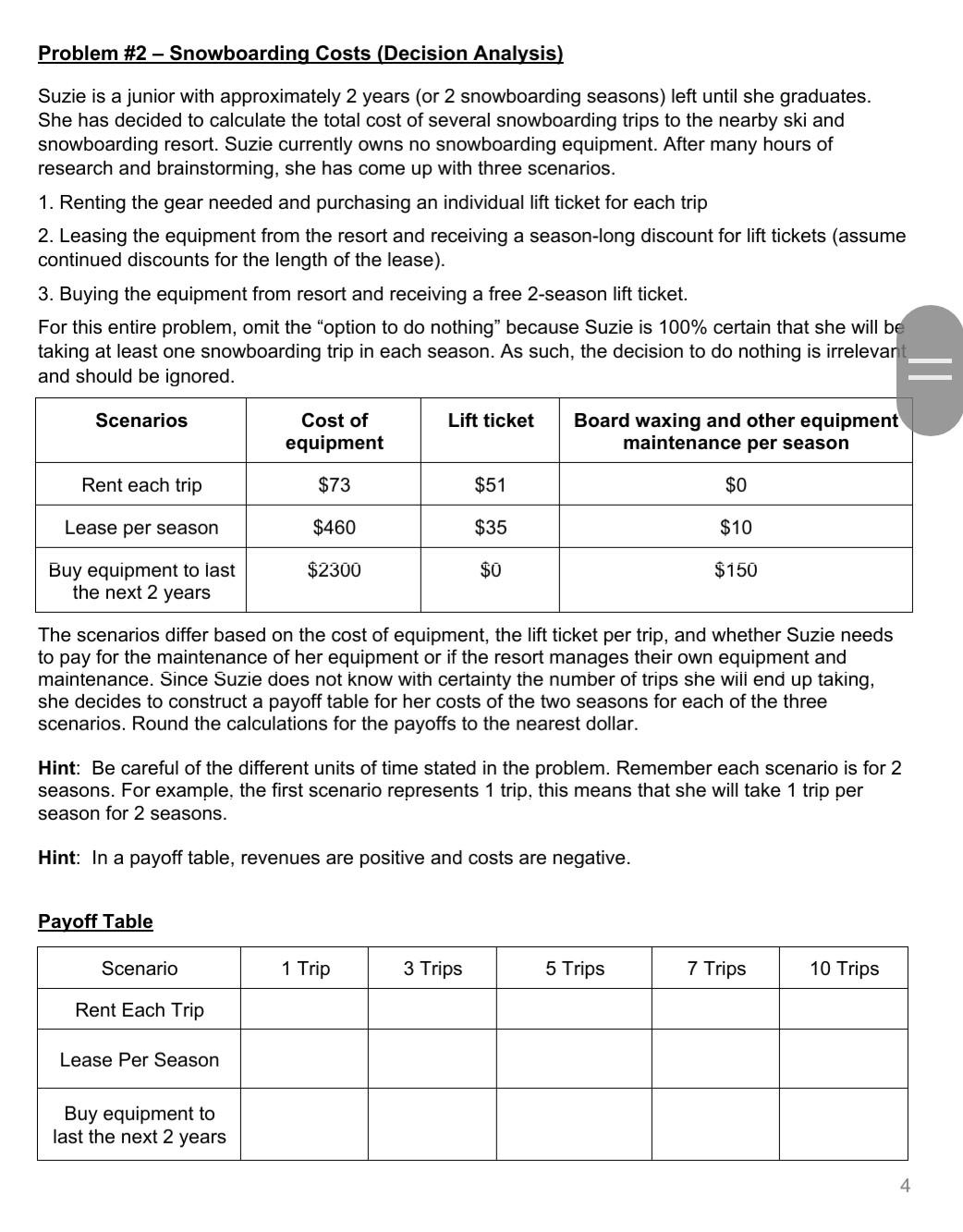
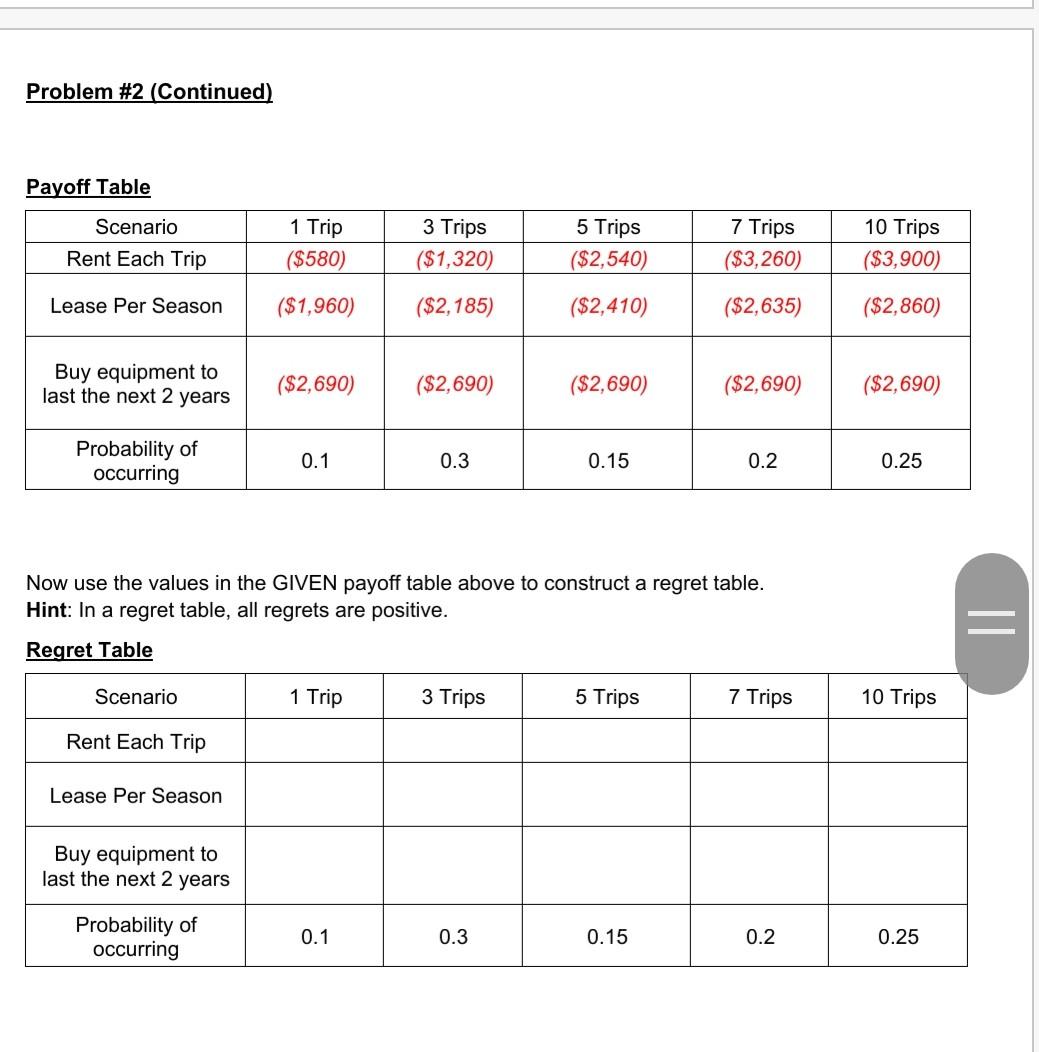
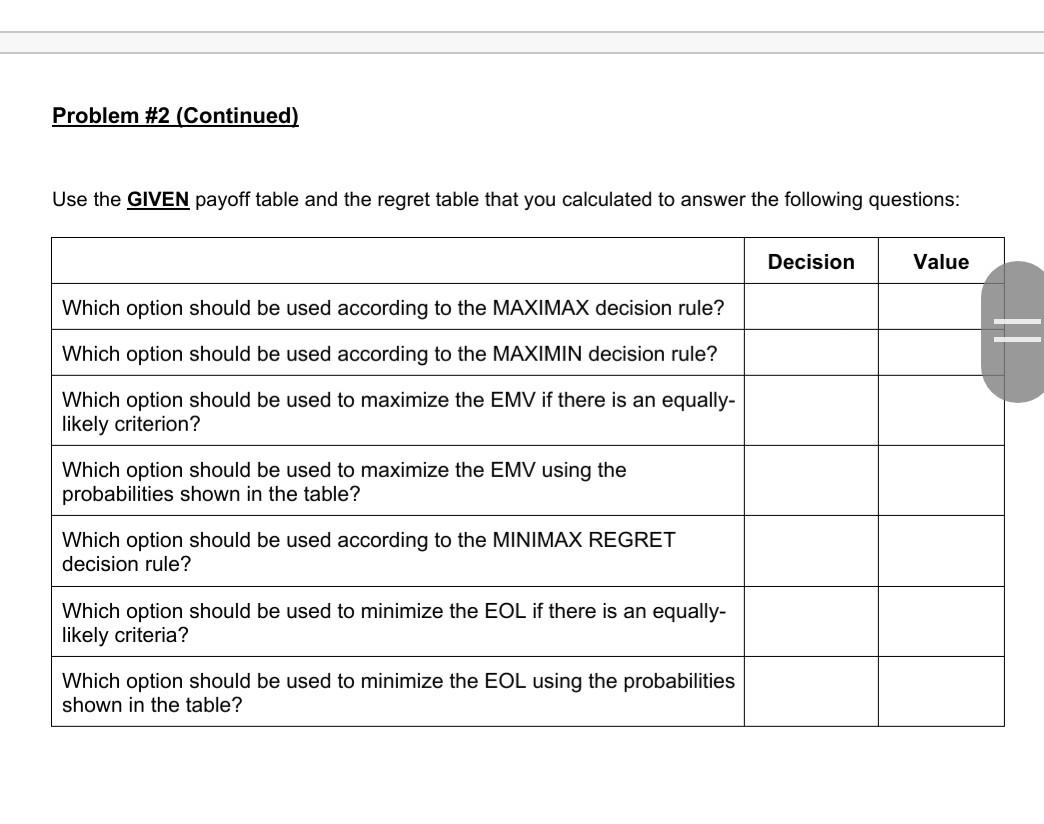
Problem #2 - Snowboarding Costs (Decision Analysis) Suzie is a junior with approximately 2 years (or 2 snowboarding seasons) left until she graduates. She has decided to calculate the total cost of several snowboarding trips to the nearby ski and snowboarding resort. Suzie currently owns no snowboarding equipment. After many hours of research and brainstorming, she has come up with three scenarios. 1. Renting the gear needed and purchasing an individual lift ticket for each trip 2. Leasing the equipment from the resort and receiving a season-long discount for lift tickets (assume continued discounts for the length of the lease). 3. Buying the equipment from resort and receiving a free 2-season lift ticket. For this entire problem, omit the "option to do nothing" because Suzie is 100% certain that she will be taking at least one snowboarding trip in each season. As such, the decision to do nothing is irrelevan and should be ignored. Scenarios Lift ticket Cost of equipment Board waxing and other equipment maintenance per season Rent each trip $73 $51 $0 Lease per season $460 $35 $10 $2300 $0 $150 Buy equipment to last the next 2 years The scenarios differ based on the cost of equipment, the lift ticket per trip, and whether Suzie needs to pay for the maintenance of her equipment or if the resort manages their own equipment and maintenance. Since Suzie does not know with certainty the number of trips she will end up taking, she decides to construct a payoff table for her costs of the two seasons for each of the three scenarios. Round the calculations for the payoffs to the nearest dollar. Hint: Be careful of the different units of time stated in the problem. Remember each scenario is for 2 seasons. For example, the first scenario represents 1 trip, this means that she will take 1 trip per season for 2 seasons. Hint: In a payoff table, revenues are positive and costs are negative. Payoff Table Scenario 1 Trip 3 Trips 5 Trips 7 Trips 10 Trips Rent Each Trip Lease Per Season Buy equipment to last the next 2 years 4. Problem #2 Continued) Payoff Table Scenario Rent Each Trip 1 Trip ($580) 3 Trips ($1,320) 5 Trips ($2,540) 7 Trips ($3,260) 10 Trips ($3,900) Lease Per Season ($1,960) ($2,185) ($2,410) ($2,635) ($2,860) Buy equipment to last the next 2 years ($2,690) ($2,690) ($2,690) ($2,690) ($2,690) Probability of occurring 0.1 0.3 0.15 0.2 0.25 Now use the values in the GIVEN payoff table above to construct a regret table. Hint: In a regret table, all regrets are positive. Regret Table II Scenario 1 Trip 3 Trips 5 Trips 7 Trips 10 Trips Rent Each Trip Lease Per Season Buy equipment to last the next 2 years Probability of occurring 0.1 0.3 0.15 0.2 0.25 Problem #2 (Continued) Use the GIVEN payoff table and the regret table that you calculated to answer the following questions: Decision Value Which option should be used according to the MAXIMAX decision rule? Which option should be used according to the MAXIMIN decision rule? Which option should be used to maximize the EMV if there is an equally- likely criterion? Which option should be used to maximize the EMV using the probabilities shown in the table? Which option should be used according to the MINIMAX REGRET decision rule? Which option should be used to minimize the EOL if there is an equally- likely criteria? Which option should be used to minimize the EOL using the probabilities shown in the table? Problem #2 - Snowboarding Costs (Decision Analysis) Suzie is a junior with approximately 2 years (or 2 snowboarding seasons) left until she graduates. She has decided to calculate the total cost of several snowboarding trips to the nearby ski and snowboarding resort. Suzie currently owns no snowboarding equipment. After many hours of research and brainstorming, she has come up with three scenarios. 1. Renting the gear needed and purchasing an individual lift ticket for each trip 2. Leasing the equipment from the resort and receiving a season-long discount for lift tickets (assume continued discounts for the length of the lease). 3. Buying the equipment from resort and receiving a free 2-season lift ticket. For this entire problem, omit the "option to do nothing" because Suzie is 100% certain that she will be taking at least one snowboarding trip in each season. As such, the decision to do nothing is irrelevan and should be ignored. Scenarios Lift ticket Cost of equipment Board waxing and other equipment maintenance per season Rent each trip $73 $51 $0 Lease per season $460 $35 $10 $2300 $0 $150 Buy equipment to last the next 2 years The scenarios differ based on the cost of equipment, the lift ticket per trip, and whether Suzie needs to pay for the maintenance of her equipment or if the resort manages their own equipment and maintenance. Since Suzie does not know with certainty the number of trips she will end up taking, she decides to construct a payoff table for her costs of the two seasons for each of the three scenarios. Round the calculations for the payoffs to the nearest dollar. Hint: Be careful of the different units of time stated in the problem. Remember each scenario is for 2 seasons. For example, the first scenario represents 1 trip, this means that she will take 1 trip per season for 2 seasons. Hint: In a payoff table, revenues are positive and costs are negative. Payoff Table Scenario 1 Trip 3 Trips 5 Trips 7 Trips 10 Trips Rent Each Trip Lease Per Season Buy equipment to last the next 2 years 4. Problem #2 Continued) Payoff Table Scenario Rent Each Trip 1 Trip ($580) 3 Trips ($1,320) 5 Trips ($2,540) 7 Trips ($3,260) 10 Trips ($3,900) Lease Per Season ($1,960) ($2,185) ($2,410) ($2,635) ($2,860) Buy equipment to last the next 2 years ($2,690) ($2,690) ($2,690) ($2,690) ($2,690) Probability of occurring 0.1 0.3 0.15 0.2 0.25 Now use the values in the GIVEN payoff table above to construct a regret table. Hint: In a regret table, all regrets are positive. Regret Table II Scenario 1 Trip 3 Trips 5 Trips 7 Trips 10 Trips Rent Each Trip Lease Per Season Buy equipment to last the next 2 years Probability of occurring 0.1 0.3 0.15 0.2 0.25 Problem #2 (Continued) Use the GIVEN payoff table and the regret table that you calculated to answer the following questions: Decision Value Which option should be used according to the MAXIMAX decision rule? Which option should be used according to the MAXIMIN decision rule? Which option should be used to maximize the EMV if there is an equally- likely criterion? Which option should be used to maximize the EMV using the probabilities shown in the table? Which option should be used according to the MINIMAX REGRET decision rule? Which option should be used to minimize the EOL if there is an equally- likely criteria? Which option should be used to minimize the EOL using the probabilities shown in the table