please read through the explanations to help answer the questions at the end, u(w)=log w and w = wealth pi is unknown?

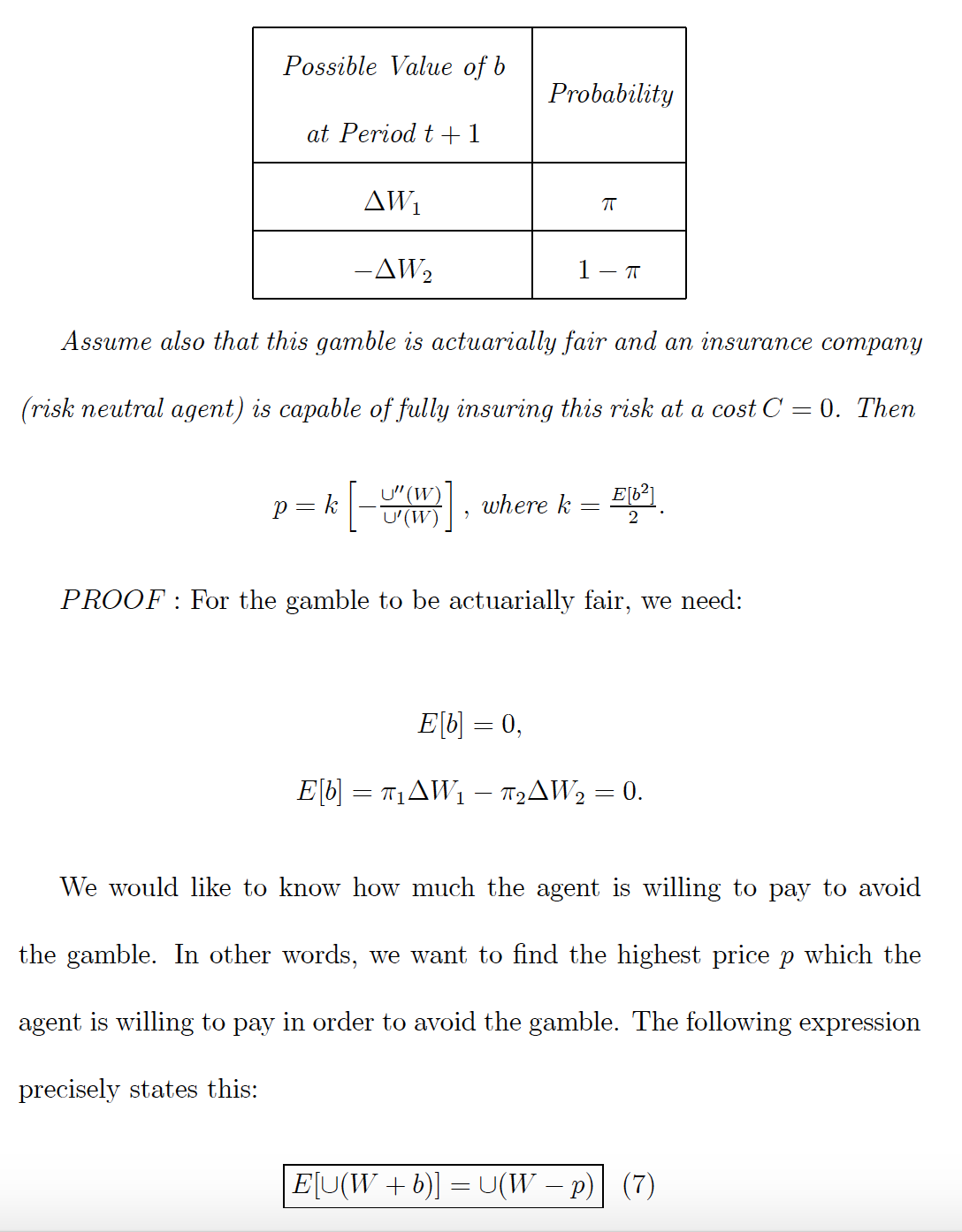
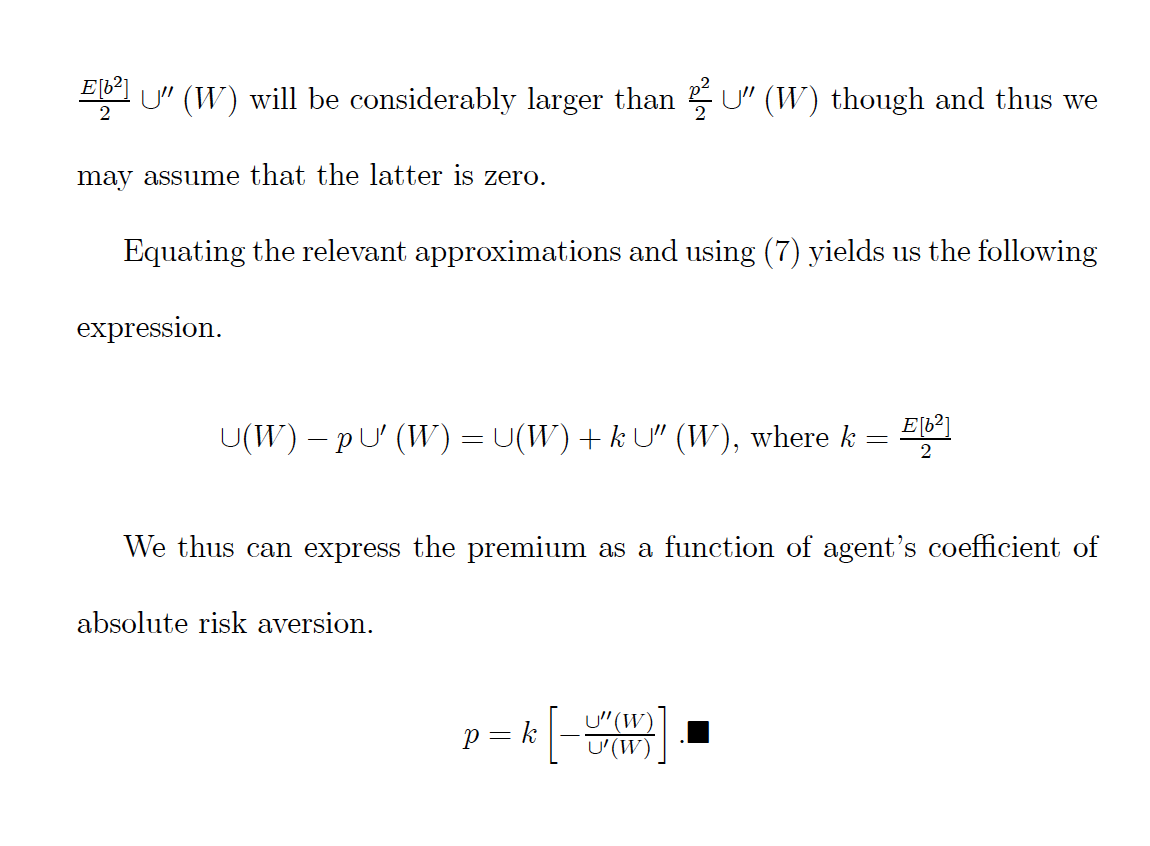
DEFINITION : Consider a gamble which pays X1 in state 1 with prob ability in and X2 in state 2 with probability 7T2. If the cost of the gamble is C', then the proposed gamble is actuarially fair if 1T1X1+7T2X2C Z 0. SO, we say that a gamble is actuarially fairj if the cost of the gamble is equal to the expected pay off of the gamble (e.g. zero prots if we think of insurance). THEOREM : Consider an individual facing an income risk as a result of which her wealth may either increase or decrease { shown in the table below): Possible Wealth Probability at Period t + 1, l/Vtirl \\V/ X1 \\_',_/ X2 In that case, the random variable b corresponding to this gamble is de- scribed by the table below Possible Value of b Probability at Period t + 1 Assume also that this gamble is actuarially fair and an insurance company (risk neutral agent) is capable of fully insuring this risk at a cost 0 : 0. Then Li" W E62 39 Z k [_ u'lWi] ? whale k : [T]- PROOF : For the gamble to be actuarially fair, we need: E[b] : o, E[b] : FIAl/I'fl WQAH/Tg : 0. We would like to know how much the agent is willing to pay to avoid the gamble. In other words, we want to nd the highest price p which the agent is willing to pay in order to avoid the gamble. The following expression precisely st ates this: |E[U(W + 5)] = U(W P)| (7) We will need the following formula for the 2nd order Taylor Expansion for twice differentiable function f in the neighborhood of a point zo: f ( z) = f(zo) + f' (zo)(z - 20) +. f" (20 (2 - 20)2. So, for f = U and zo = W we obtain U(W + b) = U(W) + bu' (W) + 5, U" (W) + higher order terms. Therefore, EU(W + b)] = EU(W) ] + E[b] u' (W) + bu" (W) + higher order terms, Similarly, we obtain U(W - p) = U(W) -pu' (W) + higher order terms. If we think of gambles with small premium, then we can ignore the second order term in the expansion of the right hand side of (7). Random variable b may still take large values even if the premium is small; the magnitude ofE[b2] 2 U\" (W) will be considerably larger than 3'32 U\" (W) though and thus we may assume that the latter is zero. Equating the relevant approximations and using (7) yields us the following expression. U(W) _ p or (W) : U(W) + k o\" (W), where k : Egg] We thus can express the premium as a function of agent's coefcient of absolute risk aversion