Please see below the slides on pricing vanilla swaps. Thank you.

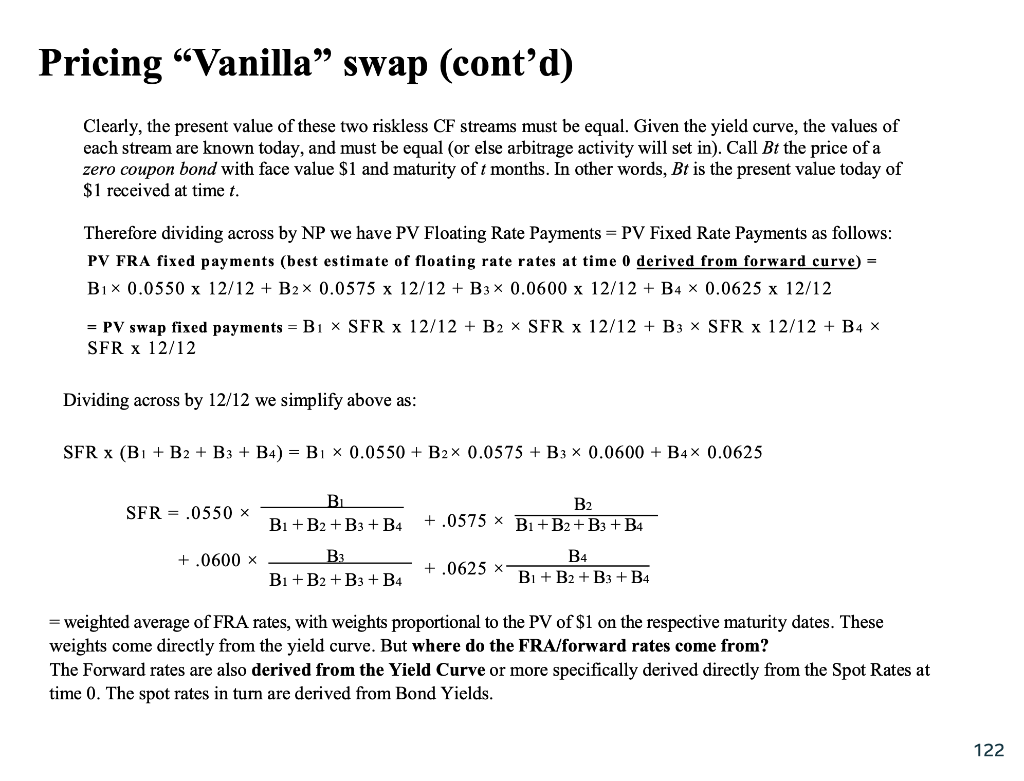
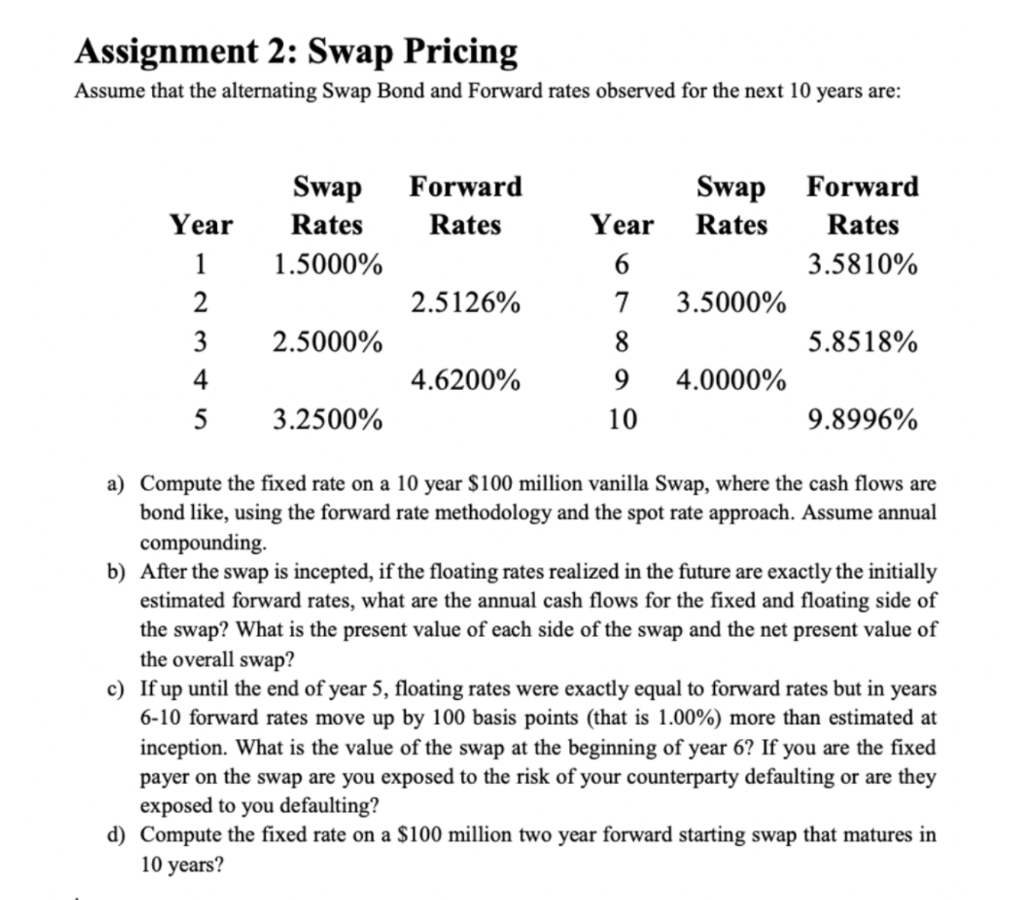
Assignment 2: Swap Pricing Assume that the alternating Swap Bond and Forward rates observed for the next 10 years are: Swap Rates 1.5000% Forward Rates Forward Rates 3.5810% 2.5126% Year 1 2 3 4 5 Swap Year Rates 6 7 3.5000% 8 9 4.0000% 10 2.5000% 5.8518% 4.6200% 3.2500% 9.8996% a) Compute the fixed rate on a 10 year $ 100 million vanilla Swap, where the cash flows are bond like, using the forward rate methodology and the spot rate approach. Assume annual compounding b) After the swap is incepted, if the floating rates realized in the future are exactly the initially estimated forward rates, what are the annual cash flows for the fixed and floating side of the swap? What is the present value of each side of the swap and the net present value of the overall swap? c) If up until the end of year 5, floating rates were exactly equal to forward rates but in years 6-10 forward rates move up by 100 basis points (that is 1.00%) more than estimated at inception. What is the value of the swap at the beginning of year 6? If you are the fixed payer on the swap are you exposed to the risk of your counterparty defaulting or are they exposed to you defaulting? d) Compute the fixed rate on a $100 million two year forward starting swap that matures in 10 years? Pricing Vanilla swap Although the swap fixed rate is quoted off the Treasury curve, it is priced off the forward rate curve. General principle: choose the swap fixed rate (SFR) that makes the market value of the swap = 0. If you are given (i.e., can observe) the forward rate curve, this computation is easy and is the main take away from these examples Start with the LIBOR forward curve. In this example we assume annual payments. The current annual forward LIBOR (0 x 12) is 5.50%. In 12 months the annual LIBOR rate that the market/forward curve expects based on what we know right now is 5.75% (12x24) or the rate starting in 12 months and ending in 24 months Period 0 x 12 12 x 24 24 x 36 36 x 48 Rate 5.50% (spot rate) 5.75% 6.00% 6.25% You can use these four Forward Rate Agreements (FRAS) to buy LIBOR x NP for the next four periods by agreeing to pay the stream of cash in the first column of the following table. You can also use a swap to buy LIBOR ~ NP for the next four periods by agreeing to pay a constant stream of cash equal to SFR x NP Period FRA cash flow Swap cash flow 1 .0550 x 1212 x NP SFR x 12/12 x NP 2 .0575 x 1212 x NP SFR x 12/12 x NP 3 .0600 x 1212 x NP SFR x 12/12 x NP 4 .0625 x 1212 x NP SFR x 12/12 x NP 121 Pricing "Vanilla" swap (cont'd) Clearly, the present value of these two riskless CF streams must be equal. Given the yield curve, the values of each stream are known today, and must be equal (or else arbitrage activity will set in). Call Bt the price of a zero coupon bond with face value $1 and maturity of t months. In other words, Bt is the present value today of $1 received at time t. Therefore dividing across by NP we have PV Floating Rate Payments = PV Fixed Rate Payments as follows: PV FRA fixed payments (best estimate of floating rate rates at time 0 derived from forward curve) = B1 * 0.0550 x 12/12 + B2 0.0575 x 12/12 + B3 x 0.0600 x 12/12 + B4 0.0625 x 12/12 = PV swap fixed payments = B1 * SFR x 12/12 + B2 X SFR x 12/12 + B3 X SFR x 12/12 + B4 X SFR x 12/12 Dividing across by 12/12 we simplify above as: SFR X (B1 + B2 + B3 + B4) = Bi X 0.0550 + B2 0.0575 + B3 x 0.0600 + B4% 0.0625 SFR = .0550 x BL B1 + B2 + B3 + B4 B2 +.0575 x B1 + B2+ B3 + B4 +.0600 X Bi + B2 +B3 + B4 +.0625 B4 Bi + B2 +B3 + B4 = weighted average of FRA rates, with weights proportional to the PV of $1 on the respective maturity dates. These weights come directly from the yield curve. But where do the FRA/forward rates come from? The Forward rates are also derived from the Yield Curve or more specifically derived directly from the Spot Rates at time 0. The spot rates in turn are derived from Bond Yields. 122 Pricing Vanilla swap (cont'd) You could also argue the reverse, assume Forward rates are the building blocks, from which spot rates are derived and then Bond Yields are the final result. It's a bit of a chicken and egg problem, as to which one comes first! Generally market participants think in terms of Bond or Spot yields. Following example shows how to derive Forward rates from Spot Rates: Time Forward Rate, ft Spot Rate y 5.500000% 1 5.500000% 2 5.624926% Zero Coupon Bond Price B 1/(1+.05500000)' = 0.947867 1/(1+.05624926) = 0.896328 1/(1+.05749803) = 0.845593 1/(1+.05874631)* = 0.795852 5.750000% 3 5.749803% 6.000000% 4 5.874631% 6.250000% Deriving Forward Rates - they are computed as Break Even rates as follows: fi:y = 5.500000% = fi f2: (1+f2) (1+y) = (1+ y2)2 since we know all the values except f2, we can easily rearrange the formula to compute f2, as follows: f2=(1+y2)2 / (1+y)- 1 = (1.05624926)2 / (1.05500000) -1 = 5.750000% f3 = (1+y3)3/(1+y2)2 1 = (1.05749803) 3 /(1.05624926)2 -1 = 6.000000% f4= (1+y4)4 /(1+y3)3 1 = (1.05874631)4/(1. 05749803) 3 -1 = 6.250000% Another quick way to derive forward rates is: fi=y = 5.500000% f2=B1/B2-1 = 0.947867 / 0.896328 -1 = 5.750000% f3 =B2/B3-1 = 0.896328 / 0.845593 - 1 = 6.000000% f4=B3/B4-1 = 0.845593 / 0.795852 - 1 = 6.250000% 123 Pricing "Vanilla" swap (cont'd) We can now solve for the Swap Fixed Rate as follows: SFR x (0.947867 +0.896328 + 0.845593 +0.795852) = 0.947867 0.0550 + 0.896328 x 0.0575 + 0.845593 x 0.0600 + 0.795852 x 0.0625 SFR x (0.947867 + 0.896328 + 0.845593 + 0.795852) = 0.204148 SFR = 0.204148 / 3.48564 = 5.8568% Also an alternative way to solve for the Swap Fixed Rate that in practice is used more often: SFR = (1 - B4) / (B1 + B2 +B3 + B4) = (1 - 0.795852) / 3.48564 = 5.8568% 124 Assignment 2: Swap Pricing Assume that the alternating Swap Bond and Forward rates observed for the next 10 years are: Swap Rates 1.5000% Forward Rates Forward Rates 3.5810% 2.5126% Year 1 2 3 4 5 Swap Year Rates 6 7 3.5000% 8 9 4.0000% 10 2.5000% 5.8518% 4.6200% 3.2500% 9.8996% a) Compute the fixed rate on a 10 year $ 100 million vanilla Swap, where the cash flows are bond like, using the forward rate methodology and the spot rate approach. Assume annual compounding b) After the swap is incepted, if the floating rates realized in the future are exactly the initially estimated forward rates, what are the annual cash flows for the fixed and floating side of the swap? What is the present value of each side of the swap and the net present value of the overall swap? c) If up until the end of year 5, floating rates were exactly equal to forward rates but in years 6-10 forward rates move up by 100 basis points (that is 1.00%) more than estimated at inception. What is the value of the swap at the beginning of year 6? If you are the fixed payer on the swap are you exposed to the risk of your counterparty defaulting or are they exposed to you defaulting? d) Compute the fixed rate on a $100 million two year forward starting swap that matures in 10 years? Pricing Vanilla swap Although the swap fixed rate is quoted off the Treasury curve, it is priced off the forward rate curve. General principle: choose the swap fixed rate (SFR) that makes the market value of the swap = 0. If you are given (i.e., can observe) the forward rate curve, this computation is easy and is the main take away from these examples Start with the LIBOR forward curve. In this example we assume annual payments. The current annual forward LIBOR (0 x 12) is 5.50%. In 12 months the annual LIBOR rate that the market/forward curve expects based on what we know right now is 5.75% (12x24) or the rate starting in 12 months and ending in 24 months Period 0 x 12 12 x 24 24 x 36 36 x 48 Rate 5.50% (spot rate) 5.75% 6.00% 6.25% You can use these four Forward Rate Agreements (FRAS) to buy LIBOR x NP for the next four periods by agreeing to pay the stream of cash in the first column of the following table. You can also use a swap to buy LIBOR ~ NP for the next four periods by agreeing to pay a constant stream of cash equal to SFR x NP Period FRA cash flow Swap cash flow 1 .0550 x 1212 x NP SFR x 12/12 x NP 2 .0575 x 1212 x NP SFR x 12/12 x NP 3 .0600 x 1212 x NP SFR x 12/12 x NP 4 .0625 x 1212 x NP SFR x 12/12 x NP 121 Pricing "Vanilla" swap (cont'd) Clearly, the present value of these two riskless CF streams must be equal. Given the yield curve, the values of each stream are known today, and must be equal (or else arbitrage activity will set in). Call Bt the price of a zero coupon bond with face value $1 and maturity of t months. In other words, Bt is the present value today of $1 received at time t. Therefore dividing across by NP we have PV Floating Rate Payments = PV Fixed Rate Payments as follows: PV FRA fixed payments (best estimate of floating rate rates at time 0 derived from forward curve) = B1 * 0.0550 x 12/12 + B2 0.0575 x 12/12 + B3 x 0.0600 x 12/12 + B4 0.0625 x 12/12 = PV swap fixed payments = B1 * SFR x 12/12 + B2 X SFR x 12/12 + B3 X SFR x 12/12 + B4 X SFR x 12/12 Dividing across by 12/12 we simplify above as: SFR X (B1 + B2 + B3 + B4) = Bi X 0.0550 + B2 0.0575 + B3 x 0.0600 + B4% 0.0625 SFR = .0550 x BL B1 + B2 + B3 + B4 B2 +.0575 x B1 + B2+ B3 + B4 +.0600 X Bi + B2 +B3 + B4 +.0625 B4 Bi + B2 +B3 + B4 = weighted average of FRA rates, with weights proportional to the PV of $1 on the respective maturity dates. These weights come directly from the yield curve. But where do the FRA/forward rates come from? The Forward rates are also derived from the Yield Curve or more specifically derived directly from the Spot Rates at time 0. The spot rates in turn are derived from Bond Yields. 122 Pricing Vanilla swap (cont'd) You could also argue the reverse, assume Forward rates are the building blocks, from which spot rates are derived and then Bond Yields are the final result. It's a bit of a chicken and egg problem, as to which one comes first! Generally market participants think in terms of Bond or Spot yields. Following example shows how to derive Forward rates from Spot Rates: Time Forward Rate, ft Spot Rate y 5.500000% 1 5.500000% 2 5.624926% Zero Coupon Bond Price B 1/(1+.05500000)' = 0.947867 1/(1+.05624926) = 0.896328 1/(1+.05749803) = 0.845593 1/(1+.05874631)* = 0.795852 5.750000% 3 5.749803% 6.000000% 4 5.874631% 6.250000% Deriving Forward Rates - they are computed as Break Even rates as follows: fi:y = 5.500000% = fi f2: (1+f2) (1+y) = (1+ y2)2 since we know all the values except f2, we can easily rearrange the formula to compute f2, as follows: f2=(1+y2)2 / (1+y)- 1 = (1.05624926)2 / (1.05500000) -1 = 5.750000% f3 = (1+y3)3/(1+y2)2 1 = (1.05749803) 3 /(1.05624926)2 -1 = 6.000000% f4= (1+y4)4 /(1+y3)3 1 = (1.05874631)4/(1. 05749803) 3 -1 = 6.250000% Another quick way to derive forward rates is: fi=y = 5.500000% f2=B1/B2-1 = 0.947867 / 0.896328 -1 = 5.750000% f3 =B2/B3-1 = 0.896328 / 0.845593 - 1 = 6.000000% f4=B3/B4-1 = 0.845593 / 0.795852 - 1 = 6.250000% 123 Pricing "Vanilla" swap (cont'd) We can now solve for the Swap Fixed Rate as follows: SFR x (0.947867 +0.896328 + 0.845593 +0.795852) = 0.947867 0.0550 + 0.896328 x 0.0575 + 0.845593 x 0.0600 + 0.795852 x 0.0625 SFR x (0.947867 + 0.896328 + 0.845593 + 0.795852) = 0.204148 SFR = 0.204148 / 3.48564 = 5.8568% Also an alternative way to solve for the Swap Fixed Rate that in practice is used more often: SFR = (1 - B4) / (B1 + B2 +B3 + B4) = (1 - 0.795852) / 3.48564 = 5.8568% 124