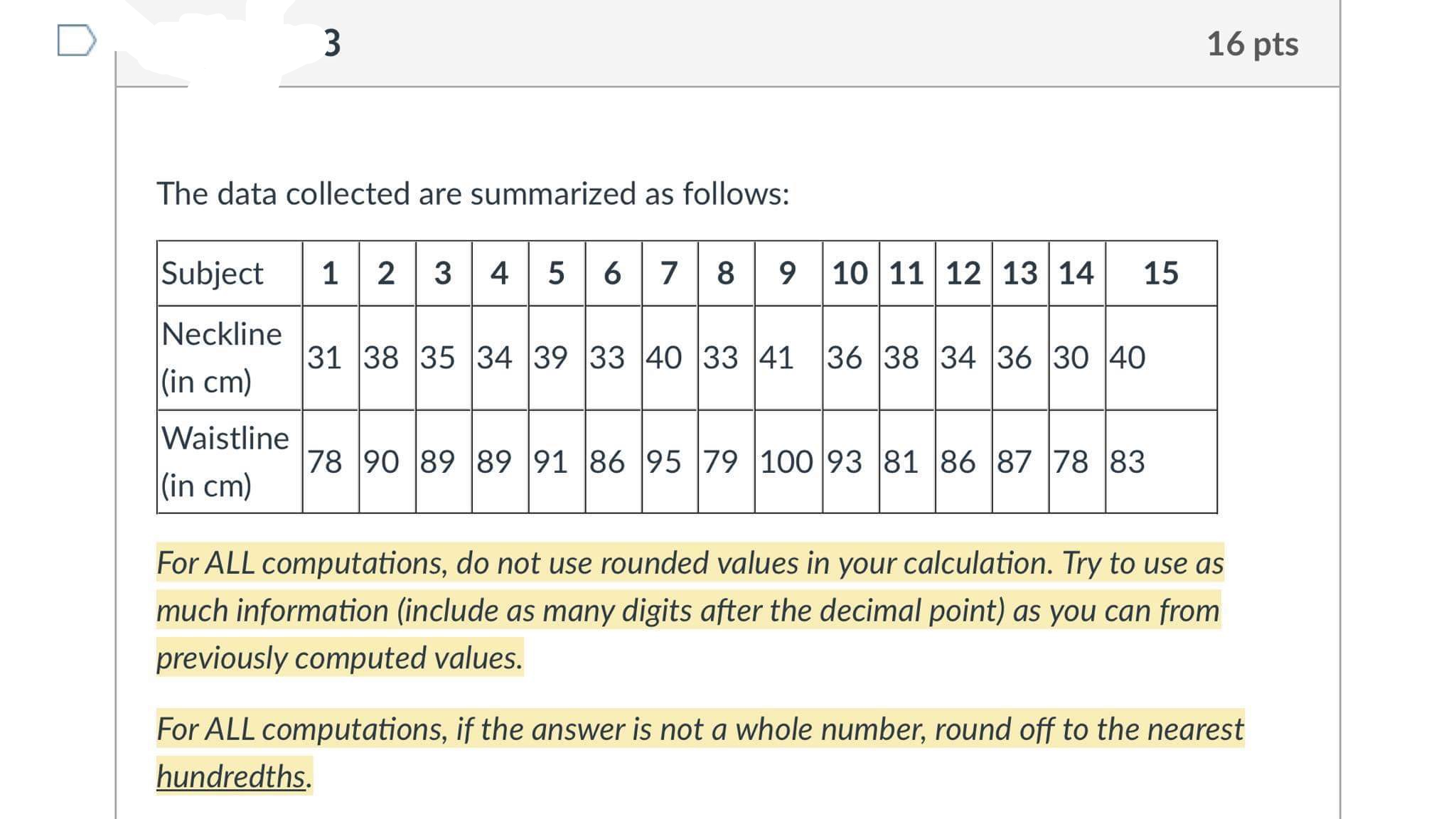


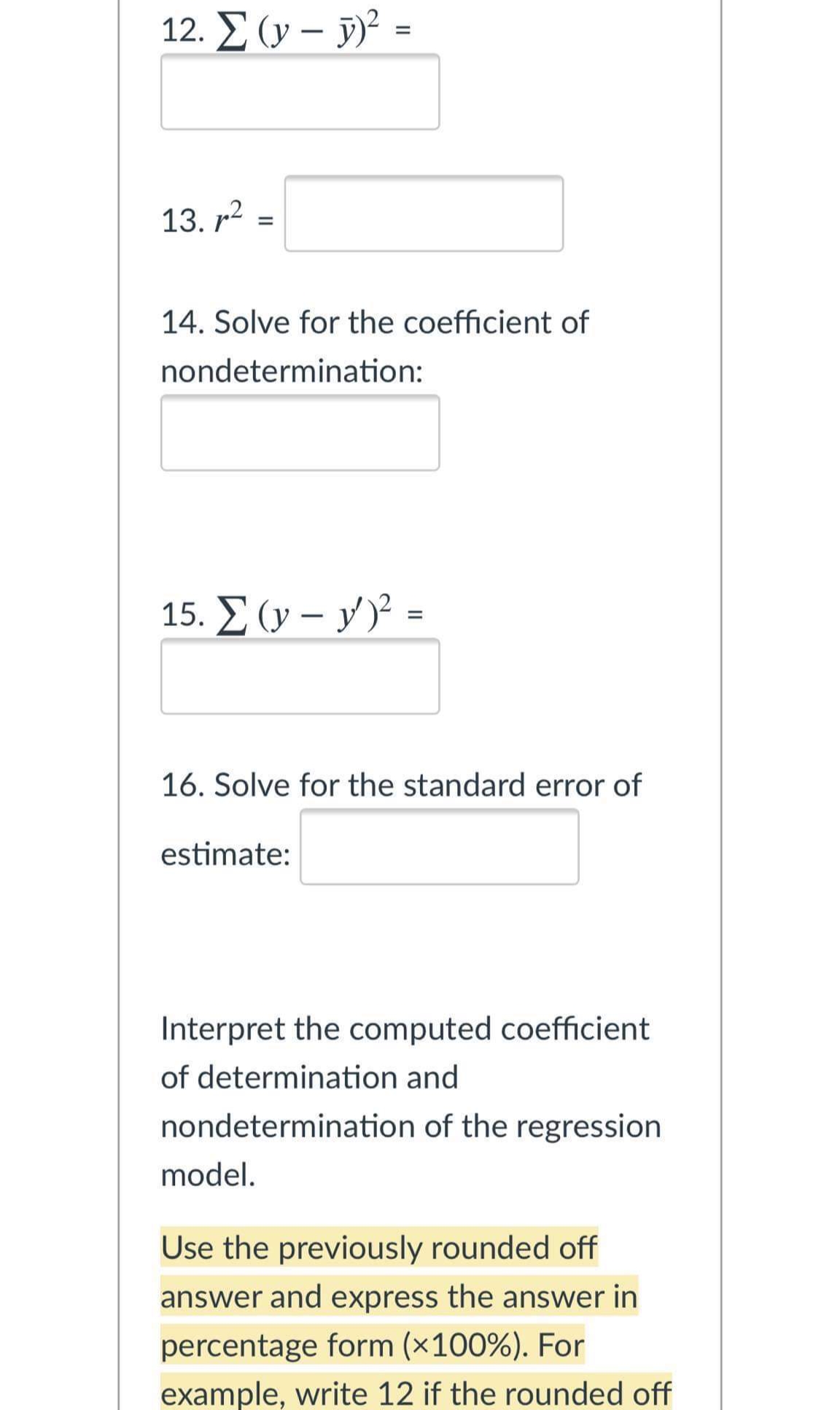
Please show all the necessary solutions.
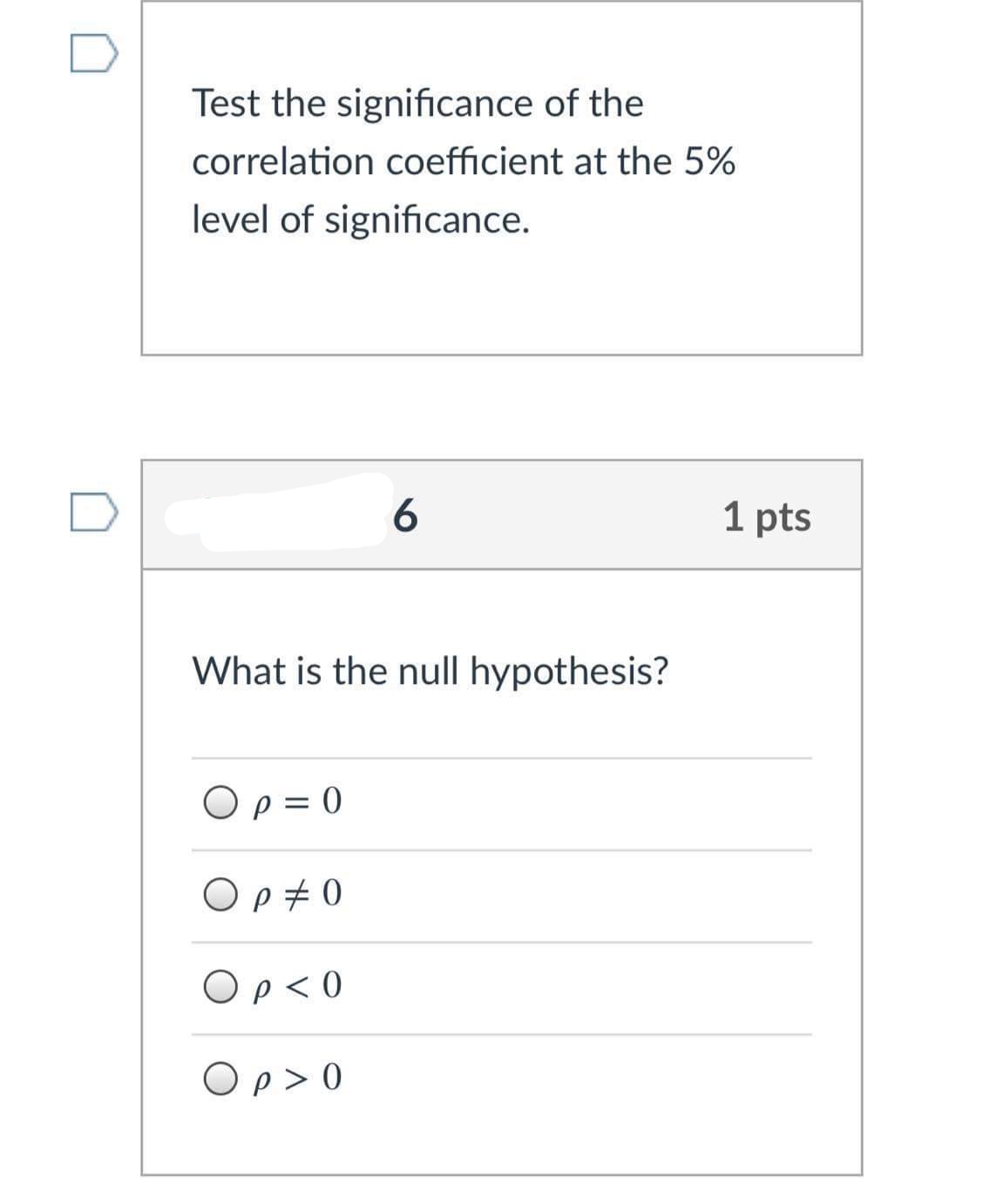
Objectives 1. Compute and interpret correlation coefficient. 2. Test the significance of correlation coefficient. 3. Estimate a simple linear regression model. 4. Test the significance of the regression constant and coefficient. 5. Interpret the overall fit of the simple linear regression model. Materials 1. z-table 2. t-table 3. calculator D As practiced by many in buying skirts or pants, they use the neckline to check if the waistline of the skirts or pants fits them. We would like to study this claim by using a random sample of neckline and waistline of 15 people. The data collected are summarized in Question 3.D 9 1 pts Using the test statistic computed in Question 3, what is the appropriate decision? O Reject Ho. O Fail to reject Ho. D 10 1 pts Conclusion: O At the 5% level of significance, there is a significant linear relationship between the neckline and waistline. O At the 5% level of significance, there is no significant linear relationship between the neckline and waistline.D 3 16 pts The data collected are summarized as follows: Subject 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 Neckline 31 38 35 34 39 33 40 33 41 36 38 34 36 30 40 (in cm) Waistline 78 90 89 89 91 86 95 79 100 93 81 86 87 78 83 (in cm) For ALL computations, do not use rounded values in your calculation. Try to use as much information (include as many digits after the decimal point) as you can from previously computed values. For ALL computations, if the answer is not a whole number, round off to the nearest hundredths.D 3 pts Construct a scatter plot for the waistline and neckline using the data presented in Question 3. Upload a jpeg file of your plot. Upload Choose a File 66876EB6-6C54-4166- B908-7EF4D709D3A6.jpeg has been removed. D 2 2 pts What does the scatter plot in Question 1 tell you about the possible relationship between neckline and waistline? Briefly answer in 1-2 sentences.Compute the sample correlation coefficient of neckline and waistline: 1. SPXY 2. SSx 3. SSY = 4. r= Test the significance of the correlation coefficient at the 5% level of significance. 5. Compute the test statistic: Estimate the regression line of neckline and waistline. Using the model, we wish to estimate the waistline given information about the neckline. Solve for the y' intercept, a and the slope, b. 6. a =7. b = 8-9. Regression line equation: waistline' = + neckline Reminder: Do not use rounded values in your calculation. When computing for the predicted value y', use the actual values of the a and b, not the rounded values in items 6 and 7. 10. If the neckline is 37 cm, estimate the waistline (in cm) using the regression model: Solve for the coefficient of determination: 11. E ( ) - D)2 =12. E ( y - J)2 = 13. r2 = 14. Solve for the coefficient of nondetermination: 15. E ( y - y)2 = 16. Solve for the standard error of estimate: Interpret the computed coefficient of determination and nondetermination of the regression model. Use the previously rounded off answer and express the answer in percentage form (x100%). For example, write 12 if the rounded offexample, write 12 if the rounded off answer was 0.12. 17-18. % of the variation of y is due to the variation of x; % is due to chance. D - 2 pts Interpret the sample correlation coefficient of neckline and waistline: The value of r suggests that there is a [ Select ] [ Select ] linear relationship.D 5 2 pts Does the result in Question 3 conform with the scatter plot in Question 1? Briefly justify your answer in 1-2 sentences. Edit View Insert Format Tools Table 12pt V Paragraph V ... i O words > p ... .D Test the significance of the correlation coefficient at the 5% level of significance. D 6 1 pts What is the null hypothesis? Op = 0 Op + 0 Op 0D 7 1 pts What is the alternative hypothesis? What is the critical value in the decision rule? if the critical value follows the z- distribuon, round off to the nearest hundredths. If the critical value follows the t distribution, round off to the nearest thousandths



























