Please solve (d)
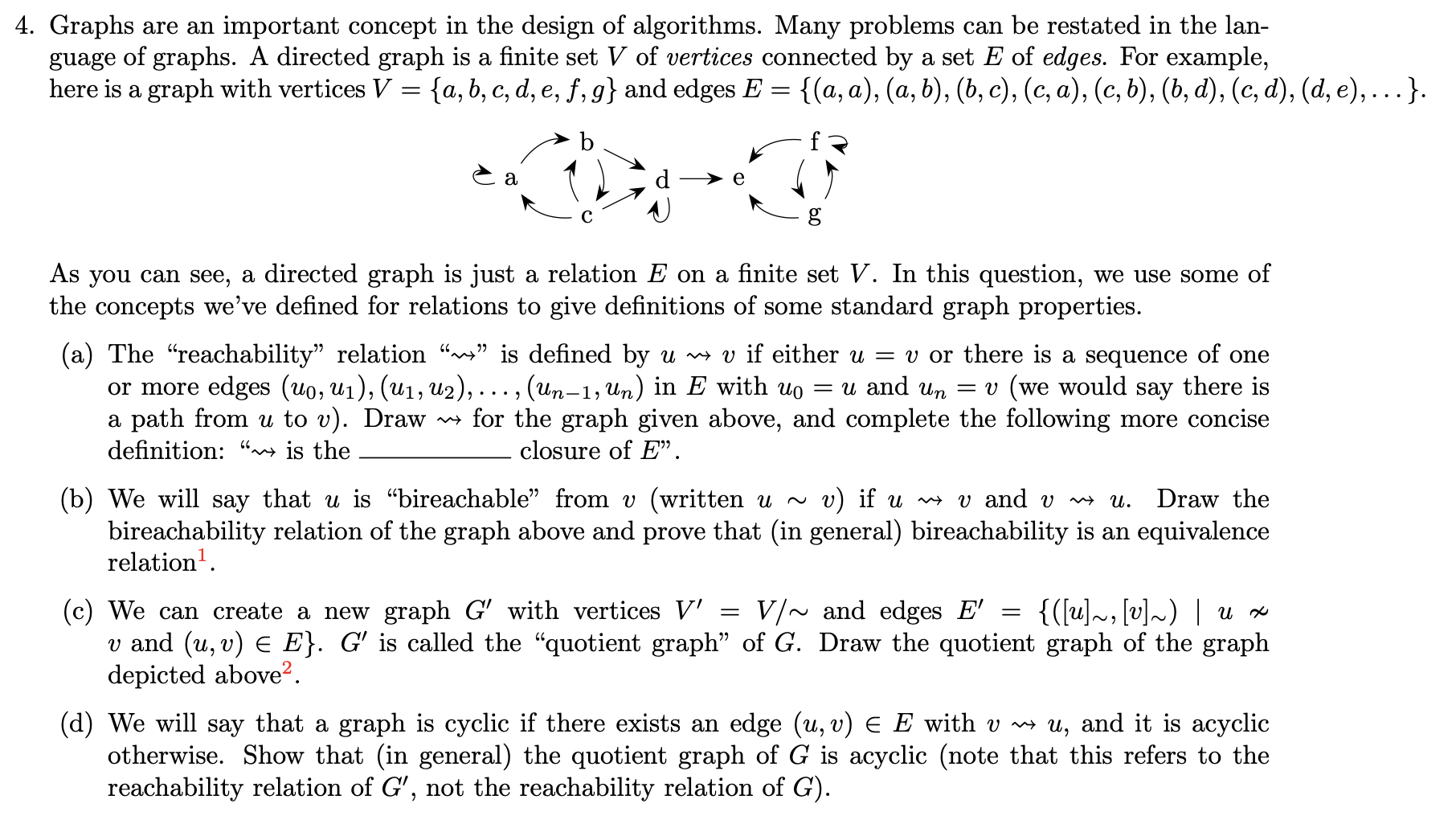
4. Graphs are an important concept in the design of algorithms. Many problems can be restated in the lan- guage of graphs. A directed graph is a finite set V of vertices connected by a set E of edges. For example, here is a graph with vertices V = {a,b, c, d, e, f,g} and edges E = {(a, a), (a,b), (b, c), (c, a), (c,b), (b, d), (c,d), (d, e),...}. >b riz a T d e C As you can see, a directed graph is just a relation E on a finite set V. In this question, we use some of the concepts we've defined for relations to give definitions of some standard graph properties. (a) The reachability relation my is defined by u my v if either u = v or there is a sequence of one or more edges (10, U1), (u1, 42),..., (Un1, Un) in E with uo = u and Un = v (we would say there is a path from u to v). Draw my for the graph given above, and complete the following more concise definition: my is the - closure of E. (b) We will say that u is bireachable from v (written u ~ v) if u my v and v my U. Draw the bireachability relation of the graph above and prove that (in general) bireachability is an equivalence relation?. (c) We can create a new graph G' with vertices V' = V/~ and edges E = {(fulm, ulu) | ux v and (u, v) E E}. G' is called the quotient graph of G. Draw the quotient graph of the graph depicted above. (d) We will say that a graph is cyclic if there exists an edge (u, v) E E with v vy U, and it is acyclic otherwise. Show that (in general) the quotient graph of G is acyclic (note that this refers to the reachability relation of G', not the reachability relation of G). 4. Graphs are an important concept in the design of algorithms. Many problems can be restated in the lan- guage of graphs. A directed graph is a finite set V of vertices connected by a set E of edges. For example, here is a graph with vertices V = {a,b, c, d, e, f,g} and edges E = {(a, a), (a,b), (b, c), (c, a), (c,b), (b, d), (c,d), (d, e),...}. >b riz a T d e C As you can see, a directed graph is just a relation E on a finite set V. In this question, we use some of the concepts we've defined for relations to give definitions of some standard graph properties. (a) The reachability relation my is defined by u my v if either u = v or there is a sequence of one or more edges (10, U1), (u1, 42),..., (Un1, Un) in E with uo = u and Un = v (we would say there is a path from u to v). Draw my for the graph given above, and complete the following more concise definition: my is the - closure of E. (b) We will say that u is bireachable from v (written u ~ v) if u my v and v my U. Draw the bireachability relation of the graph above and prove that (in general) bireachability is an equivalence relation?. (c) We can create a new graph G' with vertices V' = V/~ and edges E = {(fulm, ulu) | ux v and (u, v) E E}. G' is called the quotient graph of G. Draw the quotient graph of the graph depicted above. (d) We will say that a graph is cyclic if there exists an edge (u, v) E E with v vy U, and it is acyclic otherwise. Show that (in general) the quotient graph of G is acyclic (note that this refers to the reachability relation of G', not the reachability relation of G)







