please solve this
\
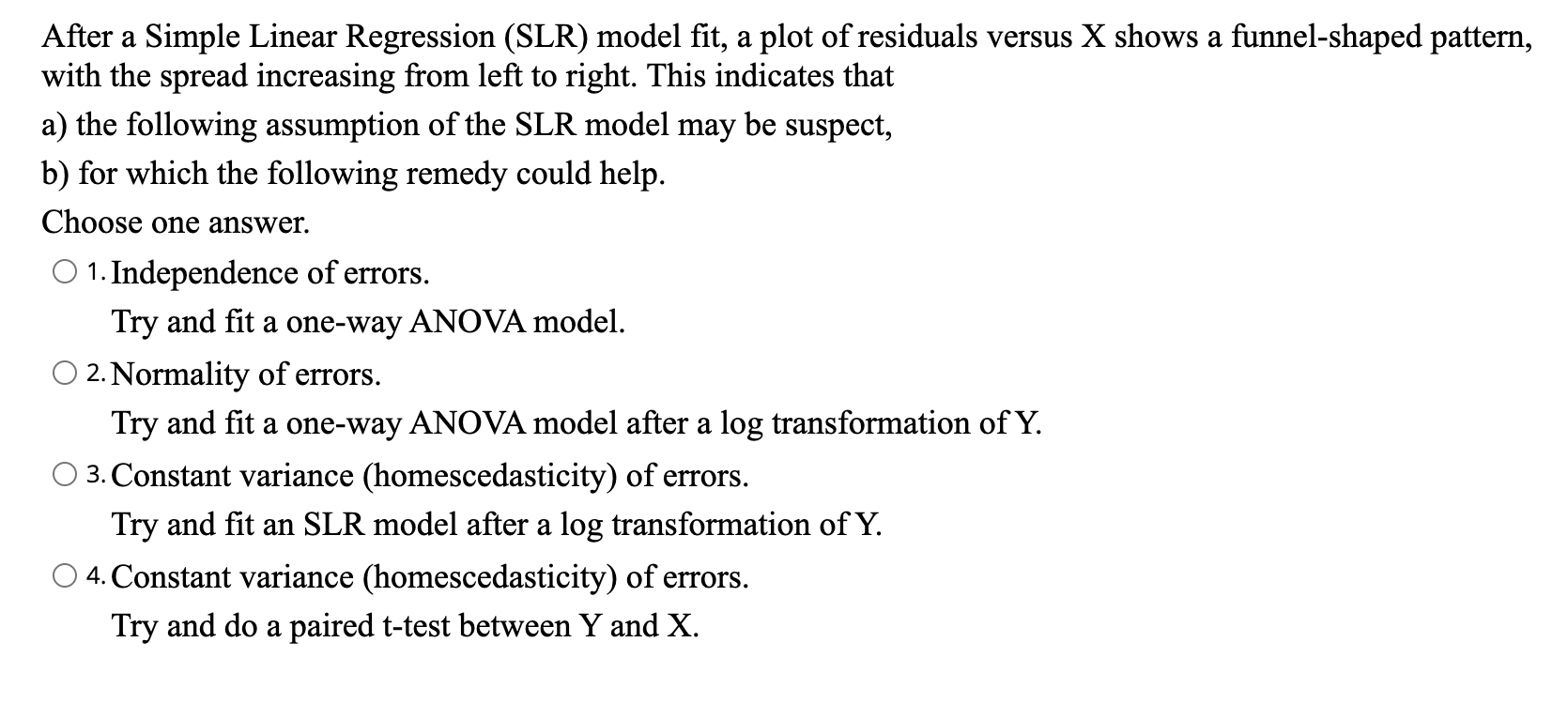
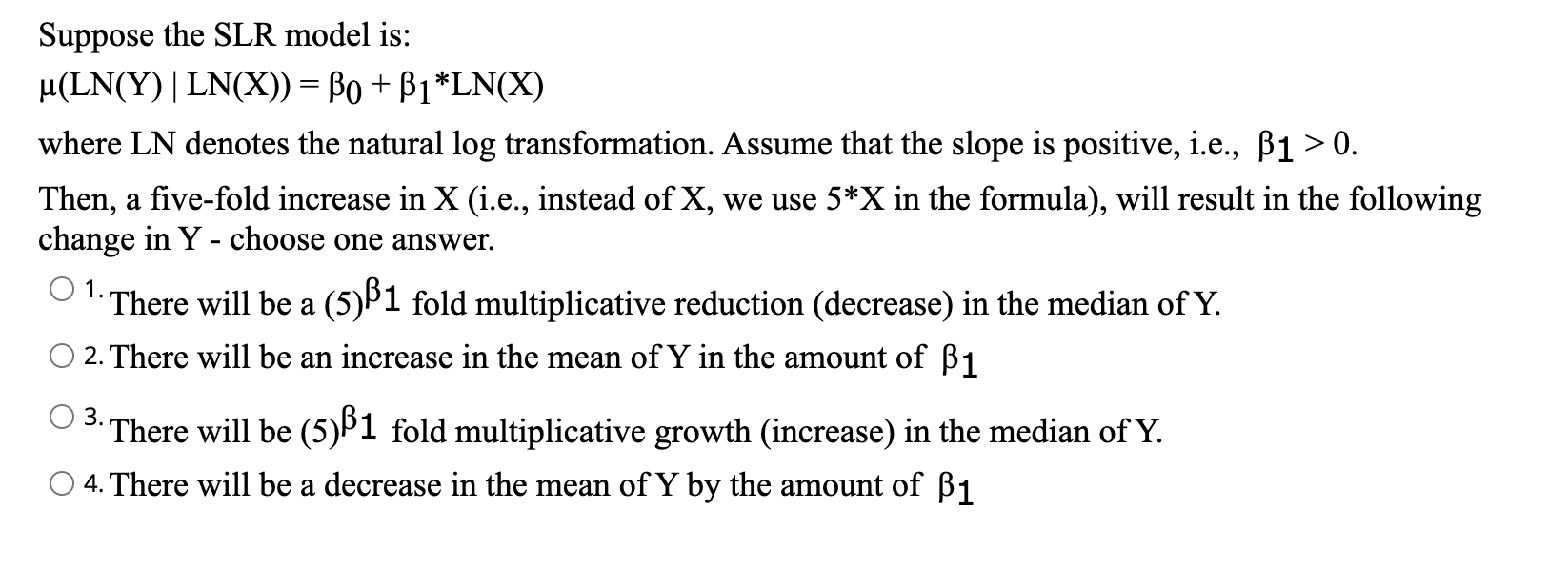
After a Simple Linear Regression (SLR) model fit, a plot of residuals versus X shows a funnel-shaped pattern, with the spread increasing from left to right. This indicates that a) the following assumption of the SLR model may be suspect, b) for which the following remedy could help. Choose one answer. O 1. Independence of errors. Try and fit a one-way ANOVA model. O 2. Normality of errors. Try and fit a one-way ANOVA model after a log transformation of Y. O 3. Constant variance (homescedasticity) of errors. Try and fit an SLR model after a log transformation of Y. O 4. Constant variance (homescedasticity) of errors. Try and do a paired t-test between Y and X.True or False? While fitting an SLR model, you must carry out both a t-test (with d.f. = n-2) and an F-test (with num. d.f. =1, and denom. d.f. = n-2) for testing Ho : B1 =0, because these two tests always give us different conclusions. That is, the t-test may suggest that you reject Ho at the 5% level of significance, whereas the F-test may suggest that you do not reject Ho at the 5% level of significance. O True O FalseSuppose there are n cases in the data set and you fit an SLR model of Y on X. In the t-test for the slope, Ho: 1 = 0 versus H1: Bj neq 0 in this SLR model, the d.f. associated with SE(B, ) is O1. n- 1 O 2. n - 2 O 3.2 4. nSuppose the SLR model is: \"(LN(Y) | LN(X)) = I30 + [31*LN(X) where LN denotes the natural log transformation. Assume that the slope is positive, i.e., B1 > 0. Then, a ve-fold increase in X (i.e., instead of X, we use 5*X in the formula), will result in the following change in Y - choose one answer. 0 1'There will be a (5)1 fold multiplicative reduction (decrease) in the median of Y. O 2. There will be an increase in the mean of Y in the amount of B1 0 3' There will be (5)51 fold multiplicative growth (increase) in the median of Y. O 4. There will be a decrease in the mean of Y by the amount of B1 Suppose you are given the data below and asked to fit an SLR model of Y on X. - U W N W UL awUANEX True or False. Even if we replace the first data pair (1, 5) by (1, 45), the estimated slope of the fitted line will be nearly the same and not be much affected. O True O FalseIn order to satisfy the normality assumption in an SLR model, 0 the scatterplot of Y versus X must show a linear pattern. 0 the sample size n must exceed 10 O the normal probability plot of residuals must show a straight line pattern. 0 the normal probability plot of residuals must have a random scatter with no pattern