please use r

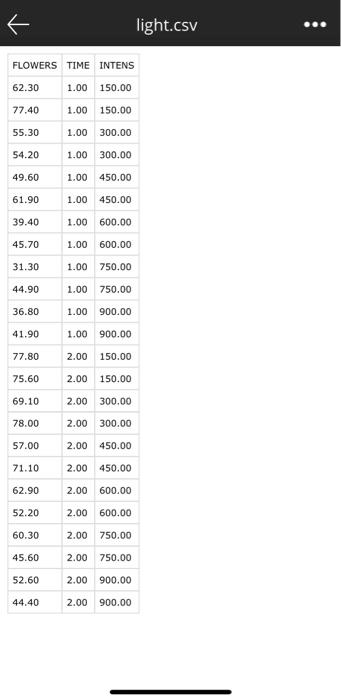
Experiment and data An experiment is conducted involving a plant called Meadowfoam. The goal of the experiment was to assess how light intensity (INTENS) and timing (TIME) of light application can effect the average number of flowers (FLOWERS) yielded by growing Meadowfoam plants. The data can be found in light.csv. Download the data set and import it into R. The data set consists of 3 variables: ## 'data.frame': 24 obs. of 3 variables: ## $ FLOWERS: num 62.3 77.4 55.3 54.2 49.6 61.9 39.4 45.7 31.3 44.9... ## $ TIME : num 1 1 1 1 1 1 1 111 ... ## $ INTENS : num 150 150 300 300 450 450 600 600 750 750 ... FLOWERS (F)- is the average number of flowers yielded under each condition TIME (T) - although it appears as numbers, they represent two categories (treat this as a factor) of the timing of when light treatment is applied to the plants. 1 represents applying light treatment at photoperiodic floral induction (PFI) and 2 represents applying the light treatment 24 days before PFI. PFI is the time at which the photo period is increased from 8 to 16 hours per day to induce flowering. INTENS (1) - is the intensity of the light treatment in umol/m2/sec. Question 1 a categorical variable regression: For this question treat level of light intensity INTENS as a factor. a. Fit the following regression equation and provide a summary of the regression model (summary()) model 1: F = Bo + B1(1300) + B2(1450) + B3(1600) + Bs(1750) + B3(1900) + Where: 1300 is an indicator variable of light intensity of 300 or not. 1450 is an indicator variable of light intensity of 450 or not. 1600 is an indicator variable of light intensity of 600 or not. 1750 is an indicator variable of light intensity of 750 or not. 1900 is an indicator variable of light intensity of 900 or not. b. Write out the fitted regression equation. c. What is the estimate and interpretation of Bo? d. What is the estimate and interpretation of B1? e. What is the estimate and interpretation of B.? light.csv FLOWERS TIME INTENS 62.30 1.00 150.00 77.40 1.00 150.00 55.30 1.00 300.00 54.20 1.00 300.00 49.60 1.00 450.00 61.90 1.00 450.00 39.40 1.00 600.00 45.70 1.00 600.00 31.30 44.90 36.80 1.00 750.00 1.00 750.00 1.00 900.00 1.00 900.00 2.00 150.00 2.00 150.00 41.90 77.80 75.60 69.10 2.00 300.00 78.00 2.00 300.00 57.00 2.00 450.00 71.10 2.00 450.00 62.90 2.00 600.00 52.20 60.30 2.00 600.00 2.00 750.00 2.00 750.00 2.00 900.00 45.60 52.60 44.40 2.00 900.00