pls help me answer the five (5) "check progress" and what can do part huhu
basically, you will just help me answer 6 questions only. the check progress and what can do part. disregard the last part thanks.
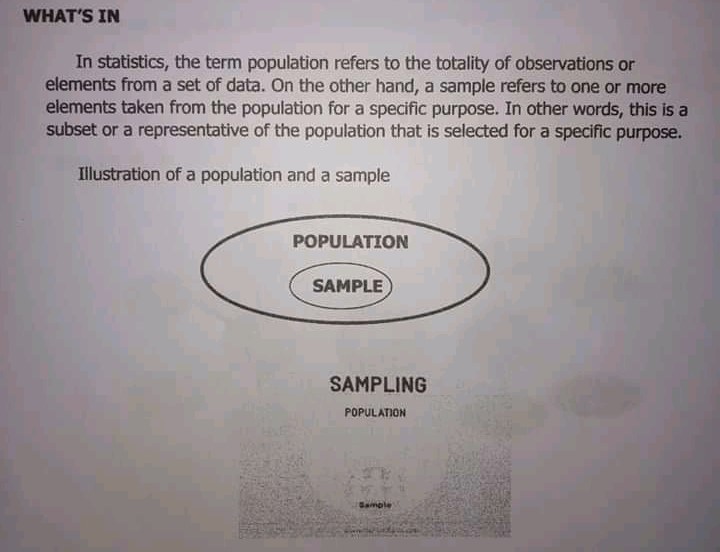
WHAT'S IN In statistics, the term population refers to the totality of observations or elements from a set of data. On the other hand, a sample refers to one or more elements taken from the population for a specific purpose. In other words, this is a subset or a representative of the population that is selected for a specific purpose. Illustration of a population and a sample POPULATION SAMPLE SAMPLING POPULATIONIn most studies, only a sample of the population is considered. This saves time, money, and effort on the part of the researcher. In medical technology, only a sample of blood is needed to determine the blood type of a patient. Also, in microbiology also a sample of water is needed to determine the potable quality of water from a pumping station. In some studies, however, it is Inevitable to include all the members of the population. One Illustration is a government census, which surveys the whole population in order to get necessary information. PARAMETER VERSUS STATISTIC Parameter - a numerical measure that describes the whole population. Statistic - a numerical description of the sample Example: Identify the parameter and the statistic used in the study. The Food and Nutrition Research Institute of the Department of Science and Technology ( FNRI - DOST ) surveyed 14 million Filipino adults aged 20 and above and determine that 80% of Filipino adults are at risk of hypertension. Answer: The parameter is the percentage of adults at risk of hypertension out of all Filipino adults aged 20 and above. The statistic is the percentage of 80% obtained from the sample of 14 million adults. CHECK YOUR PROGRESS Identify the parameter and the statistic used in the study. A researcher wants to estimate the average death age of Filipino women in the last decade and from a sample of 100 deaths, he obtained a sample mean age of 73. WHAT'S NEW SAMPLING TECHNIQUESHighly effective Random Sample is selected Very small sample when all subjects Bide introduces Sampling In a random fashion participate In data sample error colfection The person applying Accurate and this compling effective method should representation HAVE BroDEF Stratified Represents specific of all subgroups understanding of Sampling subgroups or strato Accurate the subgroups estimates in otherwise case of similarity misrepresentation or dissimilarity of sample con cause analytical fallacy Includes every Nth Systematic observation of the Time- and cost May result in high effective sompling biany Sampling population in the Efficient periodicity exists Cluster of High sampling observations Time- and costs errors are observed Cluster Sampling representing the efective In this method population are Identified as sample Efficient compared to other sampling methods population Figure: 1 10 Stratified Quota Systematic A random sample A random sample of a Selection every Selected from tired number taken nith person - eg. different groups. from each group - UR Every 10" person "2 Age groups 10 20 veur akis 10 50 to pass a shop numbers sampled year olds from bach group is proportional to Random Population are sampled Roun site - ag. 1 fromd group of 10. SAMPLING randomly - may be 3 from a group of determined using Purulom number generators - if 3, 9. and Convenience 21 ate generated then The sample taken is chosen at one that" of and 21 place at one time -therefore those people, for cumple. people present at that time are walk past a shop are jumpled sampled Types of Non-Probability Sampling (II) - Snowball Sampling Selection of additional respondents is based on referrals from the initial respondents. Used to sample from low incidence or rare populations. . Quota Sampling - The population is divided into cells on the basis of relevant control characteristics. A quota of sample units is established for each cell. - A convenience sample is drawn for each cell until the quota is met. (similar to stratified sampling)Convenience sampling is used in exploratory research for getting a gross esteratco' the results. withoutincurring the cost or lime .cauiron to selecta random samples Differentsampling is an extension of convenience Sampling For example, one may decide to draw the entire sample from one "representative" college. even though the population includes all colleges. Example: Identify the sampling technique illustrated below. 1.) A teacher assigned class numbers to each student and then spin a number roulette to identify the members of the sample. 2.) The teacher chose the top three students of the batch to represent the school in a regional quiz show. Answer: 1.) simple random sampling CHECK YOUR PROGRESS Do # 2, on the above example. WHAT IS IT Read "INTRODUCTION TO SAMPLING THEORY" pages 111 - 133 textbook (GEN. MATH - DIWA LEARNING SYSTEM INC. Copyright 2016) WHAT'S MORE SAMPLING DISTRIBUTION Is a distribution that shows the frequency with which values of statistics are observed when all the possible random samples are drawn from a given population. Example 1: A sample with size n = 3 is drawn from the set 5, 6, 8, 12, and 20. Construct the sampling distribution of the medians. Solution: Using combination, there are , Cran! / r! ( n- r ) 1 5 C3 = 5! / 31 ( 5 - 3 )! = 51 / 31 ( 21) = 20/2 = 10. There are 10 possible samples with size n = 3. The table below gives all these 10 samples and their corresponding medians. Sample Median 5, 6, 8 6 5, 6, 12 6 5, 6, 20 6 5, 8, 12 8 5, 8, 20 8 5, 12, 20 12 6, 8, 12 6, 8, 20 6, 12, 20 8, 12, 20Sample x Frequency 6 12 In sampling distribution, the standard deviation is usually called standard error. Example 2: Find the mean and standard error of the sampling distribution of the medians given in the example above. Solution: The mean of the sampling distribution is Hx = 6(3) + 8(4) + 12(3) = 86/10 = 8.6 10 Calculating the standard error of the distribution X X - Ux (X - (x ) 2 f ( x - [x ) ? 6 3 -2.6 6.76 20.28 8 4 -0.6 0.36 1.44 12 3 3.4 11.56 34.68 Total 10 56.4 o' = E f (x -[x)? / n= 56.4 / 10 = 5.64 0 = V5.64 = 2.37 The standard error of the sample medians is approximately 2.37. CHECK YOUR PROGRESS Random samples with size 4 are drawn from the population containing the values 14, 19, 26, 31, 48, and 53. a.) Construct a sampling distribution of the sample means b.) Find the mean of the sample means. c.) Compute the standard error of the sample means SAMPLING DISTRIBUTION OF THE SAMPLE MEANS The mean and the standard error of the distribution of sample means taken from a population with mean u and standard deviation o can be computed as Hs = H, Os = 0 / vn Example: Random samples with size 4 are drawn from the population containing the values 14, 19, 26, 31, 48, and 53. Find the mean and standard error of the sample means.Solution: The mean of the population is p = 14 +19 + 26 + 31 + 48 + 53 = 191/6 = 31.83 H = = 31.83 To get the standard error, compute first the population standard deviation: 0= (14 - 31 83) 4 (19 -31.83) + (26 -31 83) (31 -3181) + (48 -31 83) + (53 - 31.83) 0 - 14.3 Thus, the standard error of the mean is 0% = 0 / Vn = 14.3 / V4 - 7.15 CHECK YOUR PROGRESS A school has 900 junior high school students. The average height of these students is 68 in with a standard deviation of 6 in. Suppose you draw a random sample of 50 students. Find the mean, standard deviation, and variance of the distribution of all sample means that can be derived from the samples. DISTRIBUTION OF THE SAMPLE MEAN OF A NORMAL VARIABLE 2 = ( X - H ) o / vn THE CENTRAL LIMIT THEOREM Given a random variable x with mean u and variance o', then, regardless whether the population distribution of X Is normally distributed or not, as the sample size n gets larger, the shape of the distribution of the sample means taken from the population approaches a normal distribution, with mean u and standard deviation o / vn. Note: The emphasis of the central limit theorem is on the phrase " as the sample size gets larger". You can only assume the sampling distribution to be normal when the sample is large enough. Mostly, n 2 30 is sufficient to approximate the normality of the sampling distribution of the sample mean. Example: The average age of teachers in a certain town is 34 with a standard deviation of 4. If the principal of ABC College employs 100 teachers, find the probability that the average age of these teachers is less than 35.Solution: It is not given that the population is normally distributed but since n > 30, then you can assume that the sampling distribution of the mean ages of 100 teachers is normal according to Central Limit Theorem. Converting to standard normal score, z= (35 - 34) / ( 4 / V100 ) = 10/4 = 2.5 Then, P ( X