


Population genetics
You are planning to perform a linkage analysis study of osteoporosis and have two phenotypes of interest: (1) clinical diagnosis (i.e., binary trait), and (2) femoral bone mineral density (i.e., a normally-distributed continuous trait). You have collected two- and three-generation families. Which of the following describes an appropriate analysis plan for your study?
Haseman-Elston regression for both traits
non-parametric for clinical diagnosis, and variance components for bone mineral density
parametric for clinical diagnosis and non-parametric for bone mineral density
Haseman-Elston regression for clinical diagnosis and parametric for bone mineral density
score statistics for clinical diagnosis and non-parametric for bone mineral density
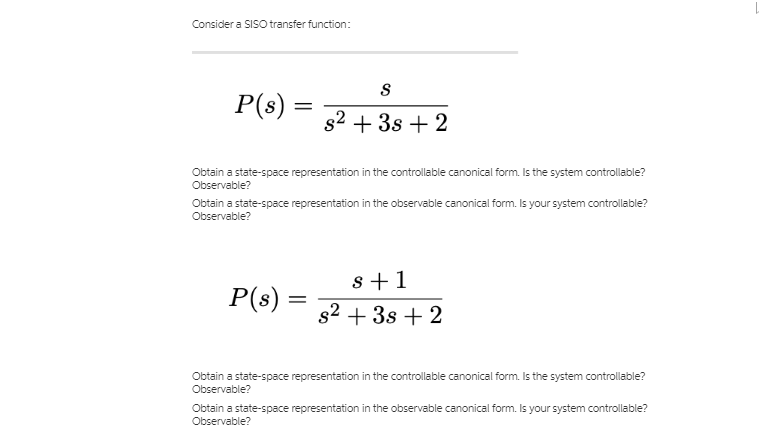
CE2.2a Use the numerical parameters in Table 2.4 for this and all ensu- ing CE2 assignments (see Figure 1.15). Simulate and plot the open-loop state variable responses for three cases (for this problem use the state-space realizations of CE1.2b); assume zero initial state for all cases [except Case i(b) below]: i. Single-input, single-output: input f () and output O(f). (a) unit impulse input f () and zero initial state. (b) zero input f () and an initial condition of e (0) = 0.1 rad (zero initial conditions on all other state variables). ii. Single-input, multiple-output: impulse input f() and two outputs w(t) and e(t). iii. Multiple-input, multiple-output: two unit step inputs f() and r() and two outputs w() and e(1). Simulate long enough to demonstrate the steady-state behavior. What are the system eigenvalues? Based on these eigenvalues and the physical system, explain the system responses. TABLE 2.4 Numerical Parameters for CE2 System Parameter Value Units Name kg cart mass - kg pendulum mass L 0.75 m pendulum length 9.81 m/s2 gravitational accelerationConsider a SISO transfer function: S P(s) = $2 + 3s + 2 Obtain a state-space representation in the controllable canonical form. Is the system controllable? Observable? Obtain a state-space representation in the observable canonical form. Is your system controllable? Observable? s +1 P(s) = $2 + 3s + 2 Obtain a state-space representation in the controllable canonical form. Is the system controllable? Observable? Obtain a state-space representation in the observable canonical form. Is your system controllable? Observable?8-1 Given the following system transfer function Y(s) s + 3 = U(s) $ 2 + 35 + 2 (a) Show its controllability canonical form (b) Show its diagonal controllability canonical form (c) Show its observability canonical formQuestion 1 {1 point) X Saved Normal Distributions are required for: O Parametric Statistics 0 NonParametric Statistics 6) Regression Analysis 0 Parametric Statistics & Regression Analysis 0 All of the other answers What type of modeling is appropriate for each of the following problems. 1. A medical student is investigating if the dosage of a drug irrflue nces the time it takes for a person to be treated. 2. A medical student is investigating if the dosage of a drug influences the probability of a patient being treated or not being treated. A. Parametric for l and non-parametric for 2 B. Logistic Regression for 1 and Least 3g uare Regression for 2 C. Least Square Regression for 1 and Logistic Regression forZ D. Nonparametric for 1 and parametric for 2















