Practical Application Scenario 1

Refer to the stock you selected in Module 1. Using the same downloaded data, build a 95 percent confidence interval for the daily stock volume using your downloaded data. What does this confidence interval mean?

Why might decision makers be interested in such an interval?

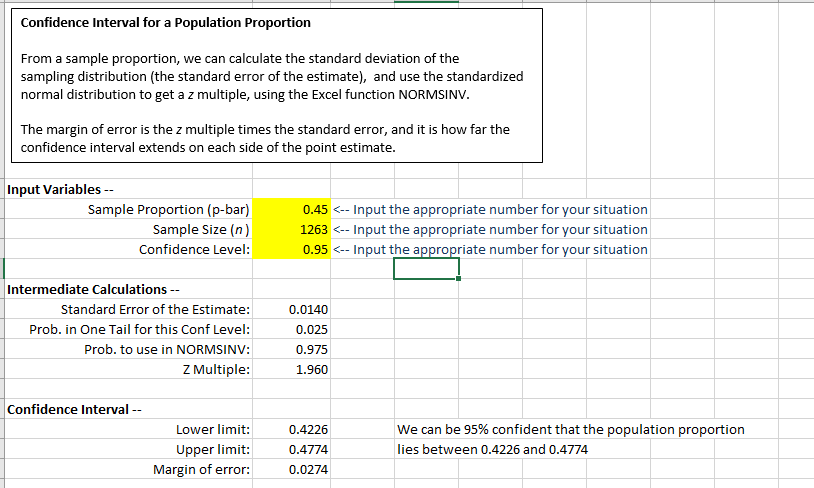
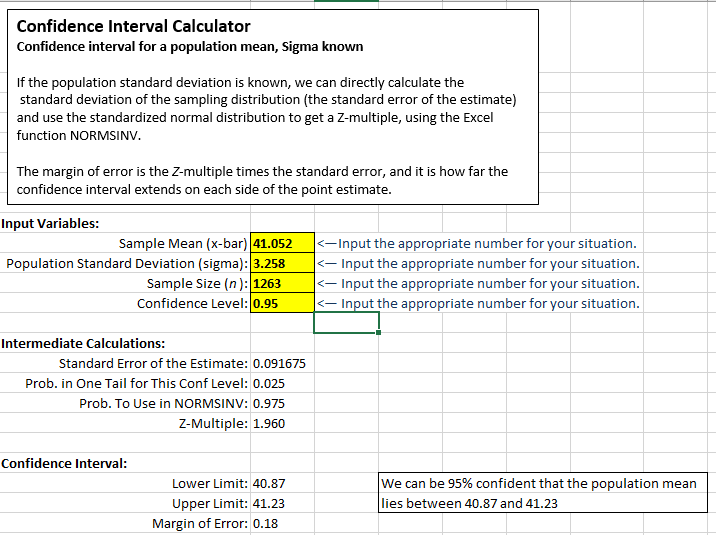
Confidence Interval for a Population Proportion From a sample proportion, we can calculate the standard deviation of the sampling distribution (the standard error of the estimate and use the standardized normal distribution to get a z multiple, using the Excel function NORMSINV The margin of error is the z multiple times the standard error, and it is how far the confidence interval extends on each side of the point estimate Input Variables Sample Proportion (p-bar) 0.45 nput the appropriate number for your situation Sample size (n) nput the appropriate number for your situation 1263 Confidence Level: 0.95 nput the a ropriate number for your situation Intermediate Calculations Standard Error of the Estimate: 0.0140 Prob. in One Tail for this Conf Level: 0.025 Prob. to use in NORMSINV: 0.975 Multiple 1.960 Confidence Interval Lower limit: We can be 95% confident that the population proportion 0.4226 Upper limit lies between 0.4226 and 0.4774 0.4774 Margin of error: 0.0274 Confidence Interval for a Population Proportion From a sample proportion, we can calculate the standard deviation of the sampling distribution (the standard error of the estimate and use the standardized normal distribution to get a z multiple, using the Excel function NORMSINV The margin of error is the z multiple times the standard error, and it is how far the confidence interval extends on each side of the point estimate Input Variables Sample Proportion (p-bar) 0.45 nput the appropriate number for your situation Sample size (n) nput the appropriate number for your situation 1263 Confidence Level: 0.95 nput the a ropriate number for your situation Intermediate Calculations Standard Error of the Estimate: 0.0140 Prob. in One Tail for this Conf Level: 0.025 Prob. to use in NORMSINV: 0.975 Multiple 1.960 Confidence Interval Lower limit: We can be 95% confident that the population proportion 0.4226 Upper limit lies between 0.4226 and 0.4774 0.4774 Margin of error: 0.0274