Present value of a coupon bond: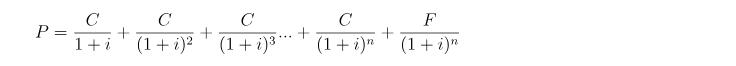 

The coupon rate is 5 percent, the coupon is paid annually, and the maturity is 5 years. The face value is 100, and investor holds the investment until the maturity. The market rate (discounting factor) is 2.5 percent. Taking this all together, we get that the present value of the bond at the issue date is 111.6 euros.

(a) The markets anticipate an increase in the default risk of the government. Since the expected default risk of T-bond increased, the market value of recently issued 5-year bonds decreased from 100 to 98. What is the change in the bond’s yield to maturity? Draw equations and explain answer as clear as possible?
(b) The government is broke, and it unilaterally declares a debt moratorium at period (i.e., year) 4. Therefore, it cancels the coupon payment at period 4 and postpones paying the last coupon and returning the face value by one year. If this had been known when the debt was issued, how much would it have affected the present value of the bond? Calculate and explain answer as clearly as possible?
 

Transcribed Image Text:

P = C C + 1+i (1 + i)² + C (1 + i)³ + C (1+i)n + F (1+i)n P = C C + 1+i (1 + i)² + C (1 + i)³ + C (1+i)n + F (1+i)n

Answer rating: 100% (QA)

Answer a To calculate the change in the bonds yield to maturity we need to determine the new price of the bond after the decrease in market value Lets denote the new price as P Given Coupon rate C 5 0... View the full answer