

Probability and statistics quiz.
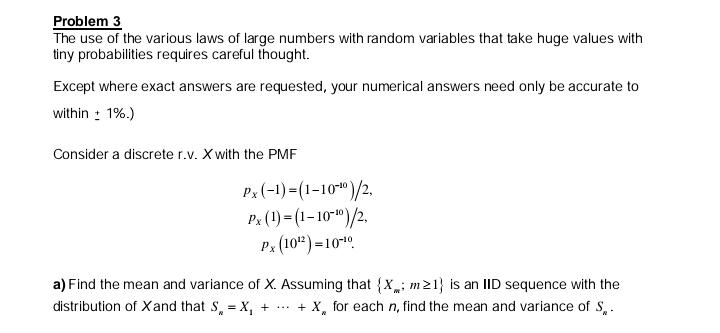
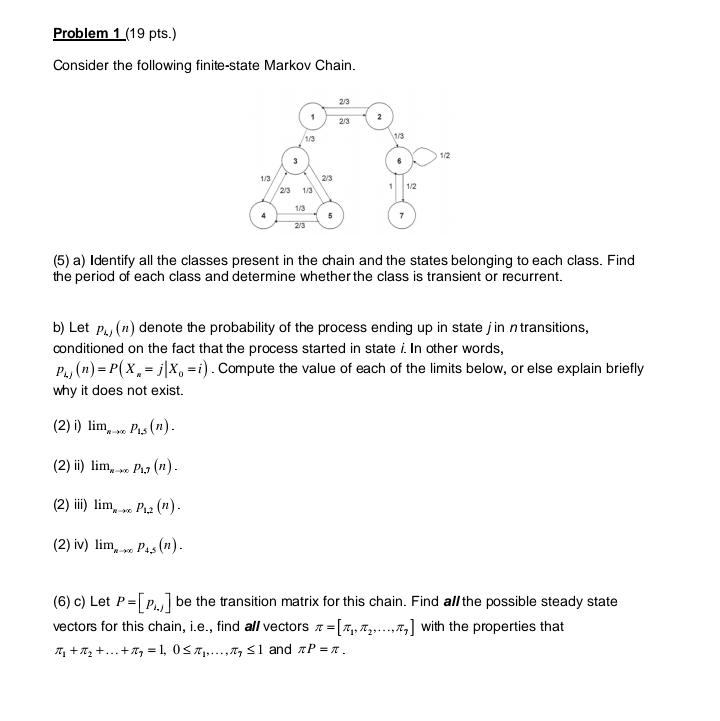
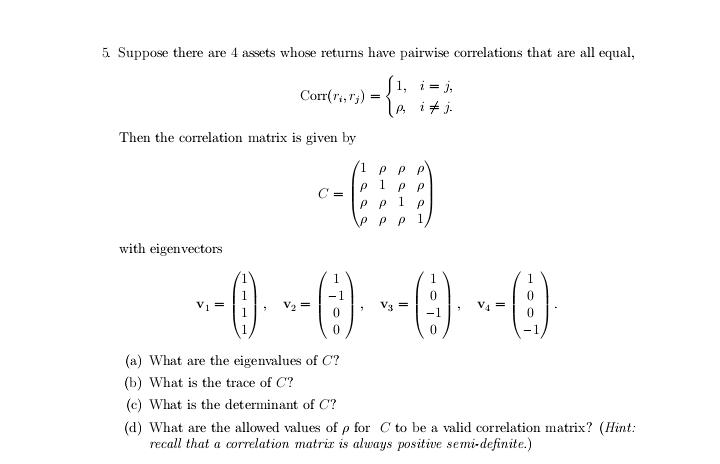
Problem 3 The use of the various laws of large numbers with random variables that take huge values with tiny probabilities requires careful thought. Except where exact answers are requested, your numerical answers need only be accurate to within : 1%.) Consider a discrete r.v. X with the PMF Px (-1)=(1-10-10)/2. Px (1) = (1-10-10)/2. px (10") =10-10 a) Find the mean and variance of X. Assuming that { X : m 21; is an IID sequence with the distribution of Xand that S.=X, + ... + X for each n, find the mean and variance of S..Problem 1 (19 pts.) Consider the following finite-state Markov Chain. (5) a) Identify all the classes present in the chain and the states belonging to each class. Find the period of each class and determine whether the class is transient or recurrent. b) Let pay (n) denote the probability of the process ending up in state / in n transitions, conditioned on the fact that the process started in state i. In other words, Py (n)= P(X. = j|X, =i) . Compute the value of each of the limits below, or else explain briefly why it does not exist. (2) i) lim, Pis (n). (2) ii) lim, Me Pix (n). (2) iii) lim, PLz (n). (2) iv) lim, " PAS (n). (6) c) Let P=[p,] be the transition matrix for this chain. Find all the possible steady state vectors for this chain, i.e., find all vectors * = [x,, ,.....; ] with the properties that Mthy+... +my = 1, 05 x,......; El and AP=1.1. [Geometric Random Variables] During a bad economy, a graduating ECE student goes to career fair booths in the technology sector (e.g., Google, Apple, Qualcomm, Texas Instruments, Motorola, etc) - and his/her likelihood of receiving an off-campus interview invitation after a career fair booth visit depends on how well he/she did in ECE 313. Specifically, an A in 313 results in a probability p = 0.95 of obtaining an invitation, whereas a C in 313 results in a probability of p = 0.15 of an invitation. (a) Give the pmf for the random variable Y that denotes the number of career fair booth visits a student must make before his/her first invitation including the visit that results in the invitation. Express your answer in terms of p. Solution: py(k) = p(1 - p)*~' for k 2 1. (b) On average, how many booth visits must an A student make before getting an off-campus interview invitation? How about a C student?5. Suppose there are 4 assets whose returns have pairwise correlations that are all equal, Com( n,r;) = 1, i= i ( p, if j. Then the correlation matrix is given by p p C= 1 p p p p 1 p p with eigenvectors - 1 OOH . = (a) What are the eigenvalues of C? (b) What is the trace of C? (c) What is the determinant of C? (d) What are the allowed values of p for C to be a valid correlation matrix? (Hint: recall that a correlation matrix is always positive semi-definite.)Problems Involving Two Genes 1 . A man with dark (dominant), curly (see problem I.5.) hair marries a woman with light, straight hair. Their daughter, who happens to have dark hair, marries a man with light, wavy hair. Answer the following questions about this dark-haired daughter and her family. a. Draw a Punnett's square for this marriage, and predict the phenotypic ratio among the offspring of the daughter and her husband. b. What is the chance that they will have a child with hair just like his or her father's? 2. In cats, again, black color is dominant to a special, temperature-sensitive albino gene which produces cats with dark legs, faces and tails (Siamese cats, in case you don't recognize it). A short haired (dominant) Siamese colored female is bred to a long-haired black male. They have eight kittens: 2 black, short-haired; 2 black, long-haired; 2 Siamese, short-haired; and 2 Siamese, long-haired. What were the genotypes of the two parents? 3. Elizabeth is married to John, and they have four children. Elizabeth has a straight nose (recessive) and is able to roll her tongue ( dominant). John is also able to roll his tongue, but he has a convex (Roman) nose (dominant). Of their four children, Ellen is just like her father, and Dan is just like his mother. The other children-Anne, who has a convex nose, and Peter, who has a straight nose-are unable to roll their tongues. Please answer the following questions about this family. a. What are the genotypes of Elizabeth and John? b. Elizabeth's father was a straight-nosed roller, while her mother was a convex-nosed non-roller. What can you figure out about their genotypes? c. John's father was a straight-nosed roller, while his mother was a convex-nosed roller. What can you determine about their genotypes? d. Diagram the three described generations of this family in accepted pedigree form, including the phenotypes for these two traits. 4. If a pure-breeding (homozygous) black (dominant), long-haired (recessive) cat is mated to a pure- breeding Siamese, short-haired cat, and one of their male offspring is mated to one of their female offspring, what is the chance of producing a Siamese colored, short-haired kitten? 5 In horses, one which runs best in water (or in wet conditions) is called (WATER), and one which runs best in dry conditions is called (DRY). (WATER) is recessive to (DRY). A horse can also be either a trotter, which we will designate (GAIT) or a pacer, which we will designate (PACE). (PACE) is recessive to (GATE). We have mated two horses, a stallion named Halter-Man and a mare named Erlich-Mane. Halter-Man is a (WATER)(PACER), while Erlich-mane is a (DRY)(GAIT). One of Erlich-Mane's parents was a (WATER)(PACER). What are the chances of Erlich-Mane and Halter-Man producing a (WATER) (GAIT) foal (that's a baby horse, in case you didn't know)? 6. When a male pig from a line of true-breeding (homozygous) black, solid-hooved pigs was crossed to a female from a breed (homozygous) of red, cloven-hooved pigs, their several progeny all looked alike with regard to color and hooves. These progeny were all mated to members of the same breed as their red, cloven-hooved mother pig. The offspring from this final cross were: 1 1 black, cloven-hooved; 8 black, solid-hooved; 14 red, cloven-hooved; and 10 red, solid-hooved. For each of these two genes (coat color and hoof type) determine which allele is the dominant one. Explain your reasoning. What were the phenotypes of the progeny produced by the first mating in this problem? 7. In garden peas, long stems are dominant to short stems, and yellow seeds are dominant to green seeds. 100 long/yellow pea plants, all of which had one short/green parent, are interbred (bred to each other). 1600 progeny result. Please answer the following questions about these progeny. a. Assuming that these two genes are unlinked, about how many long/green pea plants would you expect to find among the offspring? b. What ratio of yellow to green seed color would you expect among the offspring? c. What would you expect the overall phenotypic ratio among the 1600 offspring to be (taking into consideration both traits)
















