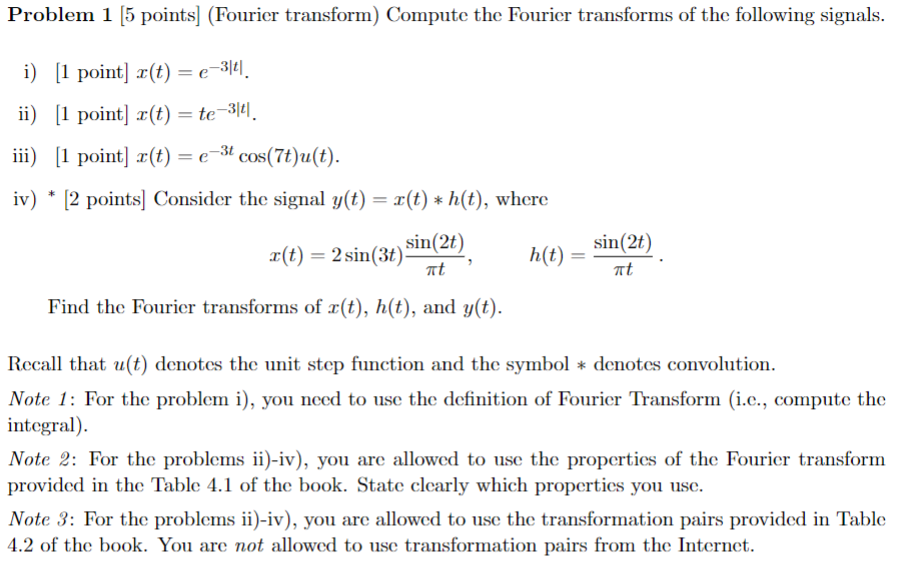
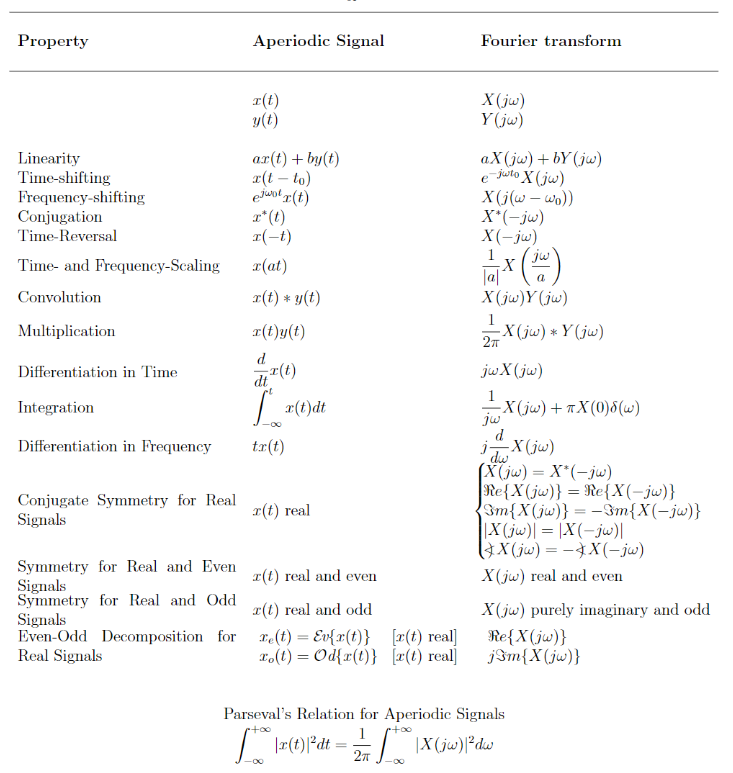
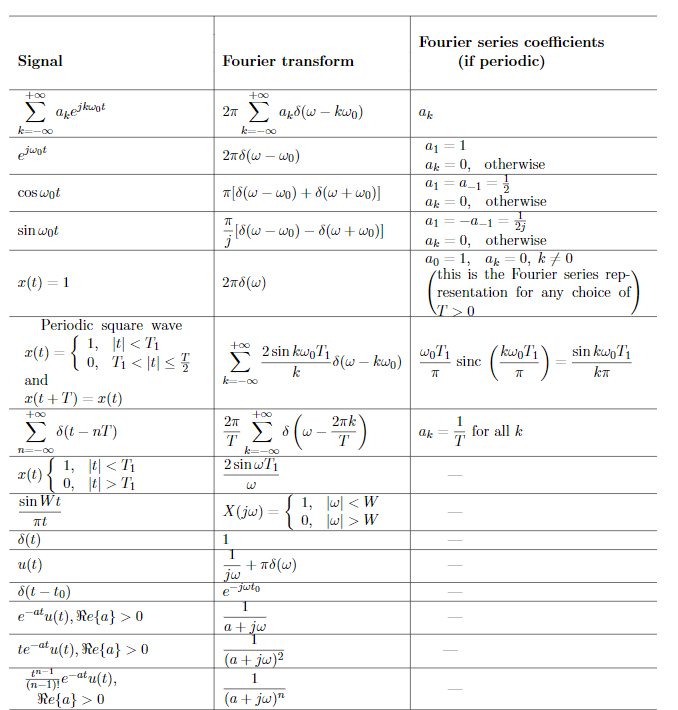
Problem 1 [5 points] (Fourier transform) Compute the Fourier transforms of the following signals. i) [1 point] x(t) = e3|t|. ii) [1 point] x(t) = te3|t|. iii) [1 point] x(t) = e3 cos(7t)u(t). iv) [2 points] Consider the signal y(t) = x(t) * h(t), where sin(2t) == x(t) = 2sin(3t) t sin(2t) h(t) = t Find the Fourier transforms of x(t), h(t), and y(t). Recall that u(t) denotes the unit step function and the symbol * denotes convolution. Note 1: For the problem i), you need to use the definition of Fourier Transform (i.e., compute the integral). Note 2: For the problems ii)-iv), you are allowed to use the properties of the Fourier transform provided in the Table 4.1 of the book. State clearly which properties you use. Note 3: For the problems ii)-iv), you are allowed to use the transformation pairs provided in Table 4.2 of the book. You are not allowed to use transformation pairs from the Internet. Property Aperiodic Signal Fourier transform x(t) y(t) Linearity Time-shifting Frequency-shifting ax(t) + by(t) x(t - to) X(jw) Y(jw) ax (jw) + by (jw) e-juto X (jw) ejwot x(t) X(j(w-wo)) Conjugation x*(t) Time-Reversal x(-t) Time- and Frequency-Scaling x(at) |a| Convolution x(t) * y(t) Multiplication x(t)y(t) d Differentiation in Time dt x(t) Integration Lx(t)dt Differentiation in Frequency tx(t) Conjugate Symmetry for Real Signals x(t) real (FX (jw) = Symmetry for Real and Even Signals x(t) real and even X*(-jw) X(-jw) X jw a X(jw)Y (jw) 2T -X (jw) *Y (jw) jwX(jw) +X (jw) + xX (0)5(w) jw d iX (jw) dw (X(jw) = x*(-jw) Re{X(jw)} = Re{X(-jw)} Im{X (jw)}=-Im{X(jw)} ||X(jw)| = |X(jw)|| X(jw) real and even X(-jw) Symmetry for Real and Odd x(t) real and odd Signals X(jw) purely imaginary and odd Even-Odd Decomposition for re(t) = Ev{x(t)} Real Signals [x(t) real] x(t) = Od{x(t)} [x(t) real] Re{X(jw)} jIm{X(jw)} Parseval's Relation for Aperiodic Signals +oo 1 |x(t)|dt = [|X (jw)dw 2T - Signal Fourier transform Fourier series coefficients (if periodic) +oo k=- @jwot cos wot +oo 2 - ) (wkwo) k=- 2d(w-wo) sin wot x(t)=1 Periodic square wave 1, |t|Ti }}=(1) x(t) = { 0, 0 te-atu(t), Re{a} > 0 th-T (n-1)e-atu(t), Re{a} > 0 a+jw (a+jw) 1 (a + jw)n