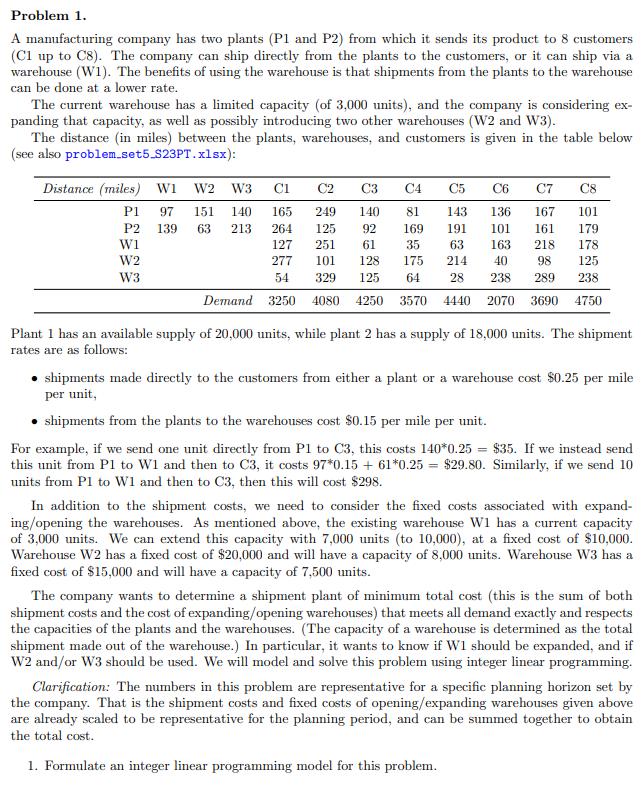
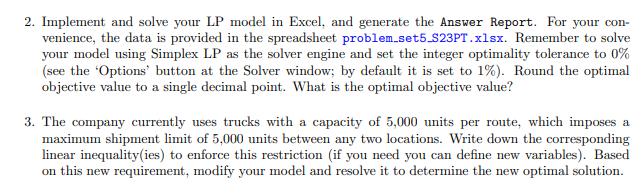
Problem 1. A manufacturing company has two plants (P1 and P2) from which it sends its product to 8 customers (C1 up to C8). The company can ship directly from the plants to the customers, or it can ship via a warehouse (W1). The benefits of using the warehouse is that shipments from the plants to the warehouse can be done at a lower rate. The current warehouse has a limited capacity (of 3,000 units), and the company is considering ex- panding that capacity, as well as possibly introducing two other warehouses (W2 and W3). The distance (in miles) between the plants, warehouses, and customers is given in the table below (see also problem.set5_S23PT.xlsx): Distance (miles) W1 W2 W3 C1 C2 C3 C4 C5 C6 C7 C8 P2 P1 97 151 140 139 165 249 140 81 143 136 167 101 63 213 264 125 92 169 191 101 161 179 W1 127 251 61 35 63 163 218 178 W2 W3 277 101 128 175 214 40 98 125 54 329 125 64 28 238 289 238 Demand 3250 4080 4250 3570 4440 2070 3690 4750 Plant 1 has an available supply of 20,000 units, while plant 2 has a supply of 18,000 units. The shipment rates are as follows: shipments made directly to the customers from either a plant or a warehouse cost $0.25 per mile per unit, shipments from the plants to the warehouses cost $0.15 per mile per unit. $35. If we instead send $29.80. Similarly, if we send 10 For example, if we send one unit directly from P1 to C3, this costs 140*0.25 this unit from P1 to W1 and then to C3, it costs 97*0.15 + 61*0.25 units from Pl to W1 and then to C3, then this will cost $298. In addition to the shipment costs, we need to consider the fixed costs associated with expand- ing/opening the warehouses. As mentioned above, the existing warehouse W1 has a current capacity of 3,000 units. We can extend this capacity with 7,000 units (to 10,000), at a fixed cost of $10,000. Warehouse W2 has a fixed cost of $20,000 and will have a capacity of 8,000 units. Warehouse W3 has a fixed cost of $15,000 and will have a capacity of 7,500 units. The company wants to determine a shipment plant of minimum total cost (this is the sum of both shipment costs and the cost of expanding/opening warehouses) that meets all demand exactly and respects the capacities of the plants and the warehouses. (The capacity of a warehouse is determined as the total shipment made out of the warehouse.) In particular, it wants to know if W1 should be expanded, and if W2 and/or W3 should be used. We will model and solve this problem using integer linear programming. Clarification: The numbers in this problem are representative for a specific planning horizon set by the company. That is the shipment costs and fixed costs of opening/expanding warehouses given above are already scaled to be representative for the planning period, and can be summed together to obtain the total cost. 1. Formulate an integer linear programming model for this problem. 2. Implement and solve your LP model in Excel, and generate the Answer Report. For your con- venience, the data is provided in the spreadsheet problem.set5_S23PT.xlsx. Remember to solve your model using Simplex LP as the solver engine and set the integer optimality tolerance to 0% (see the 'Options' button at the Solver window; by default it is set to 1%). Round the optimal objective value to a single decimal point. What is the optimal objective value? 3. The company currently uses trucks with a capacity of 5,000 units per route, which imposes a maximum shipment limit of 5,000 units between any two locations. Write down the corresponding linear inequality(ies) to enforce this restriction (if you need you can define new variables). Based on this new requirement, modify your model and resolve it to determine the new optimal solution.