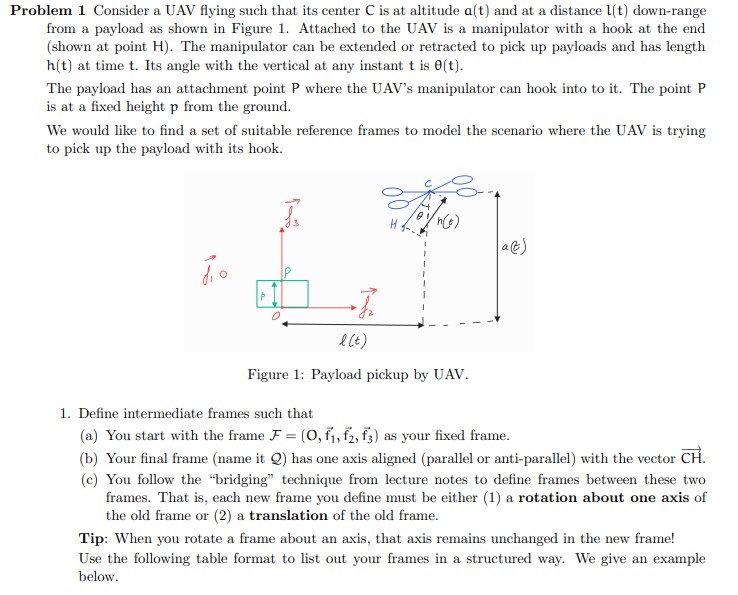
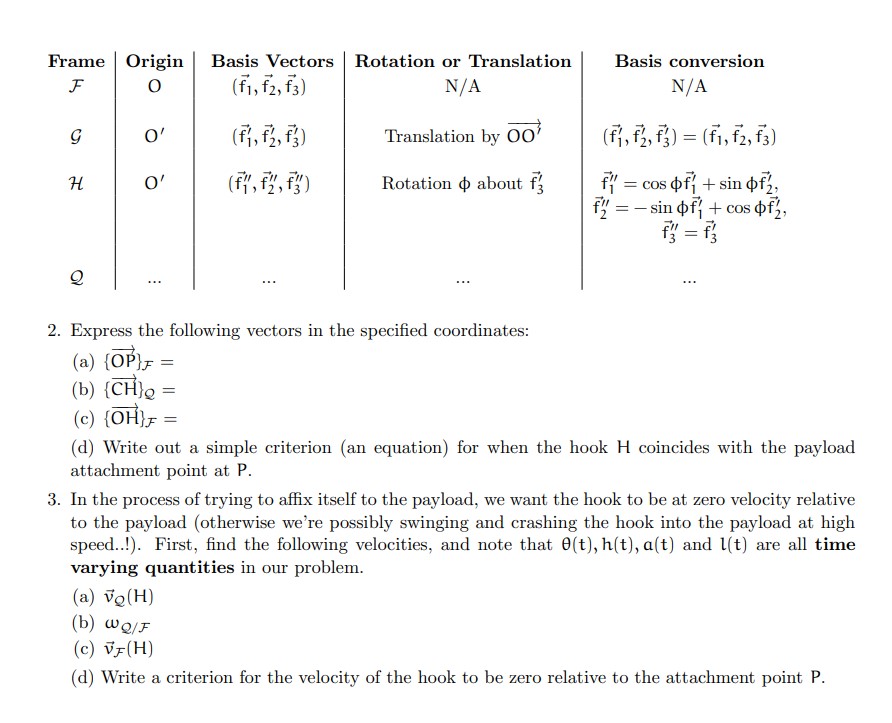
Problem 1 Consider a UAV flying such that its center C is at altitude a(t) and at a distance 1(t) down-range from a payload as shown in Figure 1. Attached to the UAV is a manipulator with a hook at the end (shown at point H). The manipulator can be extended or retracted to pick up payloads and has length h(t) at time t. Its angle with the vertical at any instant t is e(t). The payload has an attachment point P where the UAV's manipulator can hook into to it. The point P is at a fixed height p from the ground. We would like to find a set of suitable reference frames to model the scenario where the UAV is trying to pick up the payload with its hook. a) 1(t) Figure 1: Payload pickup by UAV. 1. Define intermediate frames such that (a) You start with the frame F = (0, 1, 2, f3) as your fixed frame. (b) Your final frame (name it Q) has one axis aligned (parallel or anti-parallel) with the vector CH. (c) You follow the "bridging" technique from lecture notes to define frames between these two frames. That is, each new frame you define must be either (1) a rotation about one axis of the old frame or (2) a translation of the old frame. Tip: When you rotate a frame about an axis, that axis remains unchanged in the new frame! Use the following table format to list out your frames in a structured way. We give an example below. Frame Origin Basis Vectors | Rotation or Translation F 0 (F1, F2, f3) N/A Basis conversion N/A G O' (F1, F2, f) Translation by 00' (fi, f, f) = (f1, f2, f3) H O' (f", f, f) Rotation about f F = cos of + sin of, fsin of+cos of, f = f 2 2. Express the following vectors in the specified coordinates: (a) {OP} = (b) {CH}= (c) {OH} = (d) Write out a simple criterion (an equation) for when the hook H coincides with the payload attachment point at P. 3. In the process of trying to affix itself to the payload, we want the hook to be at zero velocity relative to the payload (otherwise we're possibly swinging and crashing the hook into the payload at high speed..!). First, find the following velocities, and note that 0(t), h(t), a(t) and 1(t) are all time varying quantities in our problem. (a) vo(H) (b) wQ/F (c) VF(H) (d) Write a criterion for the velocity of the hook to be zero relative to the attachment point P.