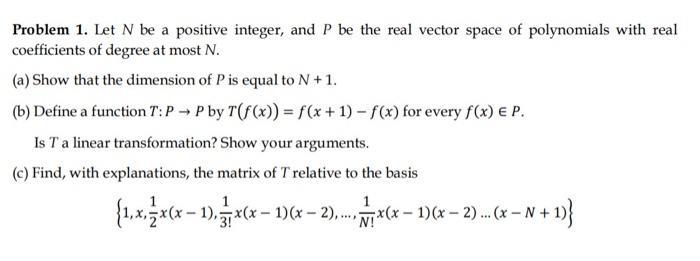
Problem 1. Let N be a positive integer, and P be the real vector space of polynomials with real coefficients of degree at most N. (a) Show that the dimension of P is equal to N+1. (b) Define a function T:P - P by T(f(x)) = f(x+ 1) - f(x) for every f(x) P. Is Ta linear transformation? Show your arguments. (c) Find, with explanations, the matrix of T relative to the basis {1,xx(x - 1),(x - 1)(* - 2).(x - 1)(x - 2).( -N + 1)}