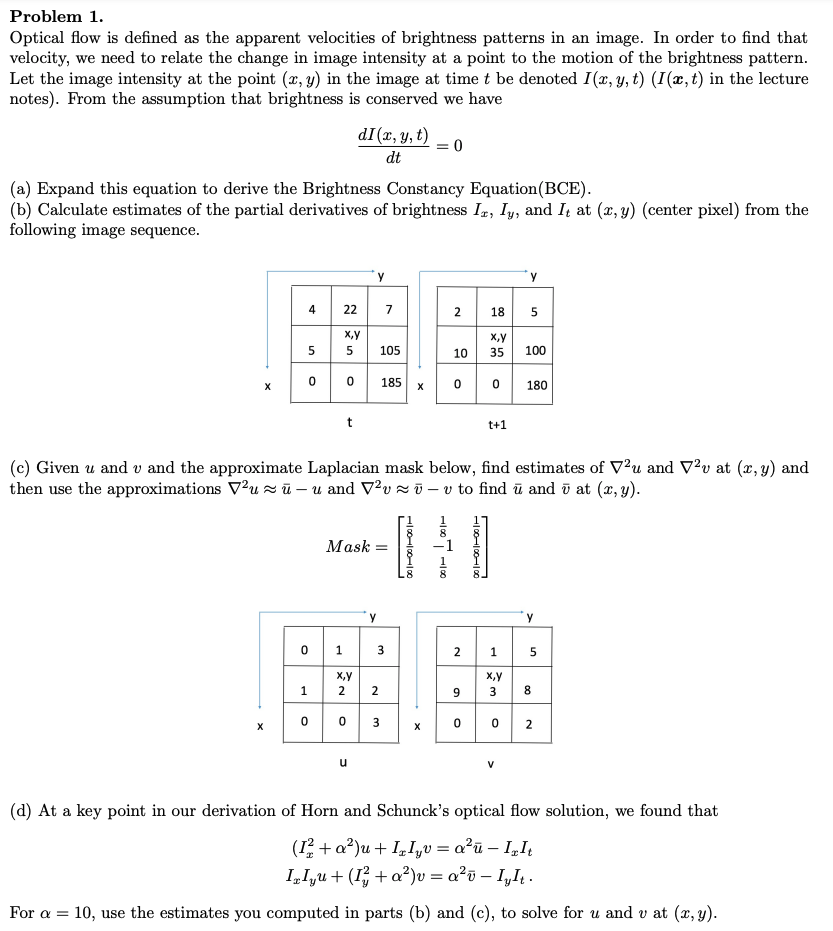
Problem 1. Optical flow is defined as the apparent velocities of brightness patterns in an image. In order to find that velocity, we need to relate the change in image intensity at a point to the motion of the brightness pattern. Let the image intensity at the point (x, y) in the image at time t be denoted I(x, y, t) (I(x, t) in the lecture notes). From the assumption that brightness is conserved we have dI(x, y, t) dt = 0 (a) Expand this equation to derive the Brightness Constancy Equation (BCE). (b) Calculate estimates of the partial derivatives of brightness Ir, Iy, and It at (x, y) (center pixel) from the following image sequence. y 18 5 x,y 35 100 y 4 5 225 22 7 2 , 105 10 10 0 0 185 x x X t 0 0 180 t+1 (c) Given u and v and the approximate Laplacian mask below, find estimates of V2u and V2v at (x, y) and then use the approximations V2uuu and Vv v to find and at (x, y). Mask = 100-100-100 -100-100 -100-100-100 y 0 1 3 x,y y 2 1 5 , 1 2 2 9 3 8 0 X 0 3 0 0 2 x u V (d) At a key point in our derivation of Horn and Schunck's optical flow solution, we found that (I + a)u + IIv = a IIt IxIyu+ (I + a)v = v IyIt . For a 10, use the estimates you computed in parts (b) and (c), to solve for u and vat (x, y).