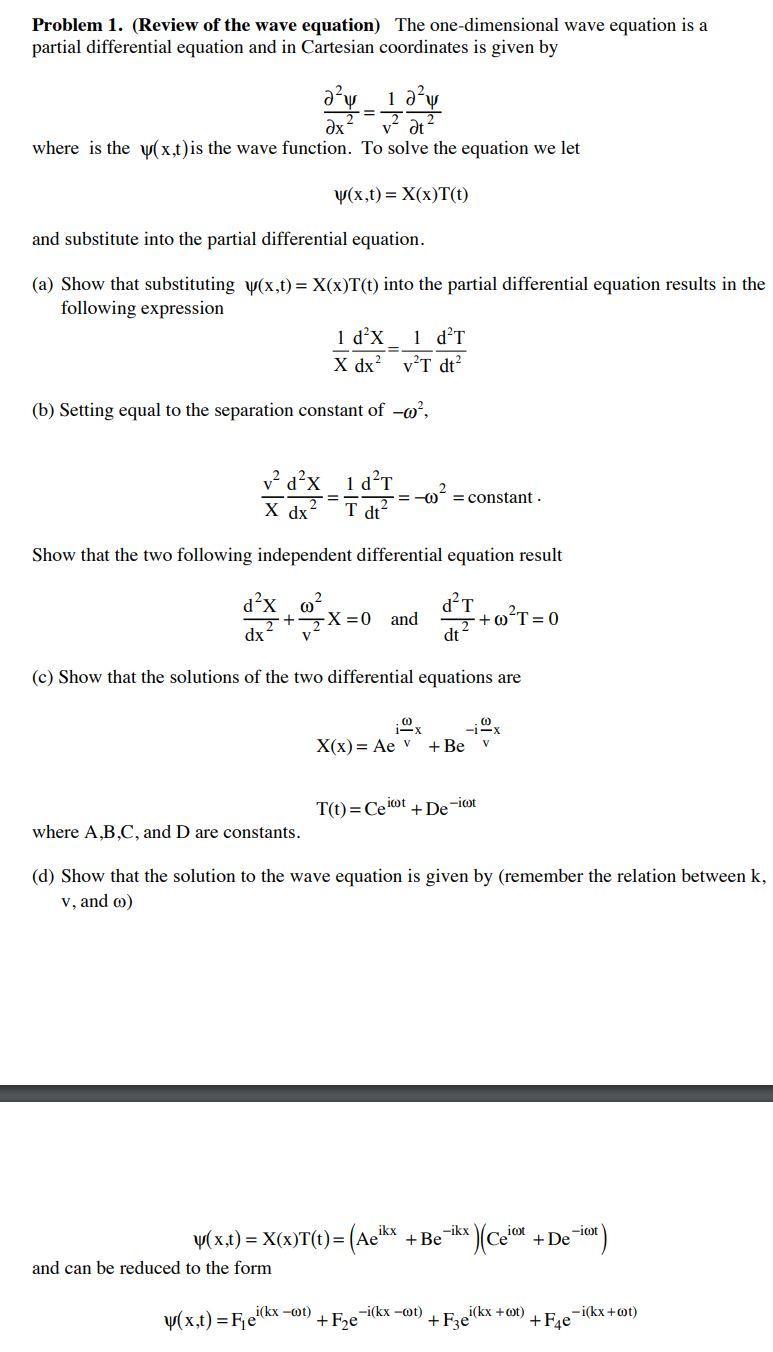
Problem 1. (Review of the wave equation) The one-dimensional wave equation is a partial differential equation and in Cartesian coordinates is given by = x v dt where is the y(x,t) is the wave function. To solve the equation we let y(x,t)= X(x)T(t) and substitute into the partial differential equation. (a) Show that substituting y(x,t)= X(x)T(t) into the partial differential equation results in the following expression 1 dX_ 1 dT = X dx vT dt (b) Setting equal to the separation constant of -, 1 2y vdx 1dT X dx T dt = Show that the two following independent differential equation result dx @. dx dT dt (c) Show that the solutions of the two differential equations are and can be reduced to the form y(x,t)=Fe 2+2X=0 -X = 0 and V i(kx -o0ot) ==constant. 2 (0) i-x -X X(x) = Ae v + Fe where A,B,C, and D are constants. (d) Show that the solution to the wave equation is given by (remember the relation between k, v, and co) 2+0T=0 2T=0 y(x,t) = X(x)T(t)= (Aeikx +Be-ikx (Ce + De 1001 -icot -i(kx-wt) + Be T(t)=Ce it +De-icot (10) -i-x V _i(kx + (ot) +F3e +F4e-i(kx+cot)