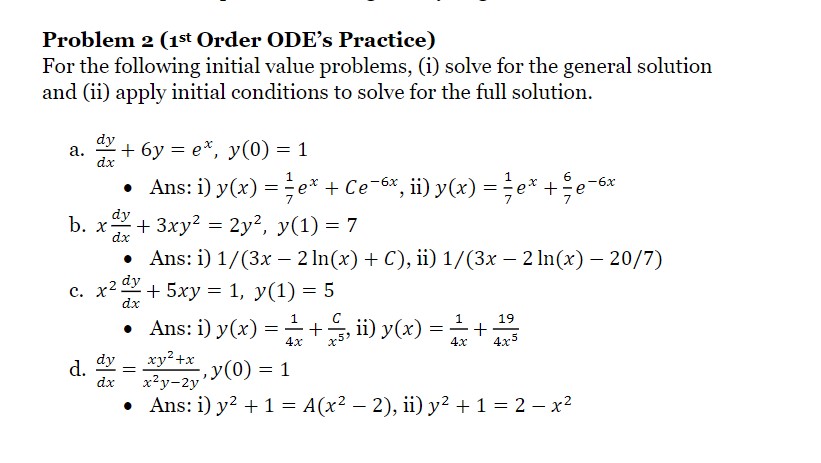
Problem 2 (1st Order ODE's Practice) For the following initial value problems, (i) solve for the general solution and (ii) apply initial conditions to solve for the full solution. +6y=ex, y(0) = 1 dy a. dx Ans: i) y(x) = px 7 b. x dy dx dy c. x2d d. dy dx dx + Cex, ii) y(x) = e* + e + 3xy2 = 2y2, y(1) = 7 -6x Ans: i) 1/(3x - 2 ln(x) + C), ii) 1/(3x - 2 ln(x) - 20/7) = +5xy 1, y(1) = 5 Ans: i) y(x) = +, ii) y(x) : = xy+x x2y-2y1 , y(0) = 1 4x - = =1+ 19 4x 4x5 Ans: i) y+1 = A(x 2), ii) y + 1 = 2 x