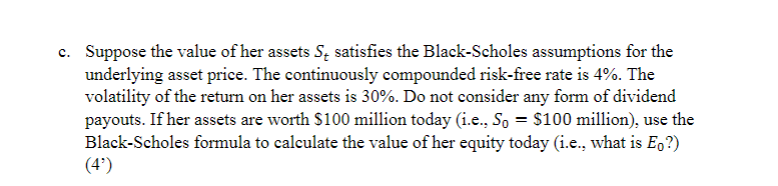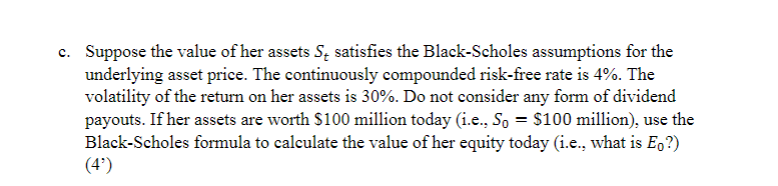
Problem 2. (Equity and debt as options, 15 ) An entrepreneur finances her project with equity (that is owned by the entrepreneur) and debt (that matures in three years). She is supposed to repay $80 million for her debt in three years. If the total assets of her project turn out to be valued above $80 million three years from now, then she will liquidate her assets, repay $80 million in full, and retains the rest as her equity. However, if her total assets turn out to be valued lower than $80 million three years from now, then due to limited liability, she does not need to repay $80 million in full. In that case, she will liquidate everything she has, pay off the creditor with whatever her assets are worth (which is less than $80 million), and retain nothing in three years. Let the value of the total assets at time t be denoted as St, the equity value at time t as Et, and the debt value at time t as Dt. Let today be time t=0, and three years from now be time t=T=3. a. Draw the plot of ET against ST, and the plot of DT against ST, respectively. (Recall that T is equal to 3 , the date of maturity of the debt) (8) b. Economists say that, with limited liability, corporate equity has features of a long call option on corporate assets, and corporate debt has features of a short put on corporate assets and a long bond. Briefly explain why that makes sense with your results from part a. (3') c. Suppose the value of her assets St satisfies the Black-Scholes assumptions for the underlying asset price. The continuously compounded risk-free rate is 4%. The volatility of the return on her assets is 30%. Do not consider any form of dividend payouts. If her assets are worth $100 million today (i.e., S0=$100 million), use the Black-Scholes formula to calculate the value of her equity today (i.e., what is E0 ?) (4) Problem 2. (Equity and debt as options, 15 ) An entrepreneur finances her project with equity (that is owned by the entrepreneur) and debt (that matures in three years). She is supposed to repay $80 million for her debt in three years. If the total assets of her project turn out to be valued above $80 million three years from now, then she will liquidate her assets, repay $80 million in full, and retains the rest as her equity. However, if her total assets turn out to be valued lower than $80 million three years from now, then due to limited liability, she does not need to repay $80 million in full. In that case, she will liquidate everything she has, pay off the creditor with whatever her assets are worth (which is less than $80 million), and retain nothing in three years. Let the value of the total assets at time t be denoted as St, the equity value at time t as Et, and the debt value at time t as Dt. Let today be time t=0, and three years from now be time t=T=3. a. Draw the plot of ET against ST, and the plot of DT against ST, respectively. (Recall that T is equal to 3 , the date of maturity of the debt) (8) b. Economists say that, with limited liability, corporate equity has features of a long call option on corporate assets, and corporate debt has features of a short put on corporate assets and a long bond. Briefly explain why that makes sense with your results from part a. (3') c. Suppose the value of her assets St satisfies the Black-Scholes assumptions for the underlying asset price. The continuously compounded risk-free rate is 4%. The volatility of the return on her assets is 30%. Do not consider any form of dividend payouts. If her assets are worth $100 million today (i.e., S0=$100 million), use the Black-Scholes formula to calculate the value of her equity today (i.e., what is E0 ?) (4)