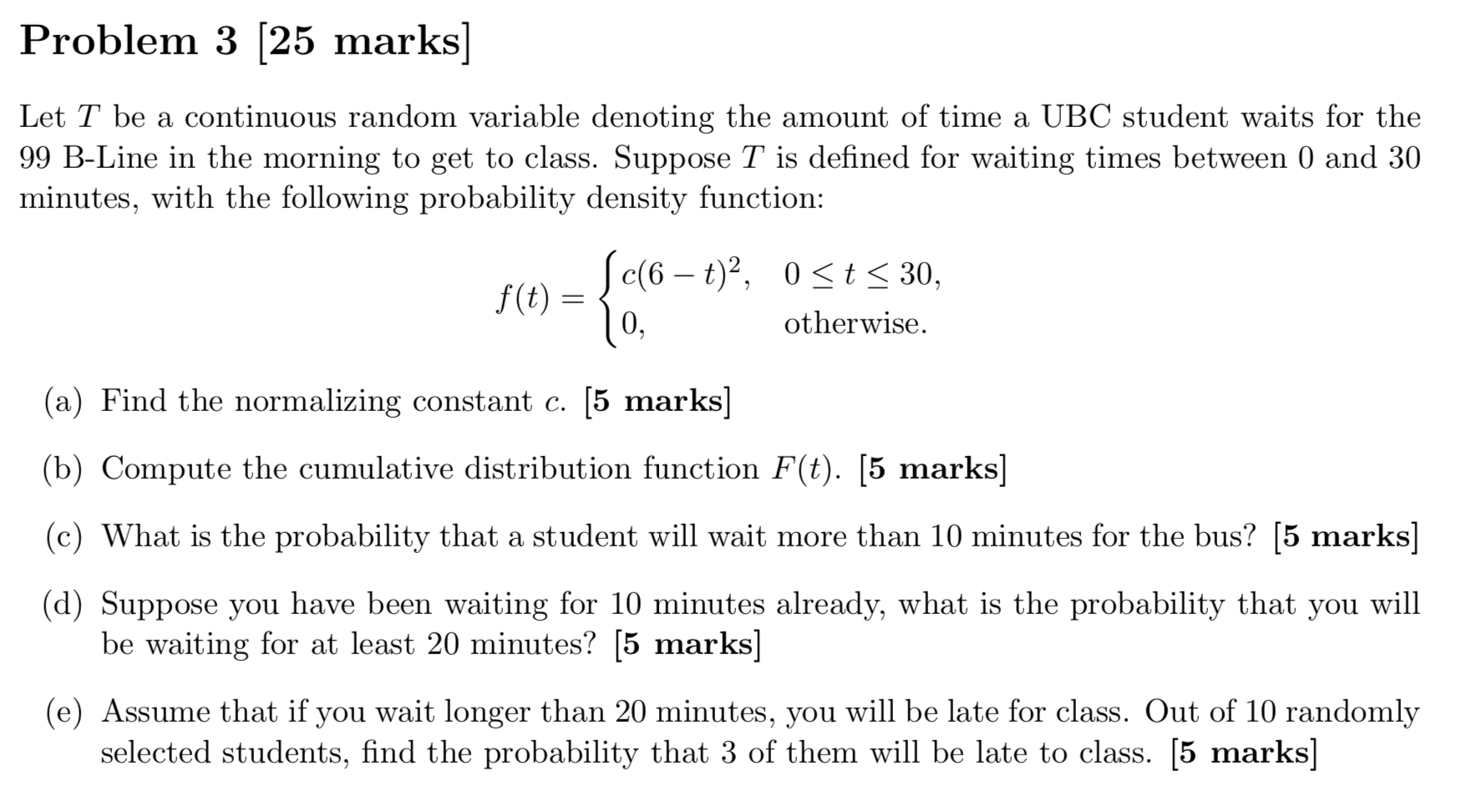
Problem 3 [25 marks]

Let T be a continuous random variable denoting the amount of time a UBC student waits for the 99 B-Line in the morning to get to class. Suppose T is defined for waiting times between 0 and 30 minutes, with the following probability density function:

?c(6?t)2, 0?t?30,

f(t) = 0, otherwise.

(a) Find the normalizing constant c. [5 marks]

(b) Compute the cumulative distribution function F (t). [5 marks]

(c) What is the probability that a student will wait more than 10 minutes for the bus? [5 marks]

(d) Suppose you have been waiting for 10 minutes already, what is the probability that you will

be waiting for at least 20 minutes? [5 marks]

(e) Assume that if you wait longer than 20 minutes, you will be late for class. Out of 10 randomly

selected students, find the probability that 3 of them will be late to class. [5 marks]

Problem 3 [25 marks Let T be a continuous random variable denoting the amount of time a UBC student waits for the 99 B-Line in the morning to get to class. Suppose T is defined for waiting times between 0 and 30 minutes, with the following probability density function: f ( t ) = c(6 - t) 2, 0