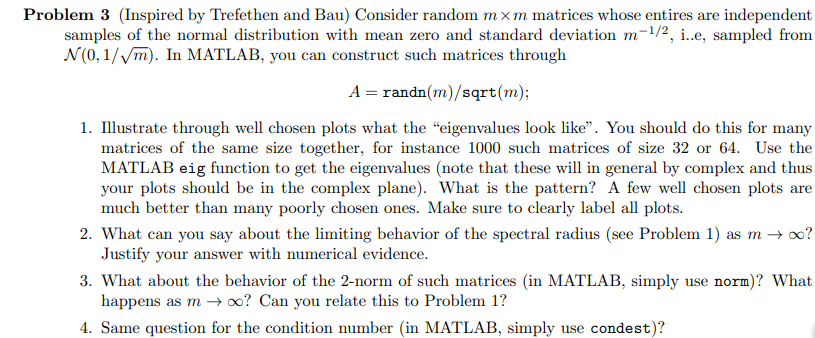
Problem 3 (Inspired by Trefethen and Bau) Consider random mxm matrices whose entires are independent samples of the normal distribution with mean zero and standard deviation m-1/2, i..e, sampled from N(0,1/m). In MATLAB, you can construct such matrices through A = randn(m)/sqrt(m); 1. Illustrate through well chosen plots what the "eigenvalues look like". You should do this for many matrices of the same size together, for instance 1000 such matrices of size 32 or 64. Use the MATLAB eig function to get the eigenvalues (note that these will in general by complex and thus your plots should be in the complex plane). What is the pattern? A few well chosen plots are much better than many poorly chosen ones. Make sure to clearly label all plots. 2. What can you say about the limiting behavior of the spectral radius (see Problem 1) as m oo? Justify your answer with numerical evidence. 3. What about the behavior of the 2-norm of such matrices (in MATLAB, simply use norm)? What happens as m oo? Can you relate this to Problem 1? 4. Same question for the condition number (in MATLAB, simply use condest)? Problem 3 (Inspired by Trefethen and Bau) Consider random mxm matrices whose entires are independent samples of the normal distribution with mean zero and standard deviation m-1/2, i..e, sampled from N(0,1/m). In MATLAB, you can construct such matrices through A = randn(m)/sqrt(m); 1. Illustrate through well chosen plots what the "eigenvalues look like". You should do this for many matrices of the same size together, for instance 1000 such matrices of size 32 or 64. Use the MATLAB eig function to get the eigenvalues (note that these will in general by complex and thus your plots should be in the complex plane). What is the pattern? A few well chosen plots are much better than many poorly chosen ones. Make sure to clearly label all plots. 2. What can you say about the limiting behavior of the spectral radius (see Problem 1) as m oo? Justify your answer with numerical evidence. 3. What about the behavior of the 2-norm of such matrices (in MATLAB, simply use norm)? What happens as m oo? Can you relate this to Problem 1? 4. Same question for the condition number (in MATLAB, simply use condest)