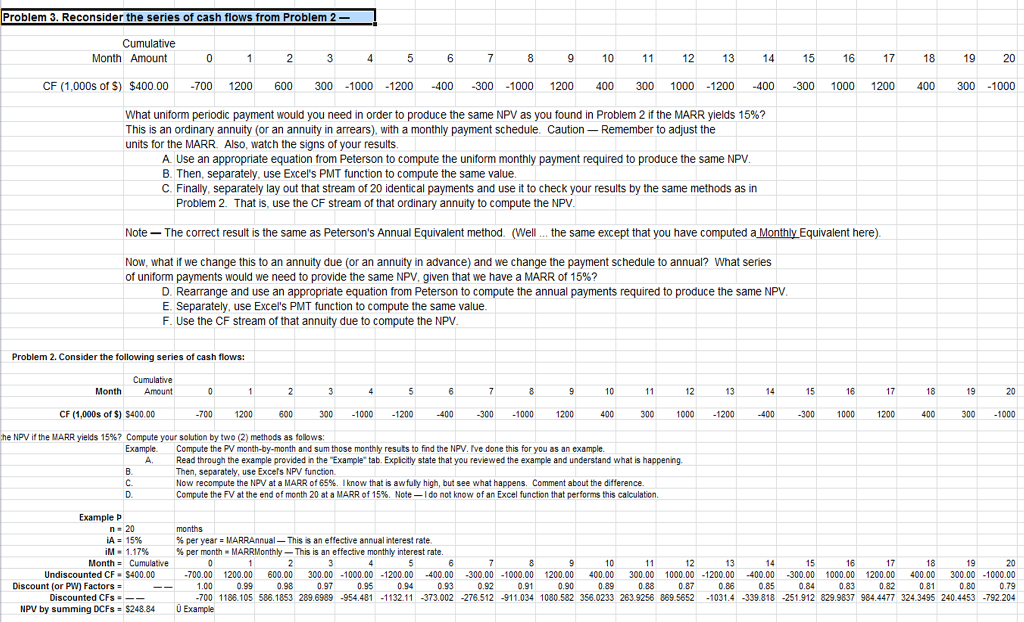
Problem 3. Reconsider the series of cash flows from Problem 2 Cumulative Month Amount 9 10 11 12 3 141561718920 CF (1,000s of S) $400.00 -700 1200 600 300-1000 -1200 400 3001000 00 400300 1000-1200 -300 1000 1200 400 -1000 what uniform periodic payment would you need in order to produce the same NPV as you found in Problem 2 if the MARR yields 15%? This is an ordinary annuity (or an annuity in arrears), with a monthly payment schedule. Caution-Remember to adjust the units for the MARR. Also, watch the signs of your results A Use an appropriate equation from Peterson to compute the uniform monthly payment required to produce the same NPV B. Then, separately, use Excel's PMT function to compute the same value C. Finally, separately lay out that stream of 20 identical payments and use it to check your results by the same methods as in Problem 2. That is, use the CF stream of that ordinary annuity to compute the NPV Note-The correct result is the same as Peterson's Annual Equivalent method.(Well. the same except that you have computed a Monthly Equivalent here). Now, what if we change this to an annuity due (or an annuity in advance) and we change the payment schedule to annual? What series of uniform payments would we need to provide the same NPV, given that we have a MARR of 15%? D. Rearange and use an appropriate equation from Peterson to compute the annual payments required to produce the same NPV E. Separately, use Excel's PMT function to compute the same value F. Use the CF stream of that annuity due to compute the NPV Problem 2. Consider the following series of cash flows 10 12 16 18 CF (1,000s of $) S400.00 -7001200 300 -1000 -1200 300 1000 200 300 1000 -200300 1000 200 300 1000 he NPV if the MARR yields 15%? Compute your solution by two (2) methods as follows: Example. Compute the PV month-by-month and sum those monthly resuts to find the NPV. Ive done this for you as an example A. Read through the example provided in the Example" tab. Expicitly state that you reviewed the example and understand what is happening. Then, separately, use Excers NPV function. Now recompute the NPV at a MAR of 65%. I know that is awfuly high, but see what happens. Comment about the difference. Compute the FV at the end of month 20 at a MARR of 15%. Note-I do not know of an Excel function that performs this calculation. Example P n 20 A: 15% iM-1.17% % per year-MARRAnnual_ This is an effective annual interest rate % per month . MARRMonthly-This is an effective monthly interest rate Month Cumulative 10 12 16 18 Undiscounted CF$400.00 -700.00 200.00 600.00 300.00-1000.00-1200.00 -400.00 -300.00-1000.00 1200.00 400.00 300.00 1000.00-1200.00 400.00 -300.00 1000.00 1200.00 400.00 300.00 -1000.00 00.99 0.98 Discount (or PW) Factors- 0.95 0.94 0.93 0.92 0.91 0.90 0.890.88 85 0.84 0.83 0.82 0.81 0.80 0.79 Discounted CFs_ NPV by summing DCFs $248.84 -700 1186.105 586.1853 289.6989 -954.481-1132.11-373.002-276.512-911.034 1080.582 356.0233 263.9256 869 5652-1031.4-339.818-251 912 829.9837 984.4477 324.3495 240.4453-792.204 Example







