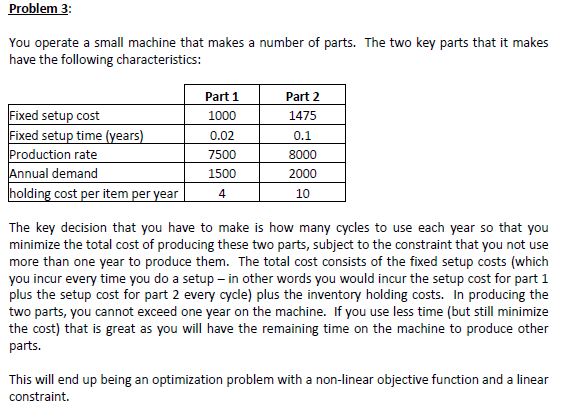
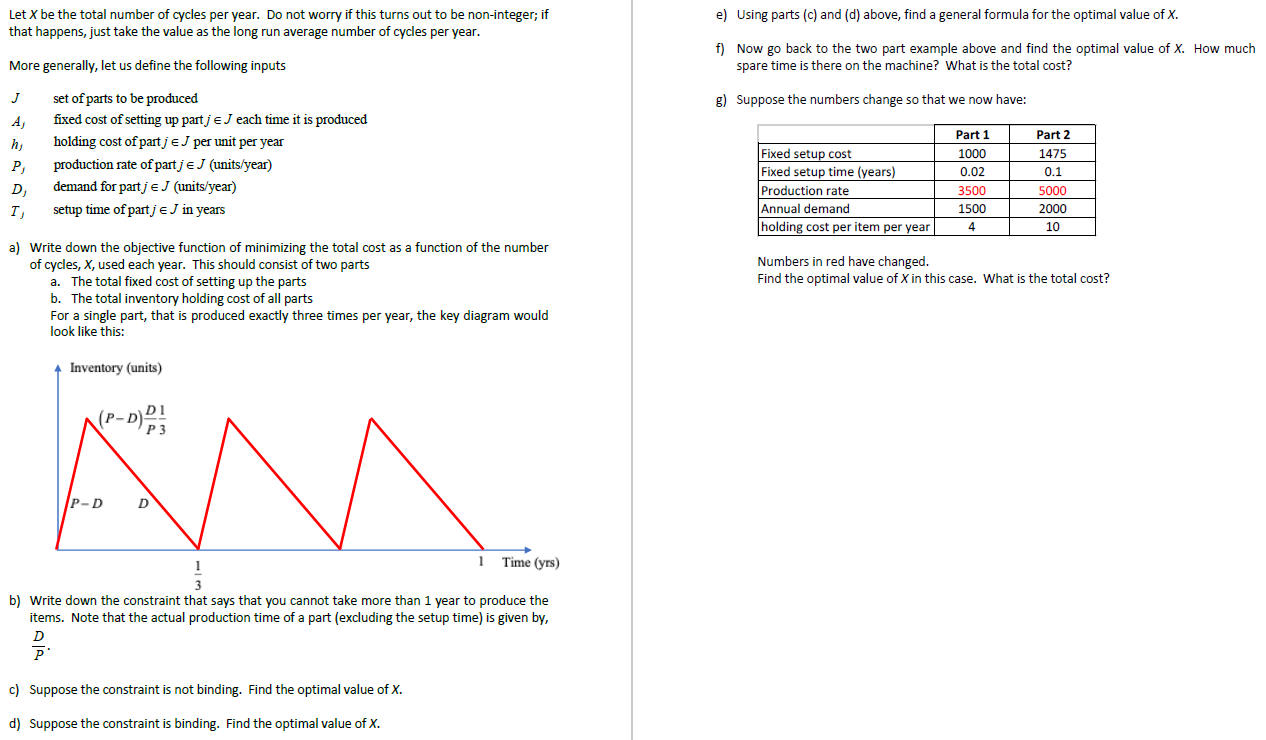
Problem 3: You operate a small machine that makes a number of parts. The two key parts that it makes have the following characteristics: Fixed setup cost Fixed setup time (years) Production rate Annual demand holding cost per item per year Part 1 1000 0.02 7500 1500 Part 2 1475 0.1 8000 2000 10 The key decision that you have to make is how many cycles to use each year so that you minimize the total cost of producing these two parts, subject to the constraint that you not use more than one year to produce them. The total cost consists of the fixed setup costs (which you incur every time you do a setup - in other words you would incur the setup cost for part 1 plus the setup cost for part 2 every cycle) plus the inventory holding costs. In producing the two parts, you cannot exceed one year on the machine. If you use less time (but still minimize the cost) that is great as you will have the remaining time on the machine to produce other parts. This will end up being an optimization problem with a non-linear objective function and a linear constraint. Let X be the total number of cycles per year. Do not worry if this turns out to be non-integer; if that happens, just take the value as the long run average number of cycles per year. e) Using parts (c) and (d) above, find a general formula for the optimal value of X. f) Now go back to the two part example above and find the optimal value of X. How much spare time is there on the machine? What is the total cost? More generally, let us define the following inputs g) Suppose the numbers change so that we now have: I A set of parts to be produced fixed cost of setting up part je J each time it is produced holding cost of parti e per unit per year production rate of partje J (units/year) demand for partj e J (units/year) setup time of partj e J in years Part 2 1475 0.1 D, I Fixed setup cost Fixed setup time (years) Production rate Annual demand holding cost per item per year Part 1 1000 0.02 3500 1500 4 5000 2000 10 Numbers in red have changed. Find the optimal value of X in this case. What is the total cost? a) Write down the objective function of minimizing the total cost as a function of the number of cycles, X, used each year. This should consist of two parts a. The total fixed cost of setting up the parts b. The total inventory holding cost of all parts For a single part, that is produced exactly three times per year, the key diagram would look like this: Inventory (units) (P-D) P-D D 1 Time (yrs) b) Write down the constraint that says that you cannot take more than 1 year to produce the items. Note that the actual production time of a part (excluding the setup time) is given by, D P c) Suppose the constraint is not binding. Find the optimal value of X. d) Suppose the constraint is binding. Find the optimal value of x. Problem 3: You operate a small machine that makes a number of parts. The two key parts that it makes have the following characteristics: Fixed setup cost Fixed setup time (years) Production rate Annual demand holding cost per item per year Part 1 1000 0.02 7500 1500 Part 2 1475 0.1 8000 2000 10 The key decision that you have to make is how many cycles to use each year so that you minimize the total cost of producing these two parts, subject to the constraint that you not use more than one year to produce them. The total cost consists of the fixed setup costs (which you incur every time you do a setup - in other words you would incur the setup cost for part 1 plus the setup cost for part 2 every cycle) plus the inventory holding costs. In producing the two parts, you cannot exceed one year on the machine. If you use less time (but still minimize the cost) that is great as you will have the remaining time on the machine to produce other parts. This will end up being an optimization problem with a non-linear objective function and a linear constraint. Let X be the total number of cycles per year. Do not worry if this turns out to be non-integer; if that happens, just take the value as the long run average number of cycles per year. e) Using parts (c) and (d) above, find a general formula for the optimal value of X. f) Now go back to the two part example above and find the optimal value of X. How much spare time is there on the machine? What is the total cost? More generally, let us define the following inputs g) Suppose the numbers change so that we now have: I A set of parts to be produced fixed cost of setting up part je J each time it is produced holding cost of parti e per unit per year production rate of partje J (units/year) demand for partj e J (units/year) setup time of partj e J in years Part 2 1475 0.1 D, I Fixed setup cost Fixed setup time (years) Production rate Annual demand holding cost per item per year Part 1 1000 0.02 3500 1500 4 5000 2000 10 Numbers in red have changed. Find the optimal value of X in this case. What is the total cost? a) Write down the objective function of minimizing the total cost as a function of the number of cycles, X, used each year. This should consist of two parts a. The total fixed cost of setting up the parts b. The total inventory holding cost of all parts For a single part, that is produced exactly three times per year, the key diagram would look like this: Inventory (units) (P-D) P-D D 1 Time (yrs) b) Write down the constraint that says that you cannot take more than 1 year to produce the items. Note that the actual production time of a part (excluding the setup time) is given by, D P c) Suppose the constraint is not binding. Find the optimal value of X. d) Suppose the constraint is binding. Find the optimal value of x








