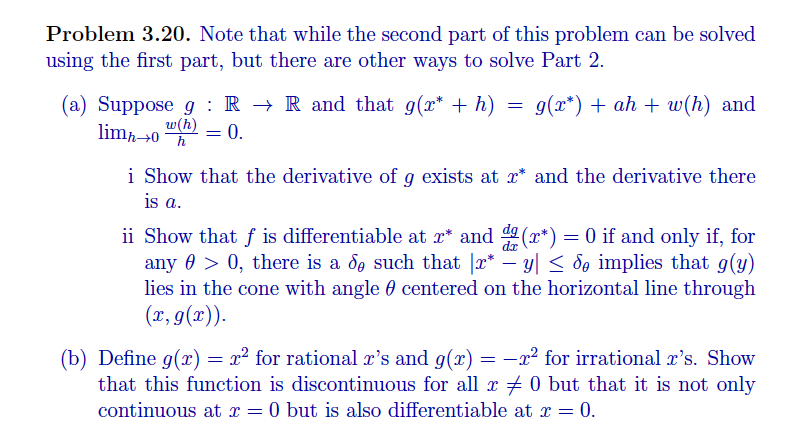
Problem 3.20. Note that while the second part of this problem can be solved using the first part, but there are other ways to solve Part 2. (a) Suppose 9 R R and that g(x* + h) limh0 (h) = 0. = g(x)+ah+w(h) and i Show that the derivative of g exists at x* and the derivative there is a. ii Show that f is differentiable at r* and d (x*) = 0 if and only if, for any > 0, there is a de such that |x* y| de implies that g(y) lies in the cone with angle 0 centered on the horizontal line through (x, g(x)). - (b) Define g(x) = x for rational x's and g(x) = x for irrational x's. Show that this function is discontinuous for all x 0 but that it is not only continuous at x = 0 but is also differentiable at x = 0.