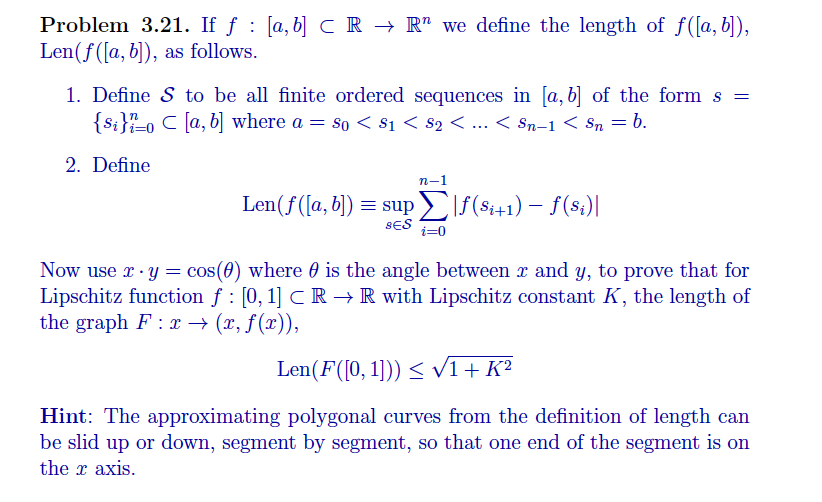
Problem 3.21. If : [a,b] C R R we define the length of ([a, b]), Len(f([a, b]), as follows. 1. Define S to be all finite ordered sequences in [a, b] of the form s = {S} [a, b] where a = 50 < $1 < 82 < ... < Sn1 < Sn = b. 2. Define n-1 Len(f([a, b]) = sup|f($i+1) (Si)|| BES i=0 - Now use x-y = cos(0) where 0 is the angle between 2 and y, to prove that for Lipschitz function f : [0, 1] C R R with Lipschitz constant K, the length of the graph Fx (x, f(x)), Len(F([0, 1])) 1+K Hint: The approximating polygonal curves from the definition of length can be slid up or down, segment by segment, so that one end of the segment is on the x axis.