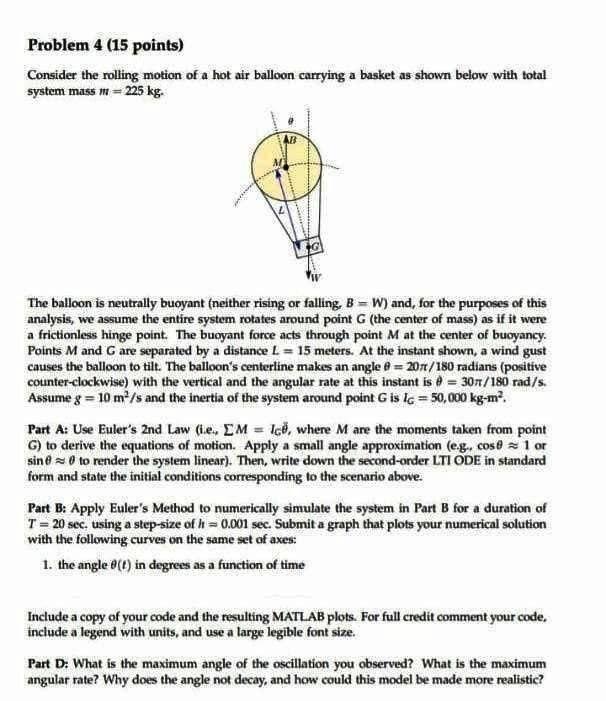
Problem 4 (15 points) Consider the rolling motion of a hot air balloon carrying a basket as shown below with total system mass m=225 kg. = The balloon is neutrally buoyant (neither rising or falling, B = W) and, for the purposes of this analysis, we assume the entire system rotates around point G (the center of mass) as if it were a frictionless hinge point. The buoyant force acts through point M at the center of buoyancy. Points M and G are separated by a distance L = 15 meters. At the instant shown, a wind gust causes the balloon to tilt. The balloon's centerline makes an angle 8 = 20/180 radians (positive counter-clockwise) with the vertical and the angular rate at this instant is 8 = 30/180 rad/s. Assume g = 10 m/s and the inertia of the system around point Gis is = 50,000 kg-m? Part A: Use Euler's 2nd Law (ie, M = Ice, where M are the moments taken from point G) to derive the equations of motion. Apply a small angle approximation (eg, cos z 1 or sine = 0 to render the system linear). Then, write down the second-order LTI ODE in standard form and state the initial conditions corresponding to the scenario above. Part B: Apply Euler's Method to numerically simulate the system in Part B for a duration of T = 20 sec. using a step-size of h = 0.001 sec. Submit a graph that plots your numerical solution with the following curves on the same set of axes: 1. the angle e(t) in degrees as a function of time Include a copy of your code and the resulting MATLAB plots. For full credit comment your code. include a legend with units, and use a large legible font size. Part D: What is the maximum angle of the oscillation you observed? What is the maximum angular rate? Why does the angle not decay, and how could this model be made more realistic? Problem 4 (15 points) Consider the rolling motion of a hot air balloon carrying a basket as shown below with total system mass m=225 kg. = The balloon is neutrally buoyant (neither rising or falling, B = W) and, for the purposes of this analysis, we assume the entire system rotates around point G (the center of mass) as if it were a frictionless hinge point. The buoyant force acts through point M at the center of buoyancy. Points M and G are separated by a distance L = 15 meters. At the instant shown, a wind gust causes the balloon to tilt. The balloon's centerline makes an angle 8 = 20/180 radians (positive counter-clockwise) with the vertical and the angular rate at this instant is 8 = 30/180 rad/s. Assume g = 10 m/s and the inertia of the system around point Gis is = 50,000 kg-m? Part A: Use Euler's 2nd Law (ie, M = Ice, where M are the moments taken from point G) to derive the equations of motion. Apply a small angle approximation (eg, cos z 1 or sine = 0 to render the system linear). Then, write down the second-order LTI ODE in standard form and state the initial conditions corresponding to the scenario above. Part B: Apply Euler's Method to numerically simulate the system in Part B for a duration of T = 20 sec. using a step-size of h = 0.001 sec. Submit a graph that plots your numerical solution with the following curves on the same set of axes: 1. the angle e(t) in degrees as a function of time Include a copy of your code and the resulting MATLAB plots. For full credit comment your code. include a legend with units, and use a large legible font size. Part D: What is the maximum angle of the oscillation you observed? What is the maximum angular rate? Why does the angle not decay, and how could this model be made more realistic







