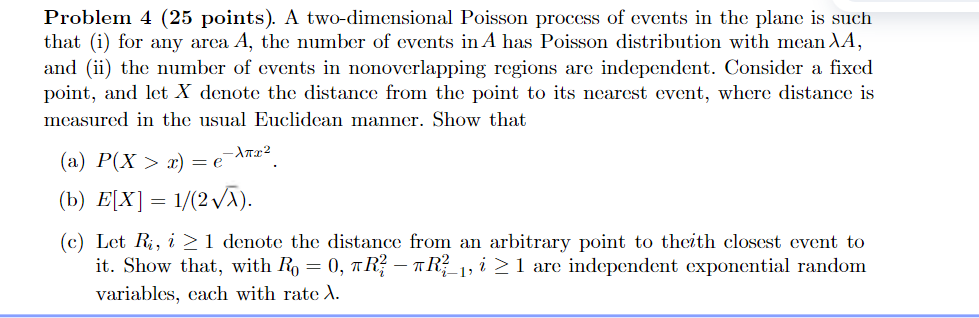
Problem 4 (25 points). A two-dimensional Poisson process of events in the plane is such that (i) for any area A, the number of events in A has Poisson distribution with mean AA, and (ii) the number of events in nonoverlapping regions are independent. Consider a fixed point, and let X denote the distance from the point to its nearest event, where distance is measured in the usual Euclidean manner. Show that (a) P(X > x) = e - (b) E[X] = 1/(2). (c) Let Ri, i 1 denote the distance from an arbitrary point to theith closest event to - it. Show that, with R = 0, R R, i 1 are independent exponential random variables, each with rate A.