
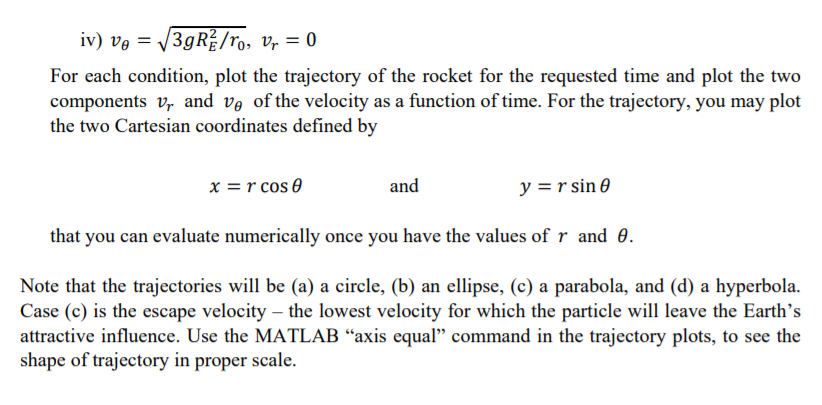
Problem 4: Consider the 2D motion of a rocket moving around the Earth. Consider Earth as being stationary and the attractive force it induces on the rocket is directed towards the center of thoe Earth and has a magnitude of mg( ) In the equation: . r is the current distance of the rocket from the center of the Earth RE 6371 km is the radius of the Earth - m is the mass of the rocket g 9.81 m/s2 is the usual acceleration due to gravity (on the Earth's surface) a) Write the equations of motion of the rocket in an appropriate polar coordinate system. b) Integrate the equations of motion for the system numerically using MATLAB and ode45 for the conditions shown below. The initial distance between the rocket and the Earth is ro. The initial velocity of the rocket towards the center of the Earth and perpendicular to it are given by vr and vg respectively. Assume that the angle 6 is zero initially and that ro-5RE and 0. Carry out the integration at a time step of 10s for 80,000 time steps (i.e., a total simulation time of 800,000 seconds) for each of the initial velocities specified below: For each condition, plot the trajectory of the rocket for the requested time and plot the two components ur and Ug of the velocity as a function of time. For the trajectory, you may plot the two Cartesian coordinates defined by x = r cos anl y r sin that you can evaluate numerically once you have the values of r and . Note that the trajectories will be (a) a circle, (b) an ellipse, (c) a parabola, and (d) a hyperbola. Case (c) is the escape velocity - the lowest velocity for which the particle will leave the Earth's attractive influence. Use the MATLAB "axis equal" command in the trajectory plots, to see the shape of trajectory in proper scale Problem 4: Consider the 2D motion of a rocket moving around the Earth. Consider Earth as being stationary and the attractive force it induces on the rocket is directed towards the center of thoe Earth and has a magnitude of mg( ) In the equation: . r is the current distance of the rocket from the center of the Earth RE 6371 km is the radius of the Earth - m is the mass of the rocket g 9.81 m/s2 is the usual acceleration due to gravity (on the Earth's surface) a) Write the equations of motion of the rocket in an appropriate polar coordinate system. b) Integrate the equations of motion for the system numerically using MATLAB and ode45 for the conditions shown below. The initial distance between the rocket and the Earth is ro. The initial velocity of the rocket towards the center of the Earth and perpendicular to it are given by vr and vg respectively. Assume that the angle 6 is zero initially and that ro-5RE and 0. Carry out the integration at a time step of 10s for 80,000 time steps (i.e., a total simulation time of 800,000 seconds) for each of the initial velocities specified below: For each condition, plot the trajectory of the rocket for the requested time and plot the two components ur and Ug of the velocity as a function of time. For the trajectory, you may plot the two Cartesian coordinates defined by x = r cos anl y r sin that you can evaluate numerically once you have the values of r and . Note that the trajectories will be (a) a circle, (b) an ellipse, (c) a parabola, and (d) a hyperbola. Case (c) is the escape velocity - the lowest velocity for which the particle will leave the Earth's attractive influence. Use the MATLAB "axis equal" command in the trajectory plots, to see the shape of trajectory in proper scale








