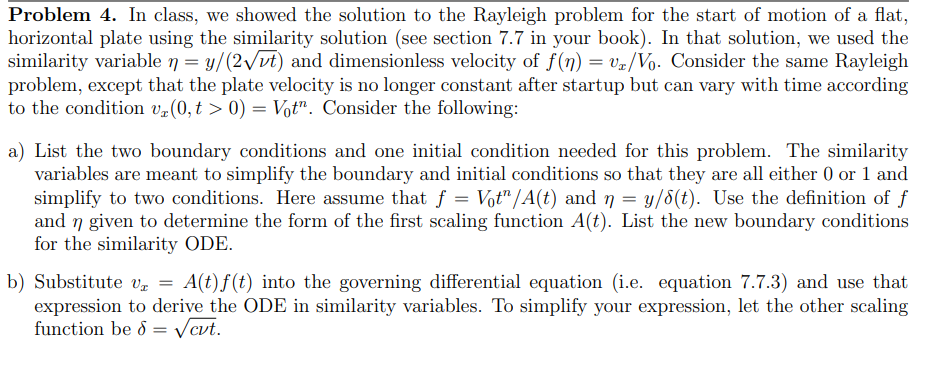
Problem 4. In class, we showed the solution to the Rayleigh problem for the start of motion of a flat, horizontal plate using the similarity solution (see section 7.7 in your book). In that solution, we used the similarity variable n = y/(2vt) and dimensionless velocity of f(n) = vx/Vo. Consider the same Rayleigh problem, except that the plate velocity is no longer constant after startup but can vary with time according to the condition v(0,t > 0) = Vot". Consider the following: a) List the two boundary conditions and one initial condition needed for this problem. The similarity variables are meant to simplify the boundary and initial conditions so that they are all either 0 or 1 and simplify to two conditions. Here assume that f = Vot"/A(t) and = y/8(t). Use the definition of f and given to determine the form of the first scaling function A(t). List the new boundary conditions for the similarity ODE. b) Substitute v = A(t)f(t) into the governing differential equation (i.e. equation 7.7.3) and use that expression to derive the ODE in similarity variables. To simplify your expression, let the other scaling function be cvt. =