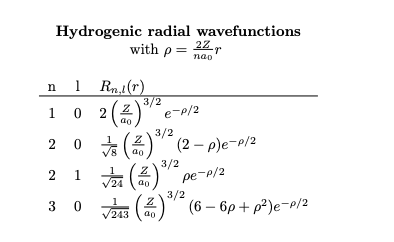
Problem 5 Consider the hydrogenic wavefunctions Vn,l,m = Rn,!(r)Y,m, (0, 0) for an elec- tron in a hydrogen atom (Z = 1). The electron is in the 2s state. a) Determine the location of the radial node in terms of ao. For a radial node, only the Rn, part of the wavefunction is important R2,0()()(2-r/ao)e-r/200 = 0 = which gives r = 2a0. b) Calculate the most probable radius of an electron in a 2s state, and com- pare this radius with the most probable radius of the 1s state (ao). P(r) = r Ro(r) = 3 () r (r/a - 4r/ao+4)e-r/00 sets r/ao, then: P(r) = = 800 (482-483 +84)e-* d = 80 (88-1682 +883 - 84)e-s = 0 This implies 88-1682 +883-84 = 0 we can write s(8-16s+ 8s s) = s(2s) (4-6s+s) = 0 using r = a.s, this gives roots at r = 0, r = 2a0 and r = (35)ao The solution r = 0 is the origin, r = 2a0 is the node, r = (3-5)ao is the first maximum, and r = (3+ 5) ao = 5.24a0 is the second maximum. The second maximum has a much higher value for P(r), implying that r = (3+5)ao is the most probable radius. Sincer > ao, the radius of the 2s state is larger than that of the 1s. Hence, the 2s orbital is more extended than the 1s orbital. Hydrogenic radial wavefunctions with p = 22, nao 1 0 2 (2 n 1 Rnt(r) 3/2 e-p/2 3/2 (2-p)e-p/2 3/2 20 2 1 (2) 124 (4) 30 243 ( 00 3/2 pe-p/2 (6-6p+p)e-p/2