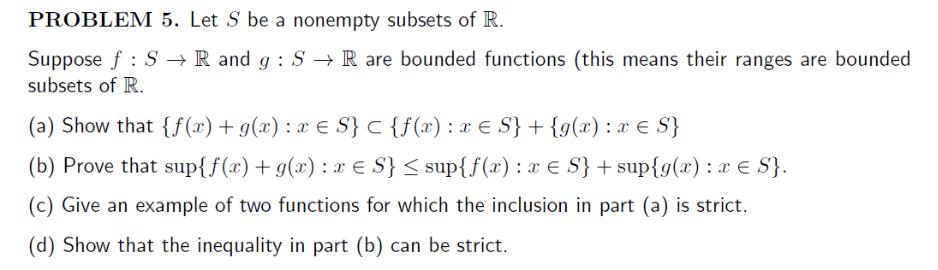
PROBLEM 5. Let S be a nonempty subsets of R. Suppose f: S R and g: S R are bounded functions (this means their ranges are bounded subsets of R. (a) Show that {f(x) + g(x): x S} c{f(x): x S} + {g(x) : x = S} (b) Prove that sup{f(x) + g(x) : x = S} sup{f(x) : x S} + sup{g(x) : x = S}. (c) Give an example of two functions for which the inclusion in part (a) is strict. (d) Show that the inequality in part (b) can be strict.