


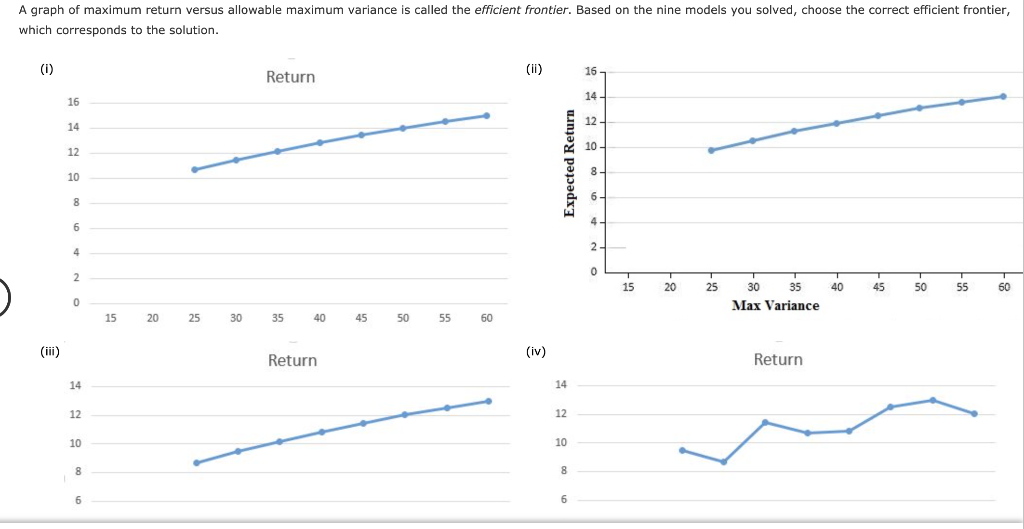
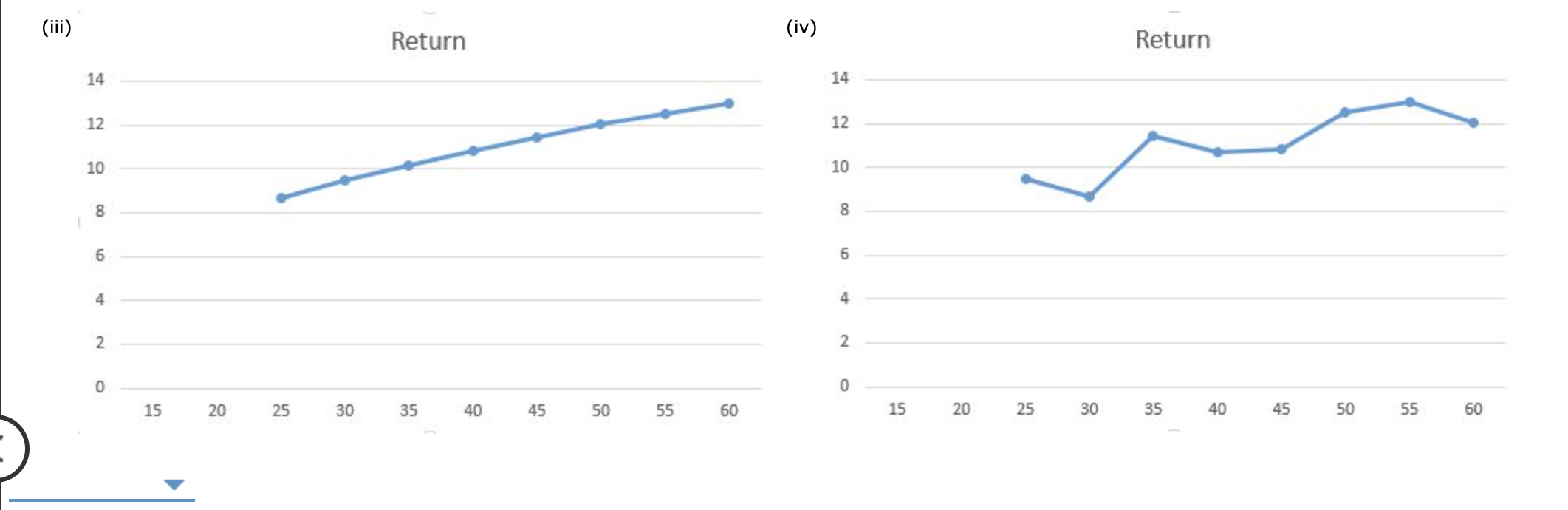
Problem 8-18 (Algorithmic) Refer to the Markowitz portfolio model, which maximizes expected return subject to a constraint that the variance of the portfolio must be less than or equal to some specified amount: Let: FS = proportion of portfolio invested in the foreign stock mutual fund IB = proportion of portfolio invested in the intermediate-term bond fund LG = proportion of portfolio invested in the large-cap growth fund LV = proportion of portfolio invested in the large-cap value fund SG = proportion of portfolio invested in the small-cap growth fund SV = proportion of portfolio invested in the small-cap value fund R = the expected return of the portfolio Rs = the return of the portfolio in years Var = the variance of the portfolio Max R s.t. 10.51 FS + 18.09 IB + 32.86 LG + 32.81 LV + 33.89 SG + 25.01 SV = R1 25.77 SV = R2 13.57 FS + 3.71 IB + 19.16 LG + 21.06 LV + 19.85 SG + 13.82 FS + 7.96 IB + 33.73 LG + 13.38 LV + 4.22 SG - 7.15 SV = R3 5.88 SV = R4 45.87 FS - 1.78 IB + 41.91 LG + 7.51 LV + 59.13 SG + -22.38 FS + 7.81 IB - 23.71 LG - 5.82 LV - 9.47 SG + 17.76 SV = R5 FS + IB + LG + LV + SG + SV = 1 ie R Max s.t. 10.51 FS + 18.09 IB + 32.86 LG + 32.81 LV + 33.89 SG + 25.01 SV = R1 13.57 FS + 3.71 IB + 19.16 LG + 21.06 LV + 19.85 SG + 25.77 SV = R2 13.82 FS + 7.96 IB + 33.73 LG + 13.38 LV + 4.22 SG 7.15 SV = R3 45.87 FS 1.78 IB + 41.91 LG + 7.51 LV + 59.13 SG + 5.88 SV R4 -22.38 FS + 7.81 IB 23.71 LG - 5.82 LV 9.47 SG + 17.76 SV = R5 FS + IB + LG + LV + SG + SV = 1 5 1 = R Io io 5 (R$ R) s Var s=1 FS, IB, LG, LV, SG, SV 20 Use the model to solve a series of models by varying the maximum allowable variance from 20 to 60 in increments of 5 and solving for the maximum return for each (solve a total of nine models). Round your answers to 3 decimal places. If there is no optimal solution, enter "NA" as your answer. Max Variance Exp Return 20 25 30 35 40 45 50 55 60 A graph of maximum return versus allowable maximum variance is called the efficient frontier. Based on the nine models you solved, choose the correct efficient frontier, which corresponds to the solution. (1) (II) Return 16 16 14 14 12- 10 12 Expected Return 8 - 10 6 8 4 6 4 2 0 2 T 15 20 25 40 45 50 55 60 30 35 Max Variance 0 15 20 25 30 35 40 45 50 55 60 ( (iv) Return Return 14 14 12 12 10 10 8 8 6 6 (iii) (iv) Return Return 14 14 12 12 10 10 8 8 6 6 4 4 2 2 o 0 15 20 25 30 35 40 45 50 55 60 15 20 25 30 35 40 45 50 55 60 Problem 8-18 (Algorithmic) Refer to the Markowitz portfolio model, which maximizes expected return subject to a constraint that the variance of the portfolio must be less than or equal to some specified amount: Let: FS = proportion of portfolio invested in the foreign stock mutual fund IB = proportion of portfolio invested in the intermediate-term bond fund LG = proportion of portfolio invested in the large-cap growth fund LV = proportion of portfolio invested in the large-cap value fund SG = proportion of portfolio invested in the small-cap growth fund SV = proportion of portfolio invested in the small-cap value fund R = the expected return of the portfolio Rs = the return of the portfolio in years Var = the variance of the portfolio Max R s.t. 10.51 FS + 18.09 IB + 32.86 LG + 32.81 LV + 33.89 SG + 25.01 SV = R1 25.77 SV = R2 13.57 FS + 3.71 IB + 19.16 LG + 21.06 LV + 19.85 SG + 13.82 FS + 7.96 IB + 33.73 LG + 13.38 LV + 4.22 SG - 7.15 SV = R3 5.88 SV = R4 45.87 FS - 1.78 IB + 41.91 LG + 7.51 LV + 59.13 SG + -22.38 FS + 7.81 IB - 23.71 LG - 5.82 LV - 9.47 SG + 17.76 SV = R5 FS + IB + LG + LV + SG + SV = 1 ie R Max s.t. 10.51 FS + 18.09 IB + 32.86 LG + 32.81 LV + 33.89 SG + 25.01 SV = R1 13.57 FS + 3.71 IB + 19.16 LG + 21.06 LV + 19.85 SG + 25.77 SV = R2 13.82 FS + 7.96 IB + 33.73 LG + 13.38 LV + 4.22 SG 7.15 SV = R3 45.87 FS 1.78 IB + 41.91 LG + 7.51 LV + 59.13 SG + 5.88 SV R4 -22.38 FS + 7.81 IB 23.71 LG - 5.82 LV 9.47 SG + 17.76 SV = R5 FS + IB + LG + LV + SG + SV = 1 5 1 = R Io io 5 (R$ R) s Var s=1 FS, IB, LG, LV, SG, SV 20 Use the model to solve a series of models by varying the maximum allowable variance from 20 to 60 in increments of 5 and solving for the maximum return for each (solve a total of nine models). Round your answers to 3 decimal places. If there is no optimal solution, enter "NA" as your answer. Max Variance Exp Return 20 25 30 35 40 45 50 55 60 A graph of maximum return versus allowable maximum variance is called the efficient frontier. Based on the nine models you solved, choose the correct efficient frontier, which corresponds to the solution. (1) (II) Return 16 16 14 14 12- 10 12 Expected Return 8 - 10 6 8 4 6 4 2 0 2 T 15 20 25 40 45 50 55 60 30 35 Max Variance 0 15 20 25 30 35 40 45 50 55 60 ( (iv) Return Return 14 14 12 12 10 10 8 8 6 6 (iii) (iv) Return Return 14 14 12 12 10 10 8 8 6 6 4 4 2 2 o 0 15 20 25 30 35 40 45 50 55 60 15 20 25 30 35 40 45 50 55 60











