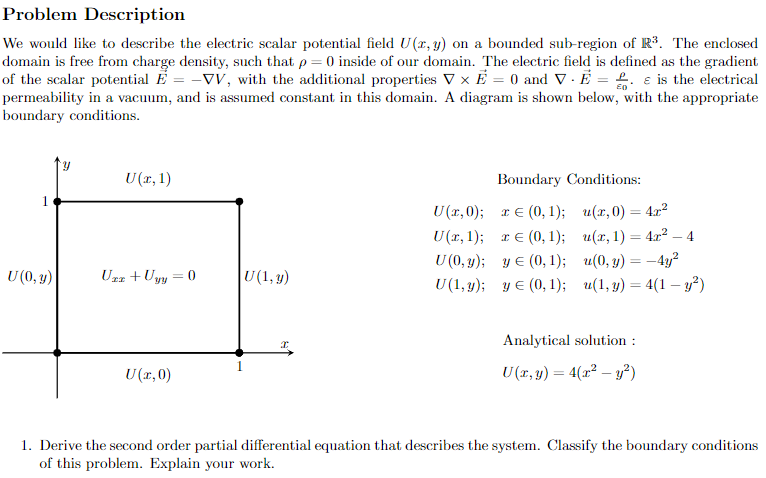
Problem Description E We would like to describe the electric scalar potential field U(x, y) on a bounded sub-region of R. The enclosed domain is free from charge density, such that p = 0 inside of our domain. The electric field is defined as the gradient of the scalar potential E = -VV, with the additional properties V x E = 0 and V E = . is the electrical permeability in a vacuum, and is assumed constant in this domain. A diagram is shown below, with the appropriate boundary conditions. Y U(x, 1) Boundary Conditions: 1 U(x, 0); (0,1); u(x, 0) = 4x U(x, 1); (0,1); u(x, 1) = 4x - 4 U(0,y); y(0, 1); u(0, y) = -4y U(0,y) Uxx + Uyy = 0 U(1,y) U(1,y); y(0, 1); u(1, y)=4(1- y) 1 U(x, 0) Analytical solution: U(x, y)=4(x-y) 1. Derive the second order partial differential equation that describes the system. Classify the boundary conditions of this problem. Explain your work.