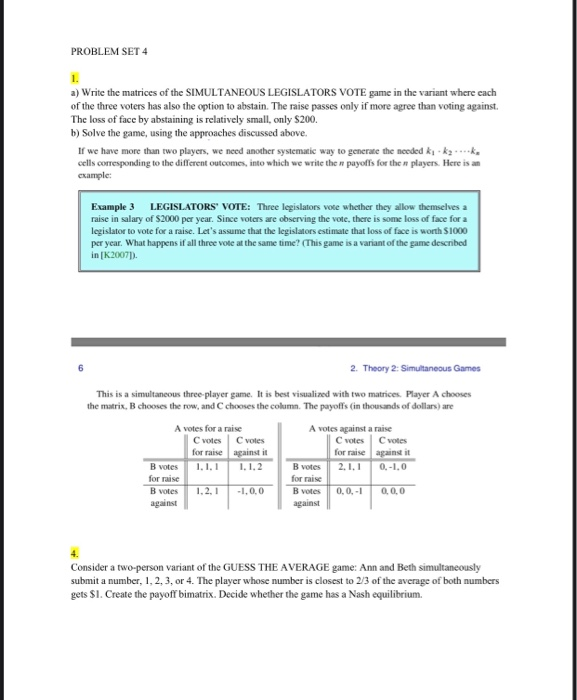
PROBLEM SET 4 1. a) Write the matrices of the SIMULTANEOUS LEGISLATORS VOTE game in the variant where each of the three voters has also the option to abstain. The raise passes only if more agree than voting against The loss of face by abstaining is relatively small, only $200. b) Solve the game, using the approaches discussed above. If we have more than two players, we need another systematic way to generate the needed k.ky....ke cells corresponding to the different outcomes, into which we write the payoffs for the n players. Here is an example: Example 3 LEGISLATORS VOTE: Three legislators vote whether they allow themselves a raise in salary of S2000 per year. Since voters are observing the vote, there is some loss of face for a legis to vote for a raise. Let's assume that the legi ors estimate that loss of face is worth $1000 per year. What happens if all three vote at the same time? (This game is a variant of the game described in [2007]). 2. Theory 2: Simultaneous Games This is a simultaneous three player game. It is best visualized with two matrices. Player A chooses the matrix, B chooses the row, and C chooses the column. The payoffs (in thousands of dollars) are A votes for a raise A votes against a raise C votes C votes C votes C votes for raise against it for raise against it B votes 1.1.1 1.1.2 B votes 2.1.1 0.-1.0 for raise for raise B votes 1.2.1 -1.0.0 B votes 0.0,- 0.0.0 against against Consider a two-person variant of the GUESS THE AVERAGE game: Ann and Beth simultaneously submit a number, 1, 2, 3, or 4. The player whose number is closest to 2/3 of the average of both numbers gets $1. Create the payoff bimatrix. Decide whether the game has a Nash equilibrium. In the TWO BARS example a lack of tourists increases competition. Assume the number of natives is 4000. For which number of tourists would both bars choose 54 as the price for a beer? For which tourist numbers is $2 possible, and for which tourist numbers is SS possible? Example 4 TWO BARS: Each one of two bars charges its own price for a beer, either 52, 54, SS. The cost of obtaining and serving the beer can be neglected. It is expected that 6000 beers per month are drunk in a bar by tourists, who choose one of the two bars randomly, and 4000 beers per month we drunk by natives who go to the bar with the lowest price and split evenly in care both bars offer the same price. What prices would the bars select? 2009 The game is all games considered so far is symmetrie. Let me illustrate in one instance how to compute the payoffs. If bar A charges 52 and bar charges 54, then all matives will choosebur A 2. Theory 2 Simreous Games Therefore har A will serve 4000 beers to the natives, and 3000 beers to tourists, serving 7000 beers in total, making 70002 - 14000 dollars. Bar will only serve 2000 beers to tourists, making 30004 - 12000 dollars The payoff matrix, with values in thousands of dollars, is 210,10 14.12 14,15 12.14 20.20 28.15 15.14 15.28 25,25 Por each her, move 4" strictly dominates move "2", therefore we could climinate both moves 2.0 get the reduced game 5 4 20, 2015 15,28 25.25 Now, but not before the elimination, more strictly dominates move "5". Therefore we eliminate these moves for both players as well and arrive at a game with only one option "4", forcach player, and a payoff of 20000 for each. Therefore both players will choose 54 as the price of the beer A weaker condition is weak domination. Ann's move weakly dominates another one of her moves if it yields at least the same payoff for Ann in all cases generated by combinations of moves of the other players and in at least one case an even better payoff. So the weakly dominating move is never worse than the weekly dominated one, and sometimes it is better. The common wide is that iterated elimination of weakly dominated moves, IEWDis not something that should performed automatically. Weakly dominated moves may still be played in particular in cases where the weakness of the weakly dominated move appears in a combination with other player's mones that are known not to be played by them. This opinion is also used different behavior of Nash equilibria (discussed in Section 2.4) under WD and TESD. Analyze the following game. Create payoff bimatrices consistent with the information given. Explain your choices. Then find the maximin moves, domination, and all pure Nash equilibria. SELECTING CLASS: Adam, Bill, and Cindy are registering for a foreign language class independently and simultaneously. The available classes are ITA100 and FRE100. They do not care much which, but they care with whom they share the class. Bill and Cindy want to be in the same class but want to avoid Adam. Adam wants to be in the same class as Bill or Cindy, or even better, both. PROBLEM SET 4 1. a) Write the matrices of the SIMULTANEOUS LEGISLATORS VOTE game in the variant where each of the three voters has also the option to abstain. The raise passes only if more agree than voting against The loss of face by abstaining is relatively small, only $200. b) Solve the game, using the approaches discussed above. If we have more than two players, we need another systematic way to generate the needed k.ky....ke cells corresponding to the different outcomes, into which we write the payoffs for the n players. Here is an example: Example 3 LEGISLATORS VOTE: Three legislators vote whether they allow themselves a raise in salary of S2000 per year. Since voters are observing the vote, there is some loss of face for a legis to vote for a raise. Let's assume that the legi ors estimate that loss of face is worth $1000 per year. What happens if all three vote at the same time? (This game is a variant of the game described in [2007]). 2. Theory 2: Simultaneous Games This is a simultaneous three player game. It is best visualized with two matrices. Player A chooses the matrix, B chooses the row, and C chooses the column. The payoffs (in thousands of dollars) are A votes for a raise A votes against a raise C votes C votes C votes C votes for raise against it for raise against it B votes 1.1.1 1.1.2 B votes 2.1.1 0.-1.0 for raise for raise B votes 1.2.1 -1.0.0 B votes 0.0,- 0.0.0 against against Consider a two-person variant of the GUESS THE AVERAGE game: Ann and Beth simultaneously submit a number, 1, 2, 3, or 4. The player whose number is closest to 2/3 of the average of both numbers gets $1. Create the payoff bimatrix. Decide whether the game has a Nash equilibrium. In the TWO BARS example a lack of tourists increases competition. Assume the number of natives is 4000. For which number of tourists would both bars choose 54 as the price for a beer? For which tourist numbers is $2 possible, and for which tourist numbers is SS possible? Example 4 TWO BARS: Each one of two bars charges its own price for a beer, either 52, 54, SS. The cost of obtaining and serving the beer can be neglected. It is expected that 6000 beers per month are drunk in a bar by tourists, who choose one of the two bars randomly, and 4000 beers per month we drunk by natives who go to the bar with the lowest price and split evenly in care both bars offer the same price. What prices would the bars select? 2009 The game is all games considered so far is symmetrie. Let me illustrate in one instance how to compute the payoffs. If bar A charges 52 and bar charges 54, then all matives will choosebur A 2. Theory 2 Simreous Games Therefore har A will serve 4000 beers to the natives, and 3000 beers to tourists, serving 7000 beers in total, making 70002 - 14000 dollars. Bar will only serve 2000 beers to tourists, making 30004 - 12000 dollars The payoff matrix, with values in thousands of dollars, is 210,10 14.12 14,15 12.14 20.20 28.15 15.14 15.28 25,25 Por each her, move 4" strictly dominates move "2", therefore we could climinate both moves 2.0 get the reduced game 5 4 20, 2015 15,28 25.25 Now, but not before the elimination, more strictly dominates move "5". Therefore we eliminate these moves for both players as well and arrive at a game with only one option "4", forcach player, and a payoff of 20000 for each. Therefore both players will choose 54 as the price of the beer A weaker condition is weak domination. Ann's move weakly dominates another one of her moves if it yields at least the same payoff for Ann in all cases generated by combinations of moves of the other players and in at least one case an even better payoff. So the weakly dominating move is never worse than the weekly dominated one, and sometimes it is better. The common wide is that iterated elimination of weakly dominated moves, IEWDis not something that should performed automatically. Weakly dominated moves may still be played in particular in cases where the weakness of the weakly dominated move appears in a combination with other player's mones that are known not to be played by them. This opinion is also used different behavior of Nash equilibria (discussed in Section 2.4) under WD and TESD. Analyze the following game. Create payoff bimatrices consistent with the information given. Explain your choices. Then find the maximin moves, domination, and all pure Nash equilibria. SELECTING CLASS: Adam, Bill, and Cindy are registering for a foreign language class independently and simultaneously. The available classes are ITA100 and FRE100. They do not care much which, but they care with whom they share the class. Bill and Cindy want to be in the same class but want to avoid Adam. Adam wants to be in the same class as Bill or Cindy, or even better, both