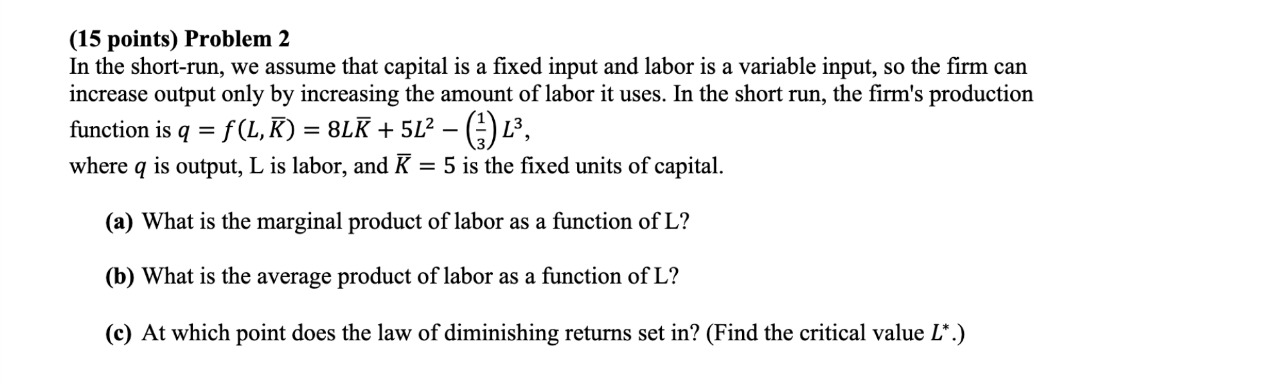
Production.

1. Consider the Cobb-Douglas production function f(x,y) = 12x0.4y0.8.

(A) Find the intensities (? and 1 ? ?) of the two factors of production. Does this firm have decreasing, increasing, or constant returns to scale? What percentage of the firm's total production costs will be spent on good x?

(B) Suppose the firm decides to increase its input bundle (x, y) by 10%. That is, it inputs 10% more units of good x and 10% more units of good y. What is the percent increase in output?

(C) Suppose the firm has a production quota of q = 1000 units, and the firm inputs x = 100 units of the first good. How many units of the second good does it need to use to meet the quota?

(D) Assume the firm has a production quota of q = 2000 units, and the input prices are (px,py) = (7,19). Find the minimized cost C(2000) and the conditional factor demands (x?,y?).

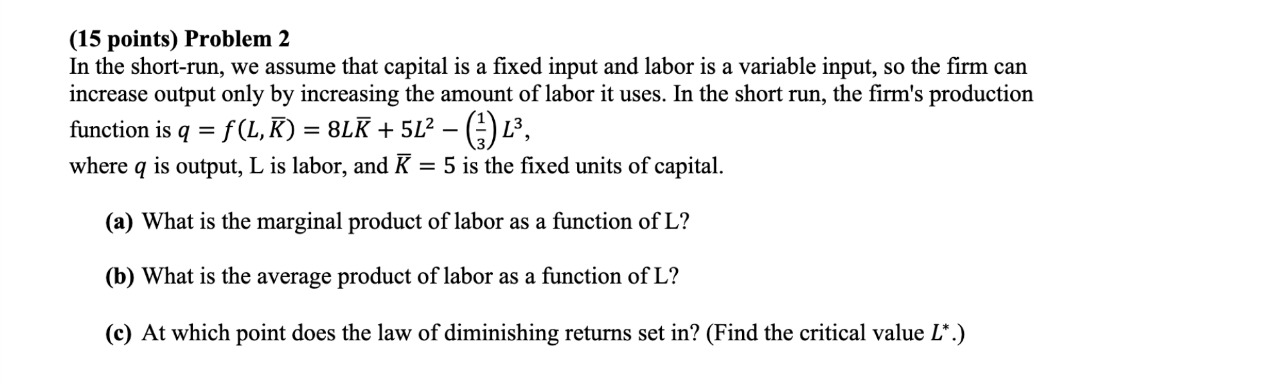
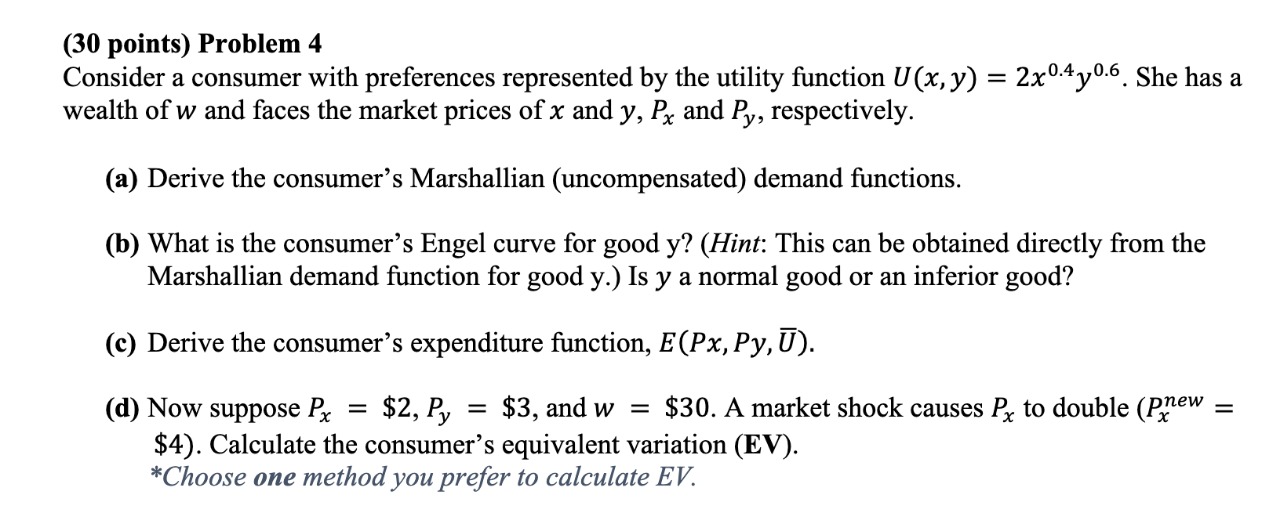
Problem 2. Consider the linear (perfect substitutes) production function f (x, y) = 12.7x + 19.4y.

(A) How many units of good y would be a perfect substitute for 1 unit of good x? What is the slope of

the firm's isoquants?

(B) Suppose the input prices are (px,py) = (5,8). What is the slope of the isocost lines? How much output does the firm get when it inputs $1 worth of good x? How much output does the firm get when it inputs $1 worth of good y?

(C) Suppose this firm has a production quota of q = 500 units. Find the minimized cost C(500) and the corresponding conditional factor demands.

(D) Draw the firm's level-500 isoquant, as well as the isocost lines. Indicate the cost minimizer on your diagram.

Problem 3. Consider the Leontiev (perfect complements) production function f (x, y) = M in x , y . 9.6 5.2

(A) How many units of good y would be a perfect complement for 1 unit of good x? What is the equation of the firm's kink line?

(B) Assume the firm has a production quota of q = 400 units. Graph the firm's level-400 isoquant. What are the coordinates of the kink?

(C) Suppose the input prices are (px,py) = (16,9). Find the minimized cost C(400). What is the cost minimizing input bundle (x?, y?)?

(D) Give a complete geometric illustration of this firm's cost minimization. On a single diagram, draw the firm's level-400 isoquant, the isocost lines, and the cost minimizing input bundle.

Problem 4. Suppose that a firm's production plan is (x, y, z) = (102, 19, 957), and the market prices are (px , py , pz ) = (10, 5, 1.25). How much profit would the firm make if it carried out this plan?

Problem 5. Suppose that a firm's production function is f(x,y) = 20x0.7y0.3. Starting from the input bundle (x, y) = (40, 60), how much extra output will the firm get if it increases x from 40 to 41? How many units of output will the firm lose if x decreases from 40 to 39?

Problem 6. Suppose that the production of airframes (for aircraft) uses two inputs: capital (good x) and labor (good y). The production function is f(x,y) = xy. Assume that the price of capital is $1 per unit, and the price of labor is $10 per unit. The manufacturer wants to make 121,000 airframes. Find the cost-minimizing combination of capital and labor inputs.

ii.

Work out the following questions with explanations.

1 Total Planned Expenditures C H Real National Income 7) In the above figure. at an income level of Yj and planned expenditure of (C : Thy. the level of autonomous Investment is AJED B) JK D) FF 8) At an income level of Yj and planned expenditures of (C + Dj in the above figure, Aj the quantity of aggregate demand eureeds real Grom Domestic Product (GDP). B) there is full employment C planned saving exceeds planned investment Dj the economy is in equilibrium. 9) How is economic growth graphically depicted? A) The aggregate demand curve shifts to the left B) The long-run aggregate supply curve shifts right C) Aggregate demand shifts to the right. Dj Short-run aggregate supply shifts left 10) Given the Not of assets below, which is the most liquid? A) A one-ounce gold coin By A 5500 traveler's check C) $500 worth of General Motors common stock D) $500 worth of General Motors bonds 11) If, at some level of output, total planned expenditures are less than real Gross Domestic Product (GDI) AJ unplanned inventories will increase and mal GDP will fall Bj real GDP remains unchanged. () real GDP will either fall or remain unchanged, depending on the MPC. DJ real GDP will rise. 12) Which of the following tools are used as part of a country's fiscal policy? A) Changing tax policy B) Changing the money supply Q Changing exchange rates Dj Changing interest rates 3) The interest-rate-based monetary policy transmission mechanism argues that an increase in the money supply A] causes interest rates to fall, which causes an increase in planned investment, and an increase in aggregate demand B) has no effect on aggregate demand but reduces long-run aggregate supply. () causes the inflation rate to decline, which causes an increase in household consumption spending and an Increase in aggregate demand. D) has no effect on aggregate demand but increases short-run aggregate supply.(15 points) Problem 2 In the short-run, we assume that capital is a fixed input and labor is a variable input, so the firm can increase output only by increasing the amount of labor it uses. In the short run, the firm's production function is q = f (L, K) = 8LK + 512 - 2 13, where q is output, L is labor, and K = 5 is the fixed units of capital. (a) What is the marginal product of labor as a function of L? (b) What is the average product of labor as a function of L? (c) At which point does the law of diminishing returns set in? (Find the critical value L*.)(30 points) Problem 4 Consider a consumer with preferences represented by the utility function U (x, y) = 2x'4y0'5. She has a wealth of w and faces the market prices of x and y, Px and P3,, respectively. (a) Derive the consumer's Marshallian (uncompensated) demand functions. (b) What is the consumer's Engel curve for good y? (Hint: This can be obtained directly from the Marshallian demand function for good y.) Is y a normal good or an inferior good? (c) Derive the consumer's expenditure function, E (Px, Py, [7). ((1) Now suppose Px = $2, 1\40. Which of the following types of production technology always results in constant marginal cost? Circle all that apply. A. Cobb-Douglas with increasing returns to scale B. Cobb-Douglas with decreasing returns to scale C. Cobb-Douglas with constant returns to scale D. Perfect complements E. Perfect substitutes F. Quasi-linear 41. Suppose that a firm's total cost function is TC(q): = 6q- + 72q + 404 and its marginal cost is MC(q) = 12q + 72. Find this firm's efficient scale and its minimized ATC. 42. Suppose that a firm's production function is f (x, y) = 3x + 7y, and input x is fixed in the short-run at x = 200 units. Assume the input prices are px = 12, py = 5. Write down a formula for the firm's short-run cost function C(q). Then, calculate the firm's long-run cost function and compare the two