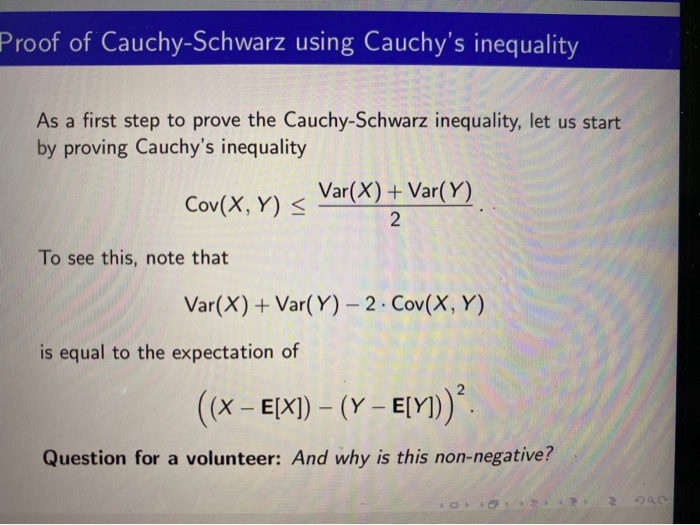
Proof of Cauchy-Schwarz using Cauchy's inequality As a first step to prove the Cauchy-Schwarz inequality, let us start by proving Cauchy's inequality Cov(X, Y) To see this, note that Var(X) + Var(Y) 2 Var(X) + Var(Y)-2. Cov(X, Y) is equal to the expectation of ((X - E[X]) - (Y - E[Y])). Question for a volunteer: And why is this non-negative?