Prove equation  Ef (Fermi energy).

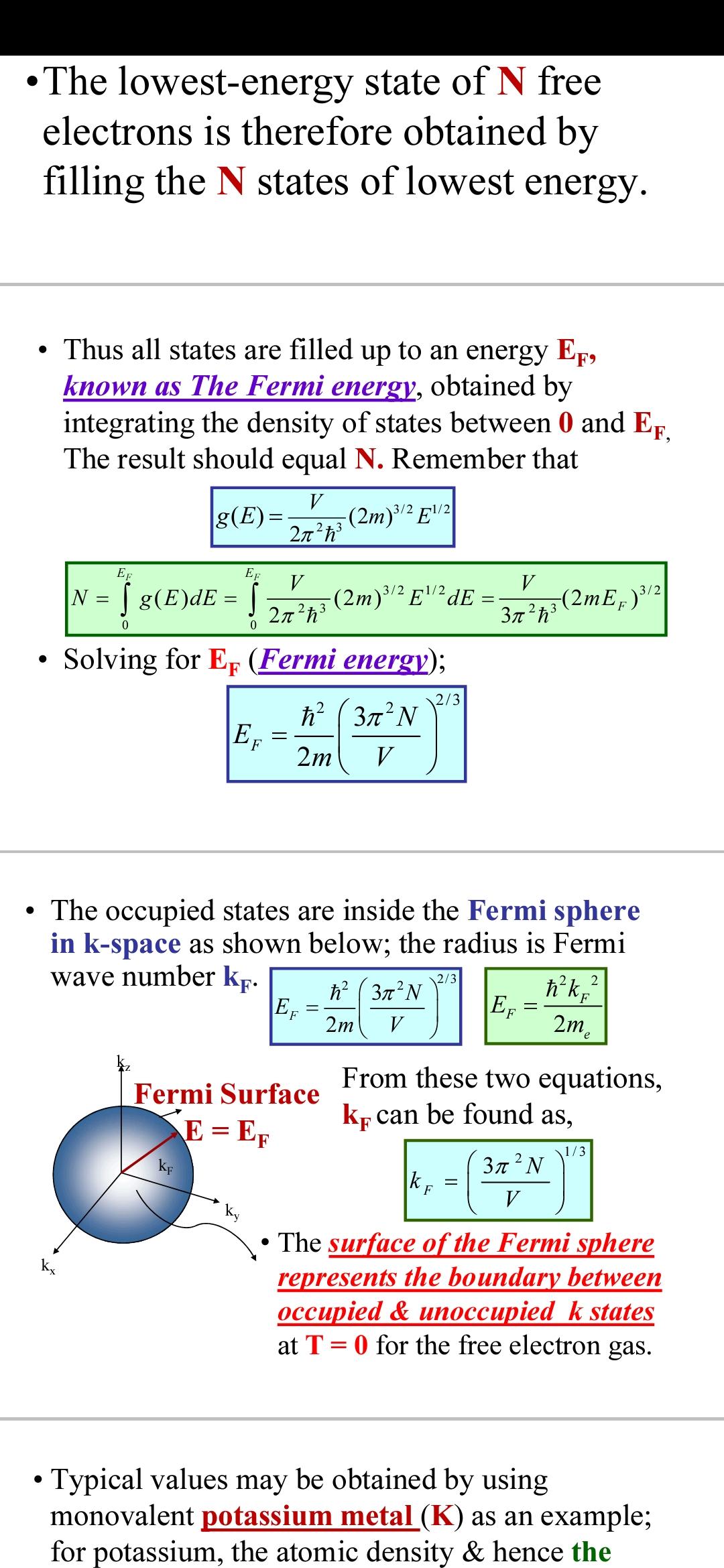
The lowest-energy state of N free electrons is therefore obtained by filling the N states of lowest energy. Thus all states are filled up to an energy EF, known as The Fermi energy, obtained by integrating the density of states between 0 and E, The result should equal N. Remember that V -(2m)/ E"2 272h 3/2 g(E)= EF EF N = [ g(E)dE = [ V (2m) 3/2 E/2 dE = V (2mE,)1 3/2 3 2n h Solving for Er (Fermi energy); 2/3 h? ( 37N E = 2m V The occupied states are inside the Fermi sphere in k-space as shown below; the radius is Fermi wave number kr. 2/3 h ( 3n N EF F EF 2m 2m. From these two equations, Fermi Surface E = EF kF can be found as, 1/3 KF k F V The surface of the Fermi sphere represents the boundary between occupied & unoccupied k states at T = 0 for the free electron gas. Typical values may be obtained by using monovalent potassium metal (K) as an example; for potassium, the atomic density & hence the